A food package was dropped from an aircraft flying horizontally. 6 s before it hit the ground, it was at a height of 780m, and had travelled a distance of 1 km horizontally. Find the speed and the altitude of the aircraft.
The correct Answer is:360km/hr,1.28km
To solve the problem, we need to find the speed of the aircraft and the altitude from which the food package was dropped. We know that the package was dropped from a height of 780 m and it traveled 1 km horizontally in 6 seconds before hitting the ground.
Step 1: Find the speed of the aircraft
1. Given Data:
- Horizontal distance traveled by the package (s) = 1 km = 1000 m
- Time taken (t) = 6 s
2. Using the formula for horizontal motion:
s=vt
Here, v is the speed of the aircraft.
3. Rearranging the formula to find v:
v=st=1000 m6 s=10006 m/s≈166.67 m/s
Step 2: Find the altitude of the aircraft
1. Given Data:
- Height of the package at the moment of dropping (h) = 780 m
- Time taken to hit the ground = 6 s
2. Using the vertical motion equations:
The vertical displacement can be calculated using the equation:
s=ut+12at2
where:
- s = vertical displacement = -780 m (downward)
- u = initial vertical velocity = 0 (since it is dropped)
- a = acceleration due to gravity = -10 m/s² (downward)
- t = time = 6 s
3. Substituting the values into the equation:
−780=0⋅6+12⋅(−10)⋅(62)
−780=−5⋅36
−780=−180
This confirms the vertical displacement calculation.
4. Finding the total height from which the package was dropped:
The total height (H) of the aircraft can be calculated as:
H=h+displacement
H=780+780=1560 m
Final Answers:
- Speed of the aircraft: 166.67 m/s
- Altitude of the aircraft: 1560 m
- Horizontal distance traveled by the package (s) = 1 km = 1000 m
- Time taken (t) = 6 s
s=vt
Here, v is the speed of the aircraft.
v=st=1000 m6 s=10006 m/s≈166.67 m/s
- Height of the package at the moment of dropping (h) = 780 m
- Time taken to hit the ground = 6 s
The vertical displacement can be calculated using the equation:
s=ut+12at2
where:
- s = vertical displacement = -780 m (downward)
- u = initial vertical velocity = 0 (since it is dropped)
- a = acceleration due to gravity = -10 m/s² (downward)
- t = time = 6 s
−780=0⋅6+12⋅(−10)⋅(62)
−780=−5⋅36
−780=−180
This confirms the vertical displacement calculation.
The total height (H) of the aircraft can be calculated as:
H=h+displacement
H=780+780=1560 m
- Speed of the aircraft: 166.67 m/s
- Altitude of the aircraft: 1560 m
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-04[B]
14 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#7
3 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A bomb is dropped from an aircraft travelling horizontally at 150ms−1 at a height of 490m. The horizontal distance travelled by the bomb before it hits the ground is
View SolutionAn aeroplane flying horizontally 1 km above the ground is observed at an elevation of 60o . After 10 seconds, its elevation is observed to be 30o . Find the speed of the aeroplane in km/hr.
View Solution
Knowledge Check
A bomb is dropped from an aeroplane flying horizontally with a velocity 720 km/hr at an altitude of980 m. The bomb will hit the ground after a time
A1 secB7.2 secC14.15 secD0.15 sec
A relief food package is dropped from a airplane which is moving horizontal with a velocity of 30ms−1 at a height h=50m. Find the (a) time of flight of the package, (b) location of the point of striking of the food package, (c ) velocity of the package at the time of striking the ground, and (d) displacement of the food package.
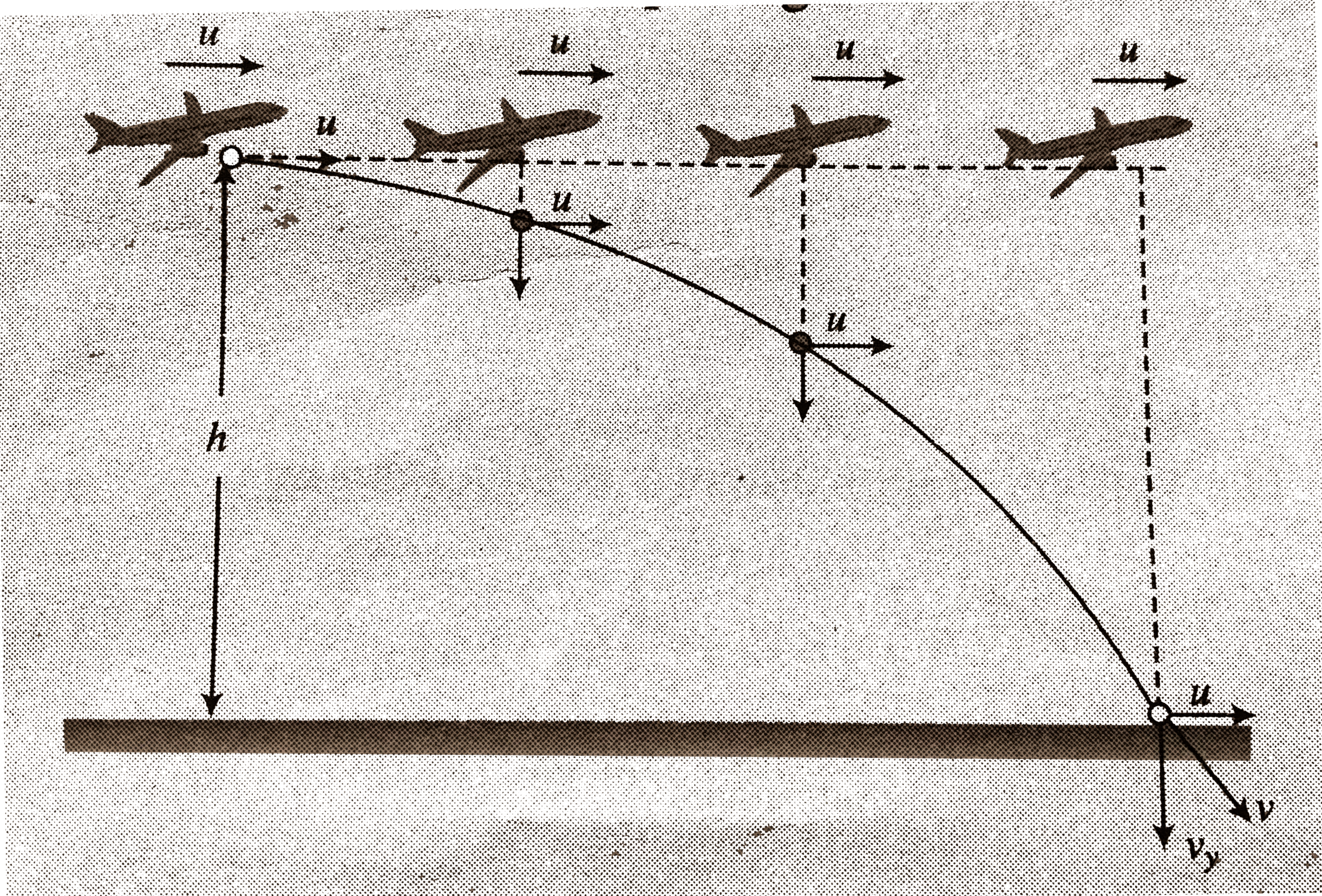 .
.
View SolutionA food packed is dropped, from a height of 500 m, from a plane flying horizontally with speed 100km/h. The packet reaches to the ground after travelling a horizontal distance. [ Take g =10ms−2]
View SolutionA ball is dropped from a height. If it takes 0.200 s to cross thelast 6.00 m before hitting the ground, find the height from which it was dropped. Takeg=10ms2.
View SolutionA plane flying horizontally at 100ms−1 releases an object which reaches the ground in 10s. At what angle with horizontal it hits the ground ?
View SolutionA projectile projected with initial velocity 20m/s and at and angle of 30∘ from horizontal. Find the total work done when it will hit the ground.
View SolutionAn aeroplane flying horizontally at an altitude of 490m with a speed of 180 kmph drops a bomb. The horizontal distance at which it hits the ground is
View SolutionA bomb is dropped from an aeroplane flying horizontally with a velocity 469ms−1 at an altitude of 980 m . The bomb will hit the ground after a time ( use g=9.8ms−2)
View Solution
