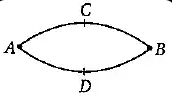
A length of path ACB is 1500 m and the length of the path ADB is 2100 m. Two particles start from point A simultaneously around the track ACBDA. One of them travels the track in clockwise sense and other in anti-clockwise sense and other in anti-clockwise with their respective constant speeds. After 12 s from the start, the first time they meet at the point B. After minimum time (in s) in which they meet first at point B, again will they meet at the point B is time tmin=(12)xs.The value of x is
2
3
4
5
The correct Answer is:(a)
The velocity of first particle is v1=150012ms−1 and the velocity of second particle is v2=210012ms−1. Let after completing n1 and n2 trips, they will again meet at the point B.
∴ 3600n1v1=3600n2v2 = t
[total path length =1500+2100=3600]
or n1n2=v1v2=150012210012=15002100=57
∴tmin=3600×5v1
3600×51500×12=144s=(12)x
∴ x = 2
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - NCERT Exemplar
6 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Assertion And Reason
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Relative Velocity
18 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
- OSCILLATIONS BOOK - NCERT FINGERTIPSCHAPTER - OSCILLATIONS EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A circle of radius unity is centered at thet origin. Two particles tart moving at the same time from the point (1,0) and move around the circle in opposite direction. One of the particle moves anticlockwise with constant speed v and the other moves clockwise with constant speed 3v. After leaving (1,0), the two particles meet first at a point P, and continue until they meet next at point Q. The coordinates of the point Q are
View SolutionA circle of radius unity is centered at thet origin. Two particles tart moving at the same time from the point (1,0) and move around the circle in opposite direction. One of the particle moves anticlockwise with constant speed v and the other moves clockwise with constant speed 3v. After leaving (1,0), the two particles meet first at a point P, and continue until they meet next at point Q. The coordinates of the point Q are
View Solution
Knowledge Check
A man is facing towards the east. He then turns anti-clockwise, again anti-clockwise and then clock wise. In which direction is he facing now?
AWestBNorthCSouthDEastTwo particles A and B execute SHM on a straight line of path length 2 m starting from the two extreme points simultaneously. If their respective time periods are 1 s and 2 s, the minimum time in which they meet is
A14sB13sC12sD25s
A man is facing towards the east. He then turns 45° anti-clockwise, again 180° anti-clockwise and then 225° clockwise. In which direction is he facing now?
View SolutionAn athlete starts running along a circular track of 50 m radius at a speed 5m/s in the clockwise direction for 40 s. Then the athlete reverses direction and runs inthe anticlockwise direction at 3 m/s for 100 s. At the end, how far around the track is the runner from the starting point ?
View SolutionHow many right angles do you make if you start facing
north and turn anti-clockwise to east ?
View SolutionThree particle A, B and C situated at the vertices of an equilateral triangle starts moving simultaneously at a constant speed "v" in the direction of adjacent particle, which falls ahead in the anti-clockwise direction. If "a" be the side of the triangle, then find the time when they meet
View SolutionThe distance between two stations is 340 km. Two trains start simultaneously from these stations on parallel tracks to cross each other. The speed of one of them is greater than that of the other by 5 km/hr. If the distance between the two trains after 2 hours of their start is 30 km, find the speed of each train.
View SolutionThe distance between two stations is 340 km. Two trains start simultaneously from these stations on parallel tracks to cross each other. The speed of one of them is greater than that of the other by 5 km/hr. If the distance between the two trains after 2 hours of their start is 30 km, find the speed of each train.
View Solution
