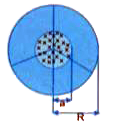
A line charge per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.08). A uniform magnetic field extends over a circular region within the rim. It is given by,
= 0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off ?
If the magnetic field changes with time, then,
Induced emf
This implies the presence of an electric field tangential to all points of the periphery of the circular region denoted by a. If we move a test charge round this periphery a work qe will be done once round it. The electric force on the test charge is q E and the work done by this force round this periphery is . Equating the two values of work done, we have
Substituting the value of , we get
In the given problem, the total charge on the rim
Therefore, force on this charge .
According to Newton.s second law of motion, force F = M(acceleration)
On integrating, we get vec omega=-(Bpia^(2)lambda)/(MR)hatk
Topper's Solved these Questions
- ELECTROMAGNETICE INDUCTIONBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETICE INDUCTIONEXERCISE - Case Based/Source-Based Integrated Questions
3 Videos
- ELECTROMAGNETICE INDUCTIONBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETICE INDUCTIONEXERCISE - Multiple Choice Questions
30 Videos
- ELECTROMAGNETICE INDUCTIONBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETICE INDUCTIONEXERCISE - Self Assessment Test Section -D
1 Videos
- ELECTROMAGNETIC WAVESBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - SELF ASSESSMENT TEST (SECTION C)
1 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - U-LIKE SERIESCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SELF ASSESSMENT TEST
1 Videos
Similar Questions
A line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.22). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=B0k (ra≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

View SolutionA line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig.). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=−B0k (r≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

View Solution
Knowledge Check
A line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
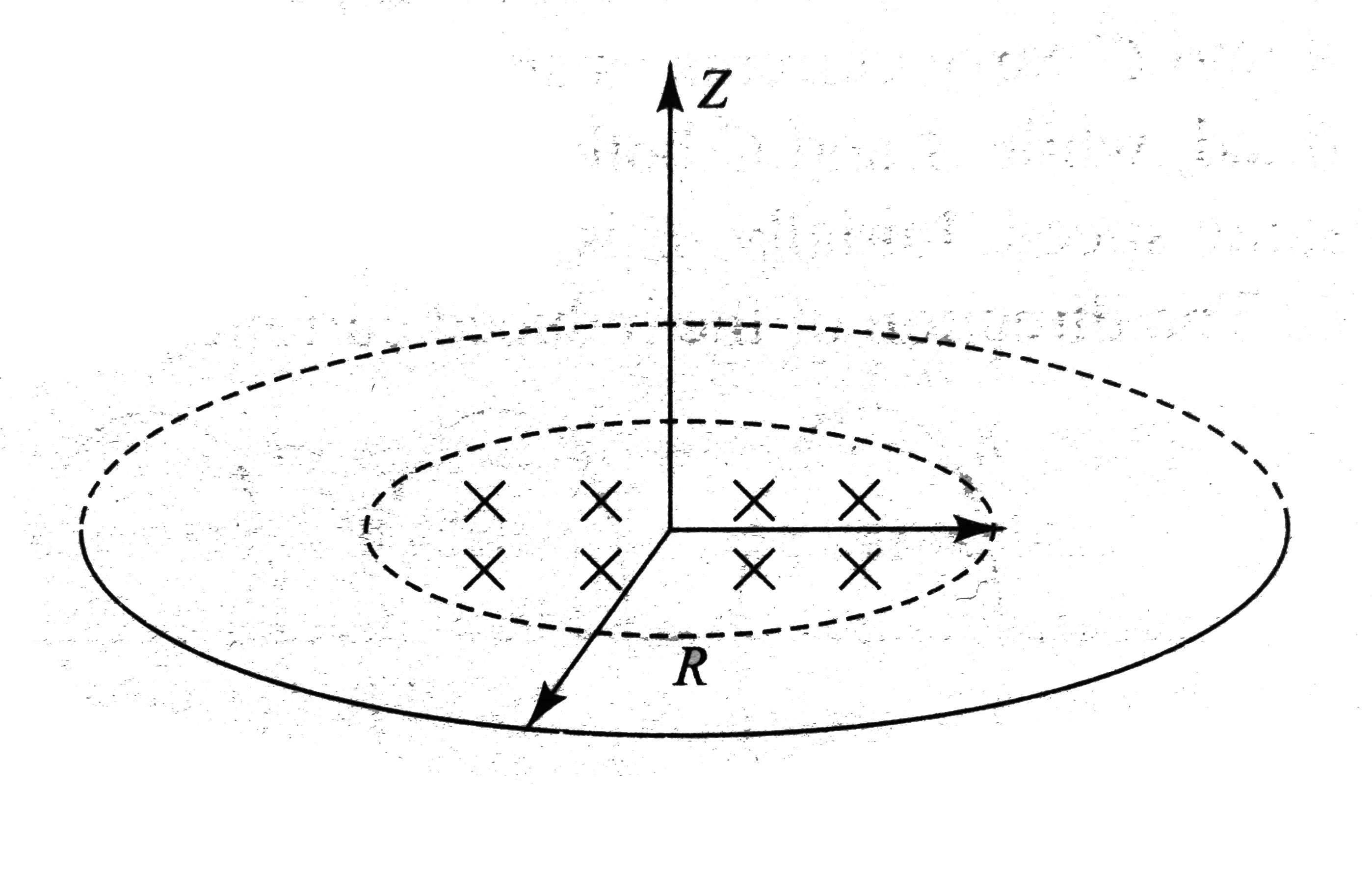 A(a) −2B0πa2rmRˆkB(b) −2B0πa2r3mRˆkC( c) B0πa2λmRˆkD( d) −B0πa2λmRˆk
A(a) −2B0πa2rmRˆkB(b) −2B0πa2r3mRˆkC( c) B0πa2λmRˆkD( d) −B0πa2λmRˆkA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is
 A(M+m4)R2B(M+m)R2C(M+3m)R2D(M+m2)R2
A(M+m4)R2B(M+m)R2C(M+3m)R2D(M+m2)R2A circular wheel is rotating in horizontal plane without friction about its axis. If a body is gently attached to the rim of the wheel then following is false.
AMoment of inertia increases but angular momentum remains sameBangular velocity decreases but angular momentum remains sameCRotational kinetic energy decreases but angular momentum remains sameDAngular momentum increases but angular velocity remins same
A line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
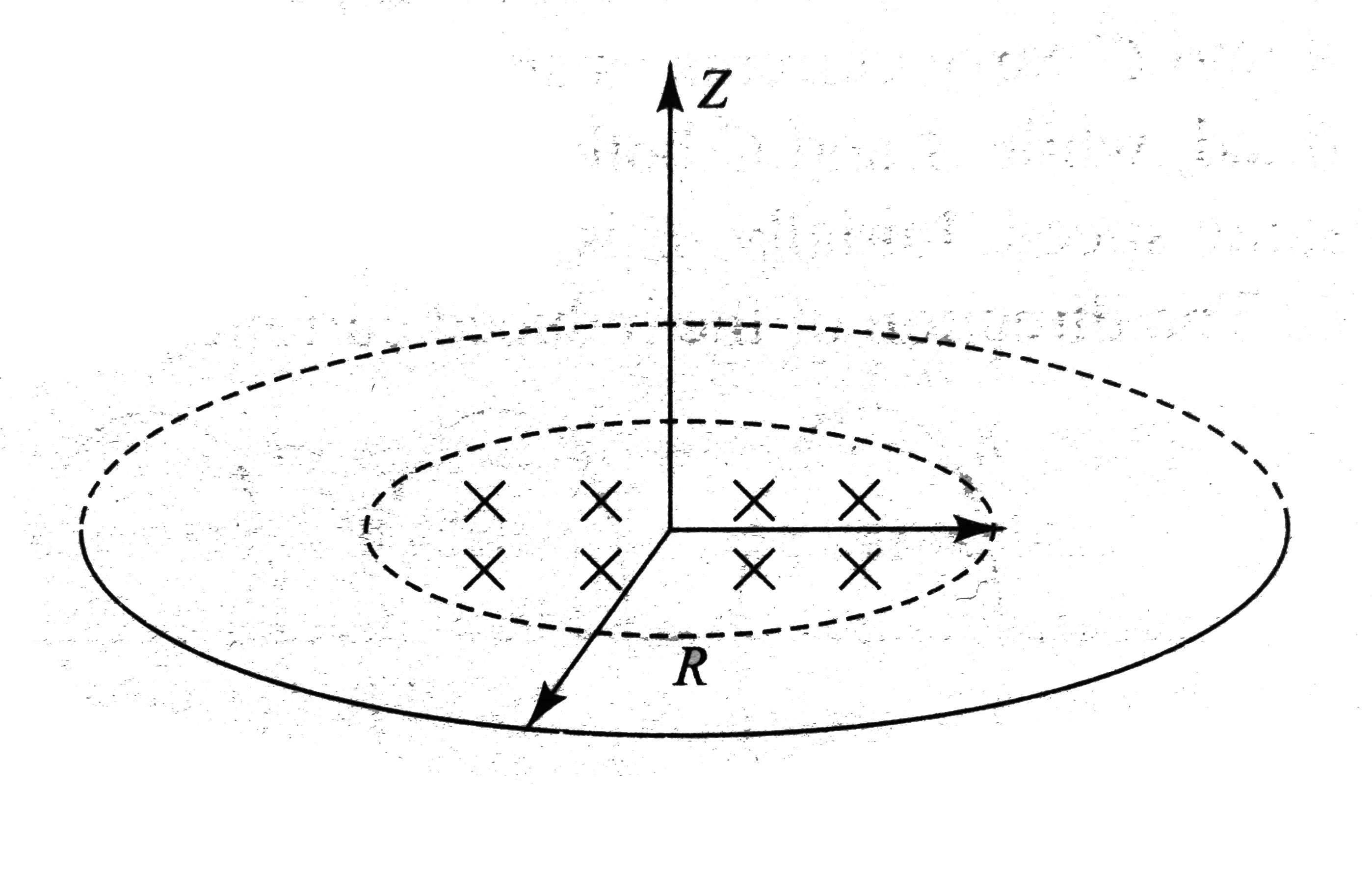
View SolutionA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is

View SolutionThe spokes of a wheel are made of metal and their lengths are of one metre. On rotating the wheel about its own axis in a uniform magnetic field of 5×10−5 tesla normal to the plane of the wheel, a potential difference of 3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is-
View SolutionA string is wrapped around the rim of a wheel of moment of inertia 0.20 kg-m^2 and radius 20 cm. The wheel is free to rotate about it axis. Initially, the wheel is at rest. The string is now pulled by a force of 20 N. Find the angular velocity of the wheel after 5.0 seconds.
View SolutionThe spokes of a wheel are made of metal and their lengths are of one metre. On rotating the wheel about its own axis in a uniform magnetic field of 5×10−5 tesla normal to the plane of the wheel, a potential difference of 3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is-
View Solution
