A mercury drop shaped as a round tablet of radius R and and thickness h is located between two horizontal glass plates. Assuming that h<<R, find the mass m of a weight which has to be placed on the upper plate to diminish the distance between the plates n-times. The contact angle equals θ. Calculate m if R=2.0cm,h=0.38mm,n=2.0, and θ=135∘.
We must first calculate the pressure difference inside the film from that outside. This is
p=α(1r1+1r2)
Here 2r1|cosθ|=h and r2~−R the radius of the tablet and can be neglected. Thus the total force exerted by mercury drop on the upper glass plate is
2πR2α|cosθ|h typically
We should put h/n for h because the tablet is compresed n times. Then since Hg is nearly,
incompressible, πR2h=constant soR→R√n. Thus,
total force = 2πR2α|cosθ|hn2
Part of the force is needed to keep the Hg in the shape of a table rather than in the shape of infinitely thin sheet. This part can be calculated being putting n=1 above. Thus
mg+2πR2α|cosθ|h=2πR2α|cosθ|hn2
or m=2πR2α|cosθ|hg(n2−1)=0.7kg.
 .
.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Phase Transformations
35 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - The Second Law Of Thermodynamics - Entropy
47 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
Two parallel plates which are sparated by a very small distance d, are dipped in water. To how much height will the water raise between the plates (Assume contact angle θ→0)
View SolutionThe' distance between the plates of a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates. What will e its effect on the capacitance.
View Solution
Knowledge Check
A mercury drop shape as a round tablet of radius R and thickness h is located between two horizontal glass plates. Assuming that h<<R, find the mass m of a weight which has to be placed on the copper plate to diminish the distance between the plates by n-times the contact angle is equal to θ. calculate m if T is surface tension of the liquid.
Am=2πRT2|cosθ|gh(n2−1)Bm=2πR2T|sinθ|gh(n2−1)Cm=2πR2T|cosθ|gh(n2+1)Dm=2πR2T|cosθ|gh(n2−1)If a water drop is kept between two glass plates, then its shape is
A_E01_027_O01.png) B
B_E01_027_O02.png) C
C_E01_027_O03.png) D
D_E01_027_O04.png)
A drop of water of mass m and density ρ is placed between two weill cleaned glass plates, the distance between which is d. What is the force of attraction between the plates?
(T= surface tension)ATm2ρd2B4Tmρd2C2Tmρd2DTmρd2
A drop of water of 0.2 g mass is placed between two well cleaned glass plates, the distance between which is 0.01 cm. Find the force of attraction between the plates,
View SolutionThe distance between the plates fo a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates, what will be the new capacity ?
View SolutionA metal slab of thickness, equal to half the distance between the plates is introduced between the plates of a parallel plate capacitor as shown. Find its capacity.
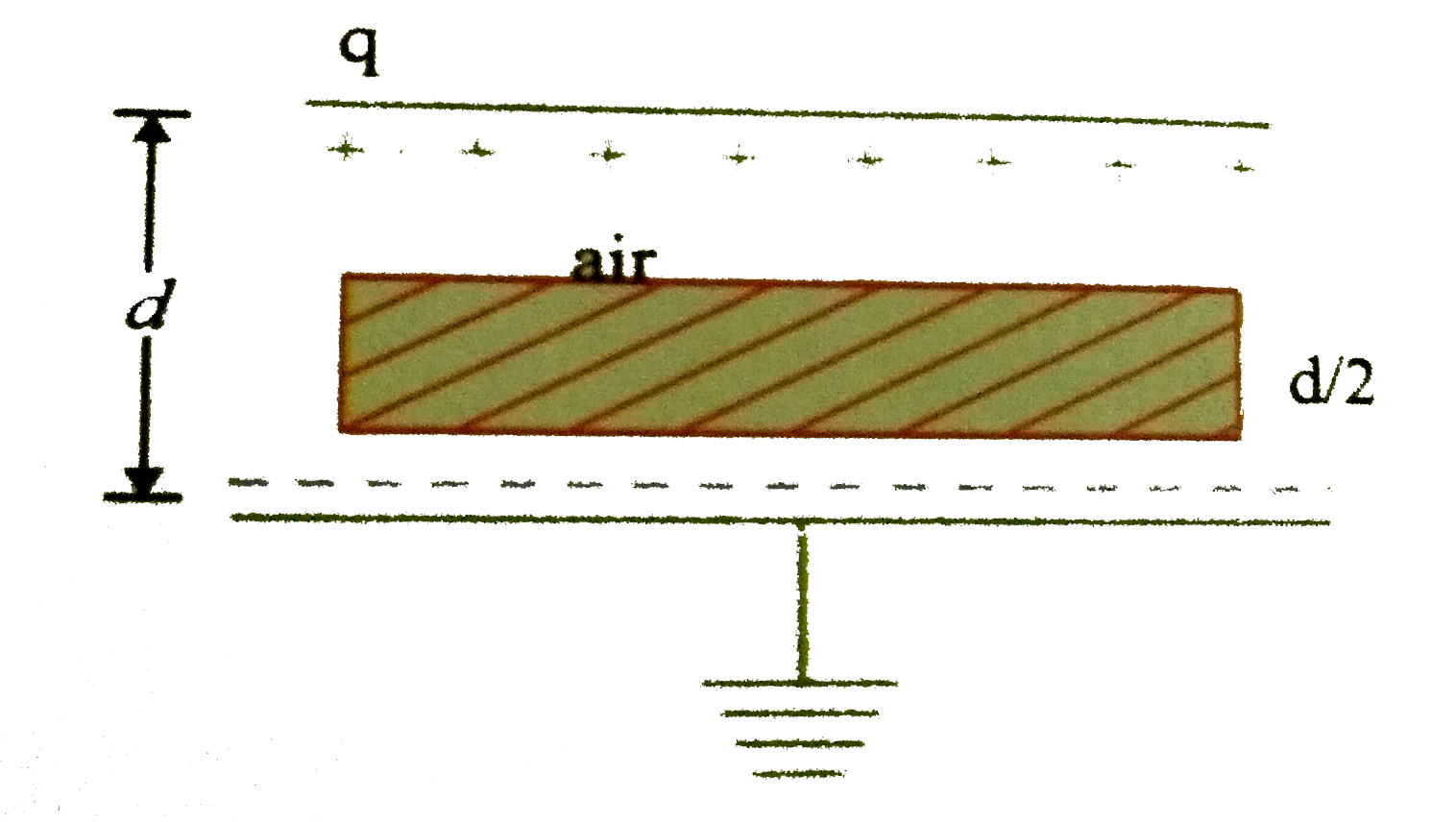 .
.
View SolutionThe distance between the plates fo a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates, what will be the new capacity ?
View SolutionThe' distance between the plates of a parallel plate capacitor is d. A metal plate of thickness d/2 is placed between the plates. What will e its effect on the capacitance.
View Solution
