A metal wire of length L1 and area of cross section A is attached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
[(Y1Y2)A][2(Y1L2+Y2L1)]
[(Y1Y2)A](L1L2)2
[(Y1Y2)A](Y1L2+Y2L1)
[(Y1Y2)1/2A](L2+L1)1/2
The correct Answer is:C
To find the effective force constant of the system of two metal wires, we will follow these steps:
Step 1: Understand the System
We have two metal wires attached in series. The first wire has length L1 and Young's modulus Y1, and the second wire has length L2 and Young's modulus Y2. Both wires have the same cross-sectional area A.
Step 2: Use Young's Modulus to Relate Force and Elongation
Young's modulus Y is defined as the ratio of stress to strain:
Y=StressStrain=F/AΔL/L
From this, we can derive the relationship for force F and elongation ΔL:
Y=FLAΔL⟹F=YAΔLL
Step 3: Define the Force Constants for Each Wire
The force constant k for a wire can be defined as:
k=FΔL
From the previous equation, we can express k in terms of Young's modulus:
k=YAL
Thus, for the first wire:
k1=Y1AL1
And for the second wire:
k2=Y2AL2
Step 4: Calculate the Effective Force Constant
When two springs (or wires in this case) are connected in series, the effective force constant kequiv is given by:
1kequiv=1k1+1k2
Substituting the expressions for k1 and k2:
1kequiv=L1Y1A+L2Y2A
This can be simplified to:
1kequiv=L1Y2+L2Y1Y1Y2A
Step 5: Invert to Find kequiv
Taking the reciprocal gives us:
kequiv=Y1Y2AL1Y2+L2Y1
Final Result
Thus, the effective force constant of the system of two wires is:
kequiv=Y1Y2AL1Y2+L2Y1
We have two metal wires attached in series. The first wire has length L1 and Young's modulus Y1, and the second wire has length L2 and Young's modulus Y2. Both wires have the same cross-sectional area A.
Young's modulus Y is defined as the ratio of stress to strain:
Y=StressStrain=F/AΔL/L
From this, we can derive the relationship for force F and elongation ΔL:
Y=FLAΔL⟹F=YAΔLL
The force constant k for a wire can be defined as:
k=FΔL
From the previous equation, we can express k in terms of Young's modulus:
k=YAL
Thus, for the first wire:
k1=Y1AL1
And for the second wire:
k2=Y2AL2
When two springs (or wires in this case) are connected in series, the effective force constant kequiv is given by:
1kequiv=1k1+1k2
Substituting the expressions for k1 and k2:
1kequiv=L1Y1A+L2Y2A
This can be simplified to:
1kequiv=L1Y2+L2Y1Y1Y2A
Taking the reciprocal gives us:
kequiv=Y1Y2AL1Y2+L2Y1
Thus, the effective force constant of the system of two wires is:
kequiv=Y1Y2AL1Y2+L2Y1
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - APPLICATIONS OF ELASTIC BEHAVIOUR OF MATERIALS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOTS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - STRESS-STRAIN CURVE
5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A wire of length L,area of cross section A is hanging from a fixed support. The length of the wire changes to L1 when mass M is suspended from its free end. The expression for Young's modulus is:
View SolutionOne end of a Nichrome wire of length 2L and cross-sectional area A is attached to an end of another Nichrome wire of length L and cross-sectional area 2A. If the free end of the longer wire is at an electric potential of 8.0 volts, and the free end of the shorter wire is at an electric potential 1.0 volts, the potential at the junction of the two wires is equal to
View Solution
Knowledge Check
A wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the force constant of the wire is
AYLABYlACYALDYAlA wire of length L and cross-sectional area A is made of a material of Young's modulus Y. IF the wire is stretched by an amount x, the workdone is
AYAx22LBYAx2L2CYAx2LDYAx2L
A body of mass M is attached to the lower end of a metal wire, whose upper end is fixed . The elongation of the wire is l.
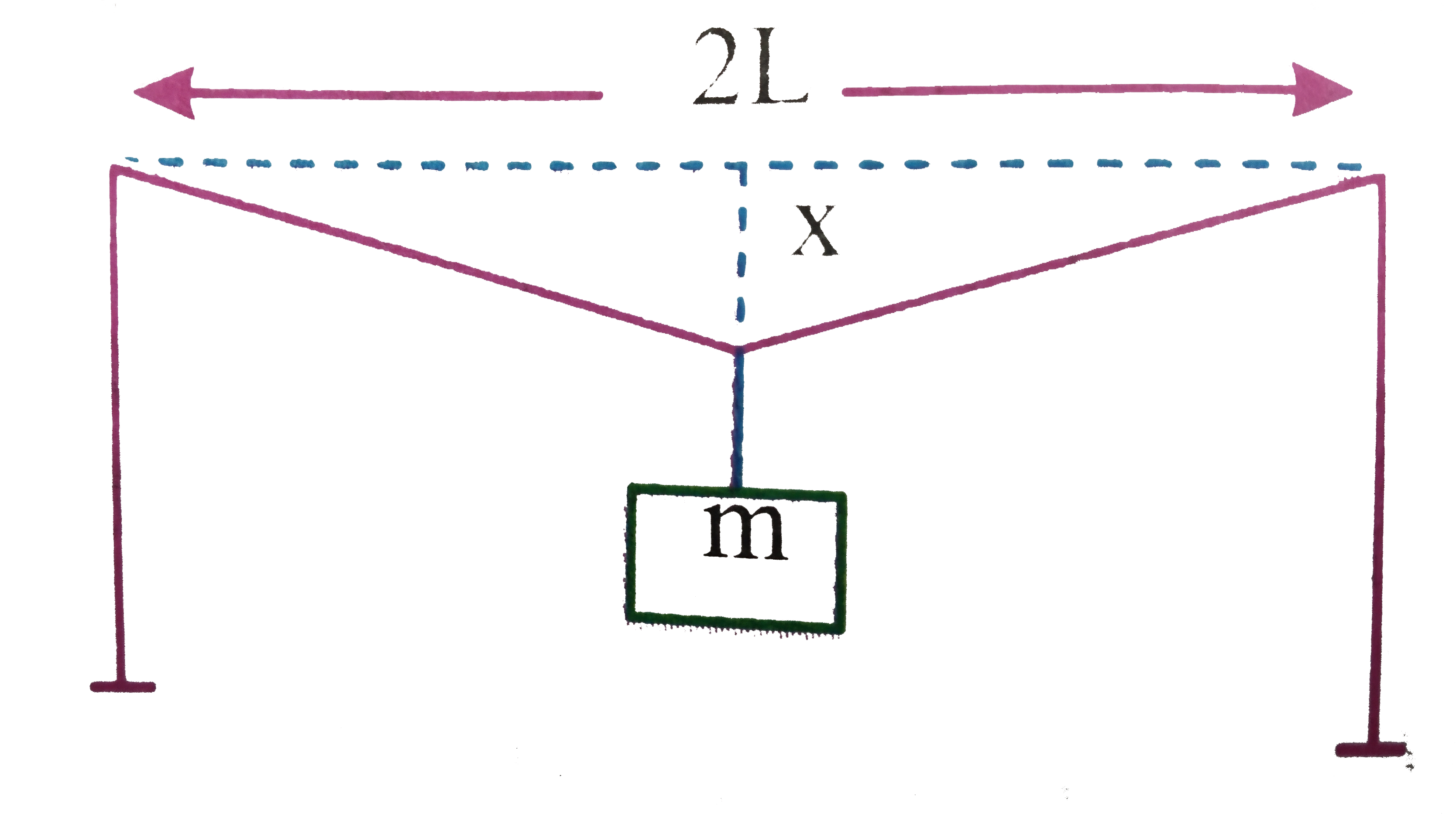
View SolutionA mild steel wire of length 1.0m and cross sectional area 2L is strethched, within its elastic limit horizontally between two pillars(figure). A mass of m is suspended form the midpont of the wire. Strain in the wire is
View SolutionA block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View SolutionThe V – I graph for a wire of copper of length L and cross -section area A is shown in the figure below . The slope of the graph will be
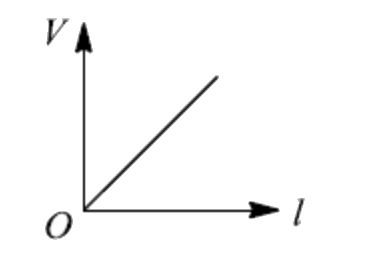
View SolutionThe V – I graph for a wire of copper of length L and cross -section area A is shown in the figure below . The slope of the graph will be

View SolutionA force F doubles the length of wire of cross-section a The Young modulus of wire is
View Solution
