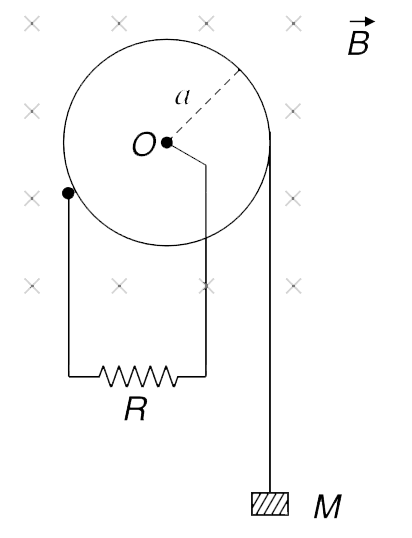
A metallic pulley is in the shape of a disc of radius a. It can rotate freely about a horizontal axis passing through its centre. The moment of inertia of the pulley about this axis is I. A light string is tightly wrapped around the pulley with its one end connected to a block of mass M. The centre of the pulley and its circumference are connected to a resistance R as shown. The contact of resistance at the circumference does not cause any friction. A uniform magnetic field B is switched on which is parallel to the axis of rotation of the pulley (see Figure). The mass M is allowed to fall. Assume that resistivity of the material of the pulley is negligible.
(a) Find the acceleration of the block of mass M at the instant its velocity becomes $v_{0}$ .
(b) Assuming that the block can fall through a large distance, calculate the terminal speed $(v_{T})$ that it will acquire.
(c) Find the rate of change of kinetic energy of the pulley at the instant speed of the falling block is $\frac{v_{T}}{2}$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(a) $\frac{M g a^{2} - \frac{B^{2} a^{4} v_{0}}{4 R^{2}}}{I + M a^{2}}$
(b) $\frac{4 M g R^{2}}{B^{2} a^{2}}$
(c) $\frac{I M^{2} g^{2} R^{2}}{B^{2} a^{2} (I + M a^{2})}$

Answer

Step by step video, text & image solution for A metallic pulley is in the shape of a disc of radius a. It can rotate freely about a horizontal axis passing through its centre. The moment of inertia of the pulley about this axis is I. A light string is tightly wrapped around the pulley with its one end connected to a block of mass M. The centre of the pulley and its circumference are connected to a resistance R as shown. The contact of resistance at the circumference does not cause any friction. A uniform magnetic field B is switched on which is parallel to the axis of rotation of the pulley (see Figure). The mass M is allowed to fall. Assume that resistivity of the material of the pulley is negligible. (a) Find the acceleration of the block of mass M at the instant its velocity becomes v_(0). (b) Assuming that the block can fall through a large distance, calculate the terminal speed (v_(T)) that it will acquire. (c) Find the rate of change of kinetic energy of the pulley at the instant speed of the falling block is (v_(T))/(2). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
Moment of inertia of a rigid body about an axis passing through its centre of mass is $I_{0}$ and moment of inertia of the same body about another axis parallel to the first axis is I. Then
AI is always equal to $I_{0}$
BI is always smaller than $I_{0}$
CI is always greater than $I_{0}$
Dany of the above three
Question 2 - Select One
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
Aincreases if its temperature is increased
Bchanges if its axis of rotation is changed
Cincreases if its angular velocity is increased
Dboth (a) and (b) are correct
Question 3 - Select One
The M.I. of disc of mass M and radius 'R' about an axis passing through midway between centre and circumference and perpendicular to its plane is
A $M R^{2} / 2$
B $5 / 4 M R^{2}$
C $M R^{2}$
D $3 / 4 M R^{2}$

ARIHANT-ELECTROMAGNETIC INDUCTION-Level 3

Home
Ask Doubt
Profile

The correct Answer is:(a) Mga2−B2a4v04R2I+Ma2 (b) 4MgR2B2a2 (c) IM2g2R2B2a2(I+Ma2)

Topper's Solved these Questions

Similar Questions

Knowledge Check

Moment of inertia of a rigid body about an axis passing through its centre of mass is I0 and moment of inertia of the same body about another axis parallel to the first axis is I. Then

The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane

The M.I. of disc of mass M and radius 'R' about an axis passing through midway between centre and circumference and perpendicular to its plane is

ARIHANT-ELECTROMAGNETIC INDUCTION-Level 3

The correct Answer is:(a) $\frac{M g a^{2} - \frac{B^{2} a^{4} v_{0}}{4 R^{2}}}{I + M a^{2}}$
(b) $\frac{4 M g R^{2}}{B^{2} a^{2}}$
(c) $\frac{I M^{2} g^{2} R^{2}}{B^{2} a^{2} (I + M a^{2})}$

Moment of inertia of a rigid body about an axis passing through its centre of mass is $I_{0}$ and moment of inertia of the same body about another axis parallel to the first axis is I. Then