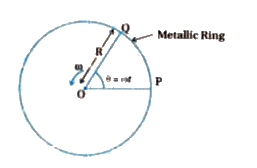
A metallic rod of 1 m length is rotated with a frequency of 50 rev/s, with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular to the plane of the ring as per figure. A constant and uniform magnetic field of 1T parallel to the axis is present everywhere. What is the emf between the centre and the metallic ring ?
 Solution in Gujrati
Solution in Gujrati

Consider uniform magnetic field →B(x), perpendicularly inside the plane of figure. Also consider a metallic ring of radius R whose plane is perpendicular to →B. There is a conducting rod (OQ) of length R, with its one end hinged at O (centre of ring) and another end in contact with the ring. Suppose, this rod rotates anticlockwise with constant angular speed ω . Because of this motion of rod, free electrons in it acquire velocity →v⊥→B and hence they are exerted upon by magnetic force →Fm=e(→B×→v) in a direction from centre to outer end. Hence, free electrons in the rod start displacing away from centre, making centre positive and outer end negative. After some time when electric force →Fe exerted on free electrons becomes equal and opposite to magnetic force →Fm, a steady state is reached when Lorentz force exerted on electrons becomes zero. At this stage, we get maximum induced emf across the two ends of rod (or between centre and periphery of ring). Let this emf be ε whose formula can be derived as follows.
Across the elemental length dr of rod chosen at distance r from centre if induced emf is dε then according to formula of motional emf we can write,
dε=Bvdr
∴dε=B(rω)dr
∴ Total induced emf across the rod will be ,
ε=∫dε
=∫B(rω)dr
=Bω∫R0rdr
∴ε=Bω{r22}R0
∴ε=12BωR2
Second Method : In above figure, suppose the rod rotates by extremely small angle dθ in time dt. Here, length of arc QQ.=Rdθ. Now, area traversed (or swept) by rod in time dt would be
dA=12 (base)(height)
=12(R)(Rdθ)
dA=12R2dθ
Magnetic flux cut by the rod in time dt is ,
dϕ=B(dA)
∴dϕ=B(12R2dθ)
∴dϕdt=12BR2dθdt
∴dϕdt=12BR2ω (∵ω=dθdt = constant angular speed of rod )
Now, induced emf across the rod will be ,
ε=−dϕdt
∴ε=−12BωR2
∴|ε|=12BωR2
Third Method :
At time t=0 , ε1=0(∵v=0⇒ε=Bvl=0)
At time t=t, ε2=Bvl ( ∵ For l=R, (v=Rω))
=B(Rω)R
ε2=BωR2
∴ Average induced emf
<ε>=ε1+ε22=0+BωR22
∴<ε>=12BωR2
Now , value of induced emf across the rod in the present situation will be ,
ε=12BωR2
=12B(2πf)R2(∵ω=2πf where f=frequency of rotation)
=(1)(3.14)(50)(1)2
∴ε=157 V
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Numerical From Textual Exercise17videos
ELECTROMAGNETIC INDUCTION
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Numerical From DARPAN Based on Textbook10videos
ELECTROMAGNETIC INDUCTION
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section A HOTS2videos
ELECTROMAGNETIC INDUCTION
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION D MCQS ASKED IN COMPETITIVE EXAMES (MCQS AKSED IN BOARD EXAM AND GUJCET)14videos
ELECTRIC CHARGES AND FIELDS
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - BOARD.S QUESTION PAPER MARCH - 2020 (PART - B) SECTION - C5videos
ELECTROMAGNETIC WAVES
Similar Questions
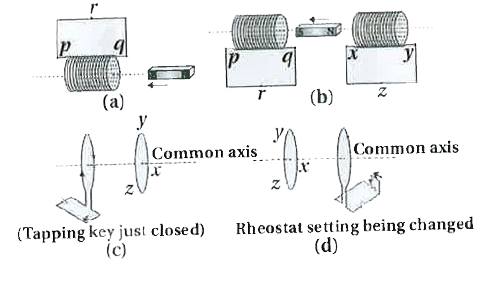
Predict the direction of induced current in the situations described by the following figures (a) to (d).
 06:37View Solution
06:37View SolutionA conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the field. Some how, the radius of the loop starts shrinking at a constant rate of 2 mm/s. Find the induced emf in the loop at an instant when the radius becomes 2 cm solution.
View SolutionGive the explanation of reason behind the origin of motional induced emf.
06:37View SolutionWhen air is replaced by dielectric medium of constant K, the maximum force of attraction between two charges separated by distance d, ..........
04:37View SolutionDetermine the current in each branch of the given network.
 05:42View Solution
05:42View SolutionA metallic rod of 1 m length is rotated with a frequency of 50 rev/s, with on end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular at to the plane of the ring. A constant uniform magnetic field of 1 T parallel to the axis is persent eveywhere. what is the e.m.f. between the centre and the metallic ring?
02:05View SolutionA metallic rod of length 'I' is rotated with a frequency with one end hinged at the center and the other end at the circumference of a circular metallic ring of radius r , about an axis passing through the centre and perpendicular to the plane of the ring. A constant uniform magnetic field B parallel to the axis is present everywhere . Using Lorentz force, explain how emf is induced between the centre and the metallic ring and hence obtained the expression for it.
04:04View Solution1 मीटर लंबी धातु की एक छड़ को 50 चक्कर/सेकंड की आवृति से घुमाया गया है | छड़ के एक सिरा वृताकार धात्विक वलय जिसकी त्रिज्या 1 मीटर है, के केंद्र पर तथा दूसरा सिरा वलय की परिधि पर कब्जे से इस प्रकार जुड़ा है कि छड़ की गति वलय के केंद्र से जाने वाले तथा वलय के ताल में अभिलंबावत अक्ष के पारित: है (चित्र 6.11) | अक्ष के अनुदिश एक स्थिर तथा एकसमान चुंबकीय क्षेत्र 1 T सर्वत्र उपस्थित है | केंद्र तथा धात्विक वलय के बिच विधुत वाहक बल क्या होगा ?
 04:25View Solution
04:25View SolutionA metallic rod of 'L' length is rotated with angular frequency of 'w' with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius L, about an axis passing through the centre and perpendicular to the plane of the ring. A constant and uniform magnetic field B parallel to the axis is present everywhere. Deduce the expression for the emf between the centre and the metallic ring.
03:05View SolutionA metallie rod of 1 m length is rotated with a frequency of 50 revis, with one end hinged at the centre and the other end at the eireumference of a circular metallic ring of radius 1m, about an axis passing through the centre and perpendicular to the plane of the ring as in figure. A constant and uniform magnetic field of the parallel to the axis is present everywhere. What is the emf between the centre and the metallic ring?
 06:14View Solution
06:14View Solution1.0 m लंबी धातु की छड़ उसके एक सिरे से जाने वाले अभिलंबवत अक्ष के परितः 400rads^(-1)की कोणीय आवृत्ति से घूर्णन कर रही है। छड़ का दूसरा सिरा एक धात्विक वलय से संपर्कित है। अक्ष के अनुदिश सभी जगह 0.5 T का एकसमान चुंबकीय क्षेत्र उपस्थित है। वलय तथा अक्ष के बीच स्थापित विद्युत वाहक बल की गणना कीजिए।
04:33View SolutionA metallic rod of 1m length is rotated with a frequency of 50 rev/s, with one end hinged at the centre and the other end at the circumference of a circular me tallic ring of radius 1m, about an axis passing through the centre and perpendicular to the plane of the ring (see figure). A constant and uniform magnetic field of 1T parallel to the axis present everywhere. What is the emf between the centre and the metallic ring?
02:07View SolutionA metallic rod of 1 m length is rotated with a frequency of 50 rev/s, with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular to the plane of the ring (Fig.). A constant and uniform magnetic field of 1 T parallel to the axis is present everywhere. What is the emf between the centre and the metallic ring?
View SolutionA metallic rod of 1 m length is rotated with a frequency of 50 rev/s, with on end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular at to the plane of the ring. A constant uniform magnetic field of 1 T parallel to the axis is persent eveywhere. what is the e.m.f. between the centre and the metallic ring?
View SolutionA metallic rod of 'L' length is rotated with angular frequency of 'w' with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius L, about an axis passing through the centre and perpendicular to the plane of the ring. A constant and uniform magnetic field B parallel to the axis is present everywhere. Deduce the expression for the emf between the centre and the metallic ring.
View Solution

