A metallic wire A of radius 1 mm is joined at one end with another metallic wire B of radius 2 mm .
when a battery is connected between two free ends of this composite wire, a currents of 20 A flows through it.
Calculate the currents density in wire A and also calculate the drift speed of electrons in wire B , if material of B has 9×1029 free electrons per unit volume.
To solve the problem step-by-step, we will calculate the current density in wire A and the drift speed of electrons in wire B.
Step 1: Calculate the Current Density in Wire A
Given:
- Radius of wire A, rA=1mm=1×10−3m
- Current through the composite wire, I=20A
Formula for Current Density (J):
JA=IAA
where AA is the cross-sectional area of wire A.
Calculate the Cross-Sectional Area of Wire A:
AA=πr2A=π(1×10−3)2=π×10−6m2
Now, substitute the values into the current density formula:
JA=20π×10−6≈203.14×10−6≈6.366×106A/m2
Step 2: Calculate the Drift Speed of Electrons in Wire B
Given:
- Radius of wire B, rB=2mm=2×10−3m
- Free electrons per unit volume in wire B, n=9×1029m−3
- Charge of an electron, e=1.6×10−19C
Calculate the Cross-Sectional Area of Wire B:
AB=πr2B=π(2×10−3)2=π×4×10−6m2
Using the formula for drift speed (v_d):
I=neABvd
Rearranging for vd:
vd=IneAB
Substituting the values:
vd=20(9×1029)(1.6×10−19)(π×4×10−6)
Calculating the denominator:
neAB=(9×1029)(1.6×10−19)(π×4×10−6)≈9×1029×1.6×10−19×12.566×10−6
≈1.81×106
Now calculate vd:
vd=201.81×106≈1.10×10−5m/s
Final Answers:
- Current Density in Wire A, JA≈6.366×106A/m2
- Drift Speed of Electrons in Wire B, vd≈1.10×10−5m/s
- Radius of wire A, rA=1mm=1×10−3m
- Current through the composite wire, I=20A
JA=IAA
where AA is the cross-sectional area of wire A.
AA=πr2A=π(1×10−3)2=π×10−6m2
JA=20π×10−6≈203.14×10−6≈6.366×106A/m2
- Radius of wire B, rB=2mm=2×10−3m
- Free electrons per unit volume in wire B, n=9×1029m−3
- Charge of an electron, e=1.6×10−19C
AB=πr2B=π(2×10−3)2=π×4×10−6m2
I=neABvd
Rearranging for vd:
vd=IneAB
vd=20(9×1029)(1.6×10−19)(π×4×10−6)
neAB=(9×1029)(1.6×10−19)(π×4×10−6)≈9×1029×1.6×10−19×12.566×10−6
≈1.81×106
vd=201.81×106≈1.10×10−5m/s
- Current Density in Wire A, JA≈6.366×106A/m2
- Drift Speed of Electrons in Wire B, vd≈1.10×10−5m/s
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE PROBLEMS
71 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - CONCEPTUAL QUESTIONS
35 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
There is a copper wire of radius 1mm and it carries a current of 2 ampere, calculate the drift speed of electrons assuming there are 8.5×1028 free electrons per metre cube.
View SolutionIf 30 coulomb of electric charge flows through a wire in two minutes, calculate the current in the wire ?
View Solution
Knowledge Check
In the circuit shown, if a wire is connected between points A and B. How much current will flow through that wire?
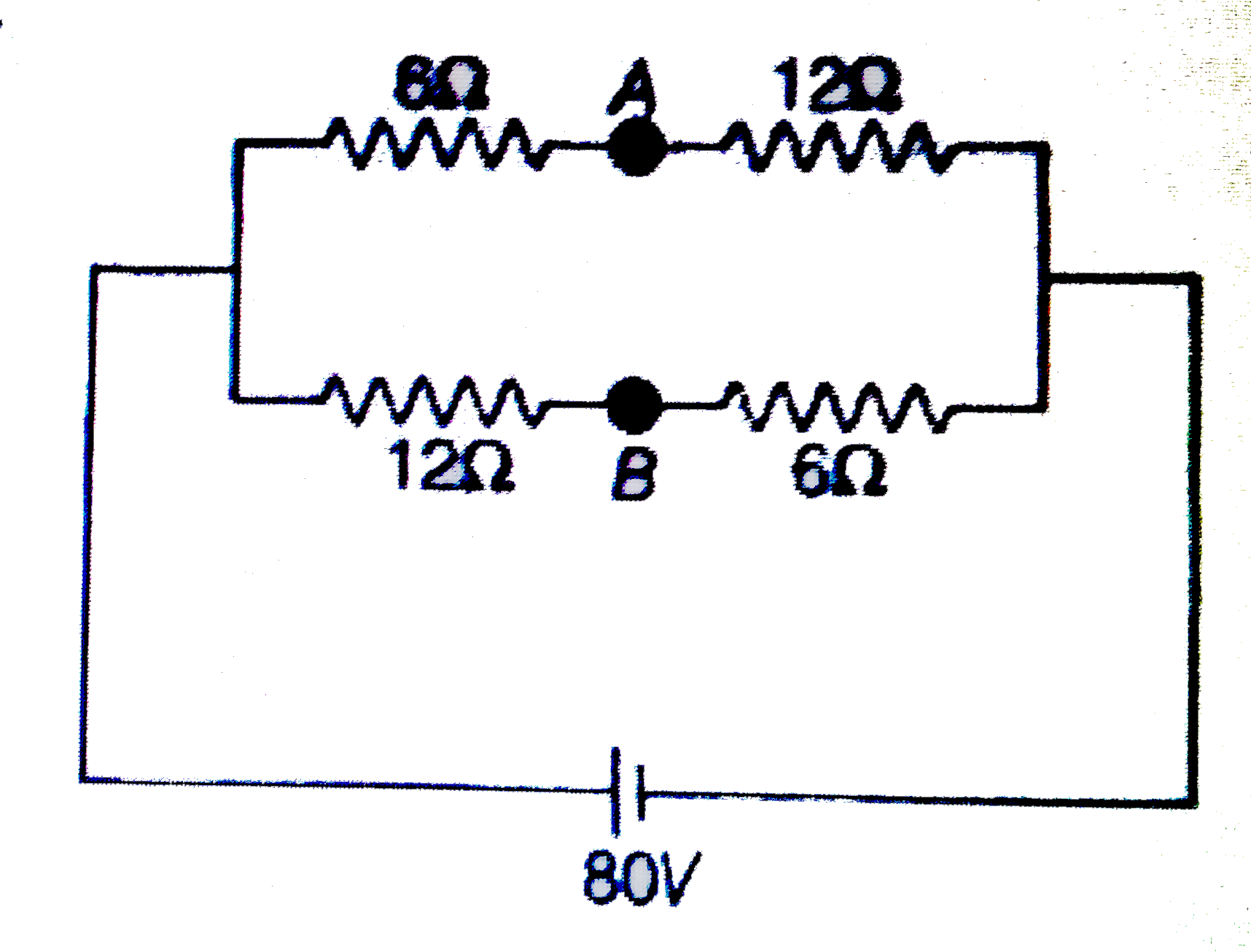 A5AB103AC203AD53A
A5AB103AC203AD53AA current of 2 amp. flows in a long, straight wire of radius 2mm. The intensity of magnetic field on the axis of the wire is
A(μ0π)×103 TeslaB(μ02π)×103 TeslaC(2μ0π)×103 TeslaDZeroIn a wire of cross-section radius r, free electrons travel with drift velocity v when a current I flows through the wire. What is the current in another wire of half the radius and of the same material when the drift velocity is 2v?
A2IBICI/2DI/4
A current of 5 amperes flows through a wire whose ends are at a potential difference of 3 volts. Calculate the resistance of the wire.
View SolutionA thin copper rod of radius 1mm having an electrical resistance of 100Ω is connected to battery of emf 12 volts and negligible internal resistance through a wire of negligible resistance. Calculate current density in the wire. Also , calculate the number of electrons entering one and of the rod per seconds.
View SolutionIf a current of 0.5A flows through a metallic wire for 2 hours, then how many electrons would flow through the wire ?
View SolutionAn aluminium wire of diameter 2.5 mm is connectedf in series with a copper wire of diameter1.6 mm. A current of 2.0 A is passed through them. Find (a) current density in aluminium wire, (b) drift velocity of electrons in copper wire. Given the number density of conduction electrons in copper is 1029m−3.
View SolutionA current of "1.6A" is flowing through "h" wire having cross- sectional area 1mm2 .If density of free electrons in the material of the wire is 1029 per m3 ,the drift velocity of electrons will be:-
View Solution
