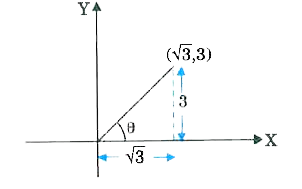
A paricle starting from the origin (0,0) moves in a straight line in $(x, y)$ plane. Its coordinates at a later time are $(\sqrt{3}, 3)$ . The path of the particle makes with the x-axis an angle of

$45^{\circ}$

$60^{\circ}$

$0^{\circ}$

$30^{\circ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

A particle starting from the origin (0,0) moves in a straight line in the (x,y) plane. Its coordinates at a later time are $(\sqrt{3}, 3)$ . The path of the particle makes with the x-axis an angle of
A $30^{\circ}$
B $45^{\circ}$
C $60^{\circ}$
D $0^{\circ}$
A particle starting from the origin (0,0) moves in a straight line in the (x,y) plane. Its coordinates at a later time are $(\sqrt{3}, 3)$ . The path of the particle makes with the x-axis an angle of
A $30^{\circ}$
B $45^{\circ}$
C $60^{\circ}$
D $0^{\circ}$
A paricle starting from the origin (0,0) moves in a straight line in $(x, y)$ plane. Its coordinates at a later time are $(\sqrt{3}, 3)$ . The path of the particle makes with the x-axis an angle of
A $45^{\circ}$
B $60^{\circ}$
C $0^{\circ}$
D $30^{\circ}$