A particle is moving uniformly in a circular path of radius r. When it moves through an angular displacement θ, then the magnitude of the corresponding linear displacement will be
A
2rcos(θ2)
B
2rcos(θ2)
C
2rtan(θ2)
D
2rsin(θ2)
Video Solution
Text Solution
The correct Answer is:D
According to cosine formula
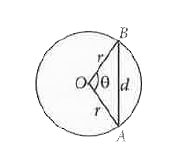
cosθ=r2+r2−d22r2
22cosθ=r2+r2−d2
d2=2r2cosθ=2r2[1−cosθ]
[∴cosθ=1−2sin2θ2]
or d=2rsin(θ)2
∴ Linear displacement, AB=2rsin(θ)2
|
Topper's Solved these Questions
- LAWS OF MOTION BOOK - MTG-WBJEECHAPTER - LAWS OF MOTION EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 2: SINGLE OPTION CORRECT TYPE (2 MARKS))
1 Videos
- LAWS OF MOTION BOOK - MTG-WBJEECHAPTER - LAWS OF MOTION EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3: ONE OR MORE THAN ONE OPTION CORRECT TYPE (2 MARKS))
2 Videos
- LAWS OF MOTION BOOK - MTG-WBJEECHAPTER - LAWS OF MOTION EXERCISE - WB JEE WORKOUT (CATEGORY 3: ONE OR MORE THAN ONE OPTION CORRECT TYPE (2 MARKS))
10 Videos
- KINETIC THEORY OF GASES BOOK - MTG-WBJEECHAPTER - KINETIC THEORY OF GASES EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (MCQ)
7 Videos
- MAGNETIC EFFECT OF CURRENTBOOK - MTG-WBJEECHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - WB JEE Previous Years Questions
22 Videos
Similar Questions
Knowledge Check
A particle is moving on a cicular path of radius 'R' . As it moves through an angular displacement θ, its linear displacement will be :
ARsinθB2Rcosθ/2C2Rsinθ/2DRcosθA particle moves through angular displacement θ on a circular path of radius' r'. The linear displacement will be:
A2rsin(θ2)B2rcos(θ2)C2rtan(θ2)D2rcot(θ2)A particle moves throught angular displacement θ on a circlur path of radius r. The liner displacement wil be
A2rsin(θ/2)B2rcos(θ/2)C2rtan(θ/2)D2rcot(θ/2)
A particle is moving in a circular path of radius r. The displacement after half a circle would be
View SolutionA particle is moving in a circular path of radius r. The displacement after half a circle would be
View SolutionA particle is moving in a circular path of radius 'r'. The displacement after half a circle would be
View SolutionA particle moves along a circular path of radius R. The distance and displacement of a particle after one completer revolution is
View SolutionA particle is moving along a circular path with uniform speed. Through what angle does its angular velocity change when it completes half of the circular path?
View Solution
