A particle is projected horizontally will speed 20ms−1 from the top of a tower. After what time velocity of particle will be at 45∘ angle from initial direction of projection.
1s
2s
3s
4s
The correct Answer is:B
To solve the problem of a particle projected horizontally from the top of a tower, we need to determine the time at which the velocity of the particle makes a 45-degree angle with its initial direction of projection. Here's the step-by-step solution:
Step 1: Understand the motion of the particle
The particle is projected horizontally with an initial speed of u=20m/s. Since it is projected horizontally, its initial vertical velocity vy0=0.
Step 2: Analyze horizontal and vertical motions
- The horizontal velocity vx remains constant because there is no horizontal acceleration:
vx=20m/s
- The vertical velocity vy increases due to gravitational acceleration g:
vy=g⋅t
where g is approximately 10m/s2 (taking the value of gravitational acceleration).
Step 3: Set up the condition for a 45-degree angle
For the resultant velocity to make a 45-degree angle with the horizontal, the magnitudes of the horizontal and vertical velocities must be equal:
vy=vx
Substituting the expressions we have:
g⋅t=20m/s
Step 4: Solve for time t
Using g=10m/s2:
10⋅t=20
t=2010=2s
Final Answer
The time after which the velocity of the particle will be at a 45-degree angle from the initial direction of projection is t=2s.
---
The particle is projected horizontally with an initial speed of u=20m/s. Since it is projected horizontally, its initial vertical velocity vy0=0.
- The horizontal velocity vx remains constant because there is no horizontal acceleration:
vx=20m/s
vy=g⋅t
where g is approximately 10m/s2 (taking the value of gravitational acceleration).
For the resultant velocity to make a 45-degree angle with the horizontal, the magnitudes of the horizontal and vertical velocities must be equal:
vy=vx
g⋅t=20m/s
Using g=10m/s2:
10⋅t=20
t=2010=2s
The time after which the velocity of the particle will be at a 45-degree angle from the initial direction of projection is t=2s.
Topper's Solved these Questions
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - A. Taking it together
1 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Taking it together
96 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Check Point 4.2
20 Videos
- MEASUREMENT AND ERRORSBOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
Similar Questions
A particle is projected horizontally with speed 20√3m/s, from some height at t=0. At what time its velocity will make 60∘ angle with initial velocity ?
View SolutionA particles is projected horizontally with a speed v from the top of a plane inclined at an angle tehta to the horizontal as shown in the figure.
(a) Hwo far from the point of projection will the particle strike the plane ?
(b) Find the time taken by the particel to hit the plane.
(c) What is the velocity of particle just before it hits the plane ?
View SolutionA body is projected horizontally with a velocity of 4ms−1 from the top of a high tower. The velocity of the body after 0.7 is nearly (Take, g=10ms−2)
View SolutionA particle is projected up with initial speed u=10ms−1 from the top of a bitlding at time t=0. At time t=5s the particle strikes the fround. Find the height of the building.
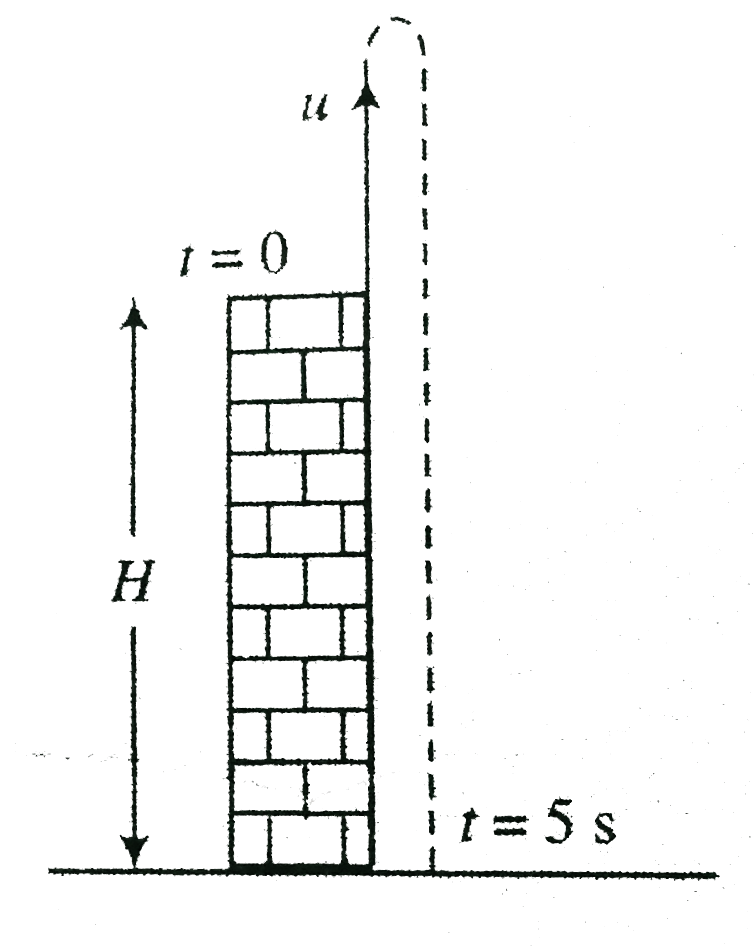 .
.
View SolutionA ball is projected horizontal from the top of a tower with a velocity v0. It will be moving at an angle of 60∘ with the horizontal after time.
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View SolutionA particle is projected with speed 20ms−1 at an angle 30∘ With horizontal. After how much time the angle between velocity and acceleration will be 90∘
View SolutionA particle is projected from horizontal making an angle of 53∘ with initial velocity 100ms−1. The time taken by the particle to make angle 45∘ from horizontal is
View SolutionA particle is projected at an angle θ with an initial speed u.
View SolutionA particle of mass 50 g is projected horizontally from the top of a tower of height 50 m with a velocity 20m/s. If g=10m/s2, then find the :-
View Solution
