A particle is suspended vertically from a point O by an inextensible mass less string of length L. A vertical line AB is at a distance of L8 from O as shown in figure. The particle is given a horizontal velocity u. At some point, its motion ceases to be circular and eventually the object passes through the line AB. At the instant of crossing AB, its velocity is horizontal. Find u.
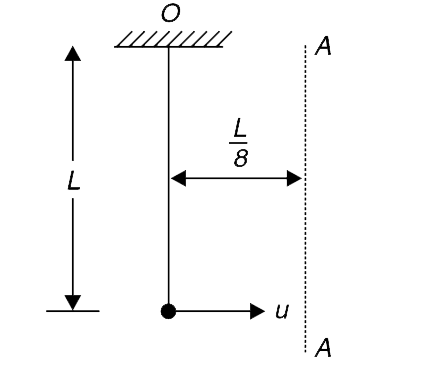
It is clear from the statement of the problem that the particle has the circular path before it crosses the line AB second time, during its upward motion.
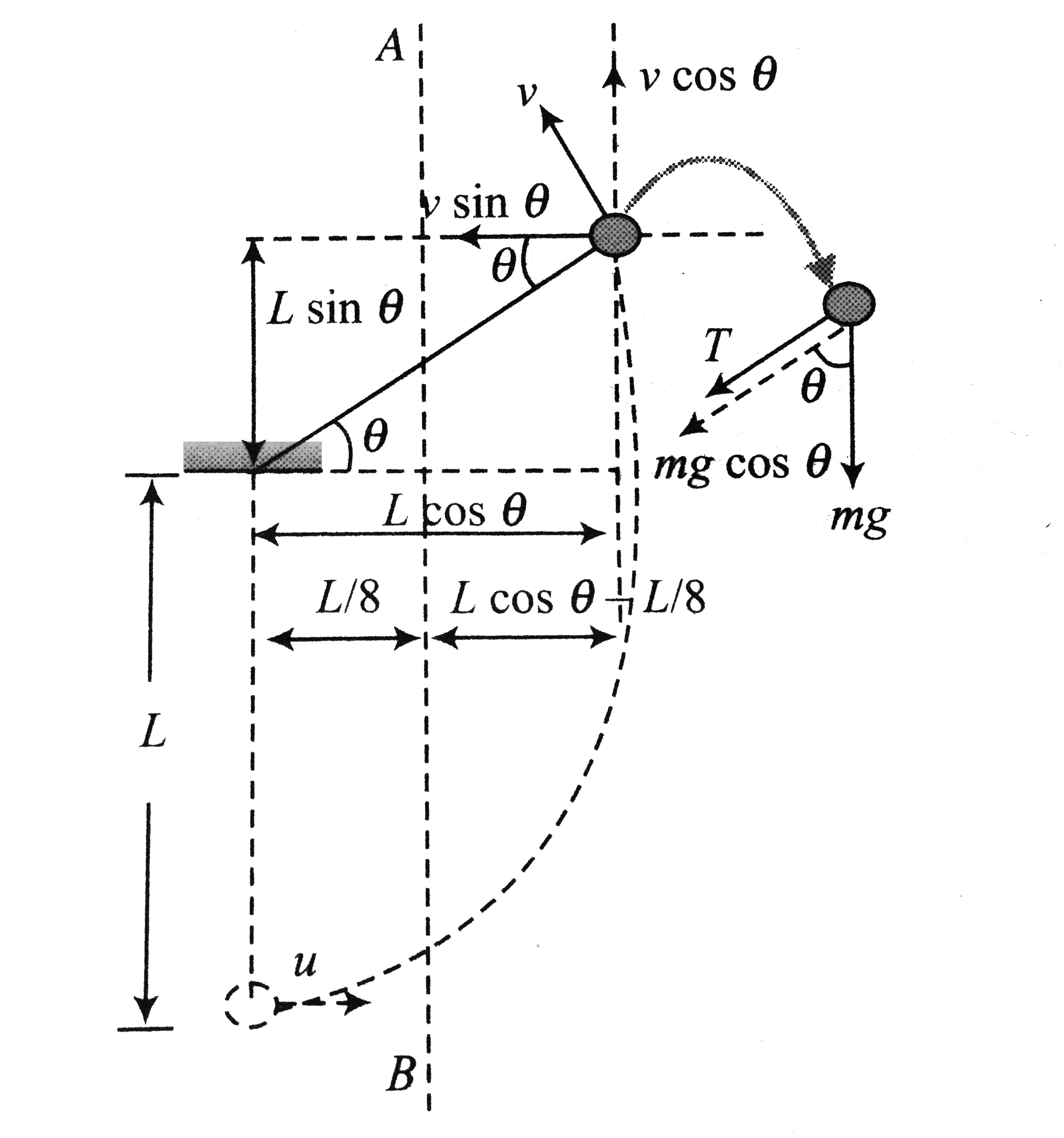
Let us assume that the particle have the circular path at P and v is the velocity of the particle at P. Then, conservation of mechanical energy at P and at the lowest point yields:
12u2=12mv2+mgL(1+sinθ)
μ2=2gL(1+sinθ)+mv2 (i)
At point T+mgsinθ=mv2L.
If T becomes zero:
v2=gLsinθ (ii)
After having the circular path, the particle moves along a parabolic trajectory as a projectile.
As it is given that velocity of the particle when it crosses AB is horizontal, it is clear that, when it is crossing AB, it is passing through its highest point in the parabolic path.
Hence, half of the range of this projectile path =Lcosθ−L8
As projection angle of the particle with respect to horizontal time at
P=(90∘−θ), therefore, maximum range =v2sin2(90∘−θ)8
Hence, 12×v2sin2(90∘−θ)g=Lcosθ−L8 (iii)
From Eq. (ii) and (iii): cosθ=12⇒θ=60∘
Using this information and (I) and (ii),
u=
⎷[4+3√32gL]
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.2
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A particle is moving with constant speed v in xy plane as shown in figure. The magnitude of its angular velocity about point O is

View SolutionTwo point masses m are connected the light rod of length l and it is free to rotate in vertical plane as shown in figure. Calculate the minimum horizontal velocity is given to mass so that it completes the circular motion in vertical lane.
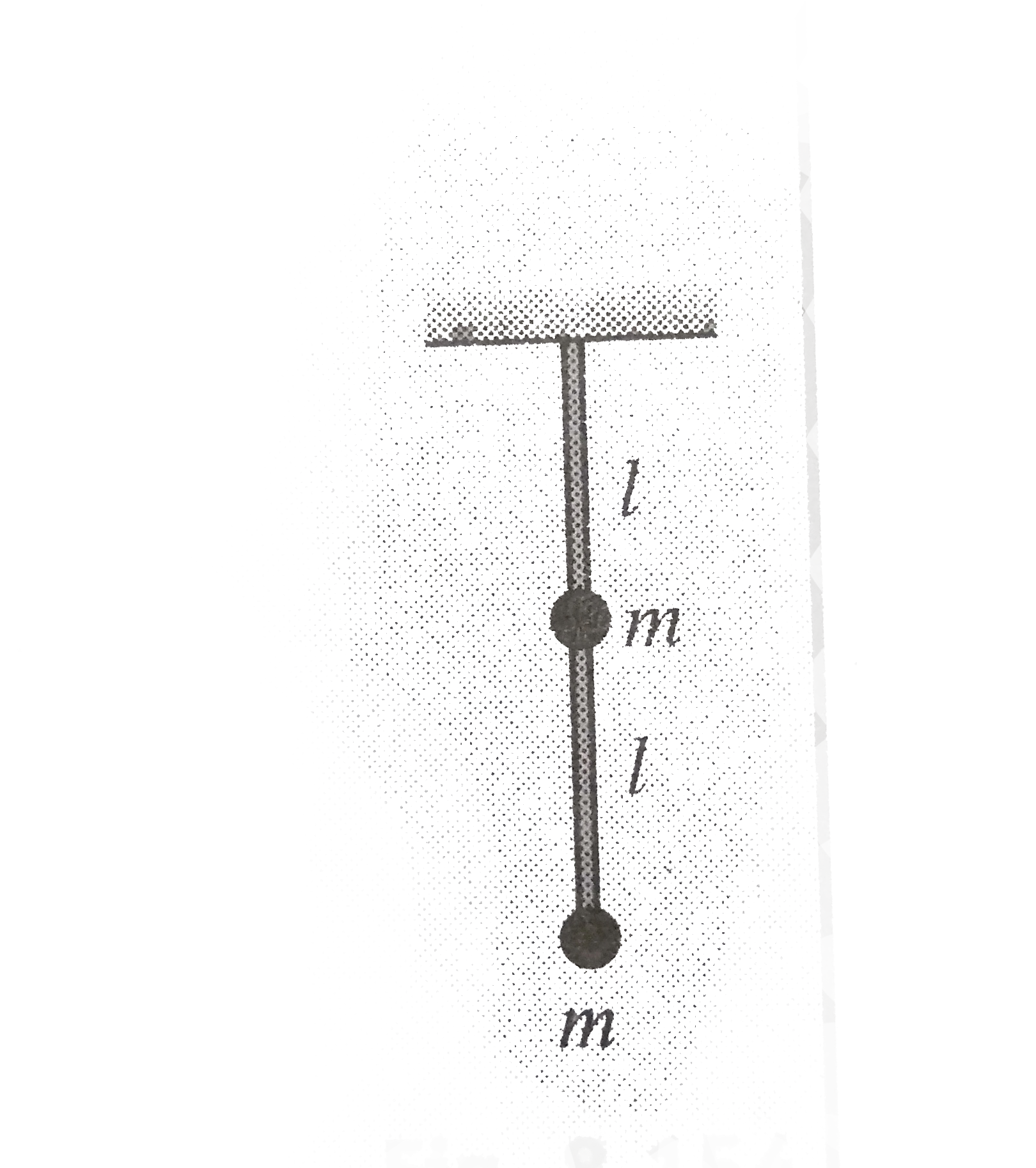
View SolutionA particle is projected vertically upward with velocity u from a point A, when it returns to the point of projection .
View SolutionA particle suspended from a fixed point, by a light inextensible thread of length L is projected horizontally from its lowest position with velocity 7gL . The thread will slack after swinging through an angle , such that equal
View SolutionA particle of mass m is attached to one end of a light inextensible string and the other end of the string is fixed in vertical plane as shown. Particle is given a horizontal velocity u=√52gl

The maximum angle made by the string with downward vertical is
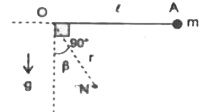
View SolutionA point mass m is connected with ideal inextensible string of length l and other end of string is tied at point O as shown in the figure. The string is made horizontal and mass is released from point A to perform vertical circular motion. There is a nail at point N such that ON =r . Find the value of r so that mass m is able to just perform complete vertical circular motion about point N.
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizontally with speed (gl) . Then the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string equal the weight of the particle-
View SolutionTwo masses A and B connected with an inextensible string of length l lie on a smooth horizontal plane. A is given a velocity of vm/s along the ground perpendicular to line AB as shown in figure. Find the tension in string during their subsequent motion
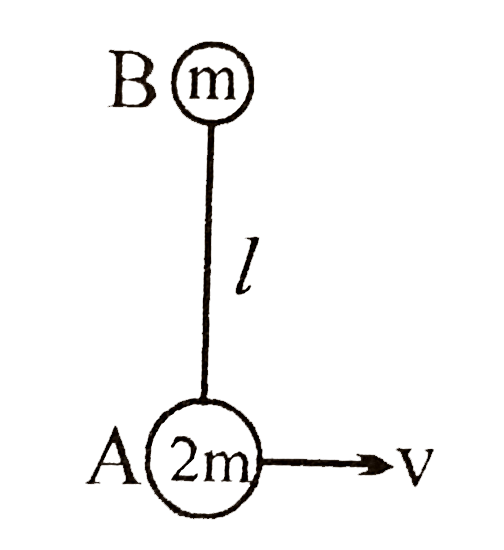
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizonally with speed √gl . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
View SolutionA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is

View Solution
