A particle of mass m is lying on smooth horizontal table. A constant force F tangential to the surface is applied on it. Find .
(a) average power over a time interval from to
(b) instantaneous power as function of time t.
The correct Answer is:B
To solve the problem, we will break it down into two parts: (a) finding the average power over a time interval from t=0 to t=t, and (b) finding the instantaneous power as a function of time t.
Part (a): Average Power
1. Identify the Given Information:
- Mass of the particle: m
- Constant force applied: F
- Time interval: from t=0 to t=t
2. Determine the Acceleration:
- According to Newton's second law, the acceleration a of the particle can be calculated as:
a=Fm
3. Calculate the Velocity at Time t:
- Since the particle starts from rest (u=0), we can use the equation of motion:
v=u+at
- Substituting the values, we get:
v=0+(Fm)t=Ftm
4. Calculate the Work Done:
- The work done W on the particle is equal to the change in kinetic energy. The kinetic energy KE at time t is given by:
KE=12mv2
- Substituting the expression for v:
KE=12m(Ftm)2=12m⋅F2t2m2=F2t22m
5. Calculate the Average Power:
- Average power Pavg is defined as the work done divided by the time taken:
Pavg=Wt=F2t22mt=F2t2m
Part (b): Instantaneous Power
1. Define Instantaneous Power:
- The instantaneous power PI is given by the formula:
PI=F⋅v⋅cos(θ)
- Since the force is applied tangentially to the surface, θ=0 degrees, and thus cos(0)=1:
PI=F⋅v
2. Substitute the Expression for Velocity:
- We already found that:
v=Ftm
- Substituting this into the power equation gives:
PI=F⋅(Ftm)=F2tm
Final Answers:
- (a) Average Power:
Pavg=F2t2m
- (b) Instantaneous Power:
PI=F2tm
- Mass of the particle: m
- Constant force applied: F
- Time interval: from t=0 to t=t
- According to Newton's second law, the acceleration a of the particle can be calculated as:
a=Fm
- Since the particle starts from rest (u=0), we can use the equation of motion:
v=u+at
- Substituting the values, we get:
v=0+(Fm)t=Ftm
- The work done W on the particle is equal to the change in kinetic energy. The kinetic energy KE at time t is given by:
KE=12mv2
- Substituting the expression for v:
KE=12m(Ftm)2=12m⋅F2t2m2=F2t22m
- Average power Pavg is defined as the work done divided by the time taken:
Pavg=Wt=F2t22mt=F2t2m
- The instantaneous power PI is given by the formula:
PI=F⋅v⋅cos(θ)
- Since the force is applied tangentially to the surface, θ=0 degrees, and thus cos(0)=1:
PI=F⋅v
- We already found that:
v=Ftm
- Substituting this into the power equation gives:
PI=F⋅(Ftm)=F2tm
- (a) Average Power:
Pavg=F2t2m
- (b) Instantaneous Power:
PI=F2tm
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Solved Examples
12 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - TYPE2
1 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
A block of mass 1kg start moving with constant acceleration a=4m/s2 Find.
(a) average power of the net force in time inteval from t=0 to t=2s,
(b) instantaneous power of the net force at t=4s.
View SolutionA time varying power P=2t is applied on particle of mass m. find.
(a) kinetic energy and velocity of particle as function of time.
(b) average power over a time intrval from t=0 to t=t.
View Solutionvelocity-time graph of a particle moving in a straight line is as shown in figure. At time t=0, velocity of the particle is zero. Find
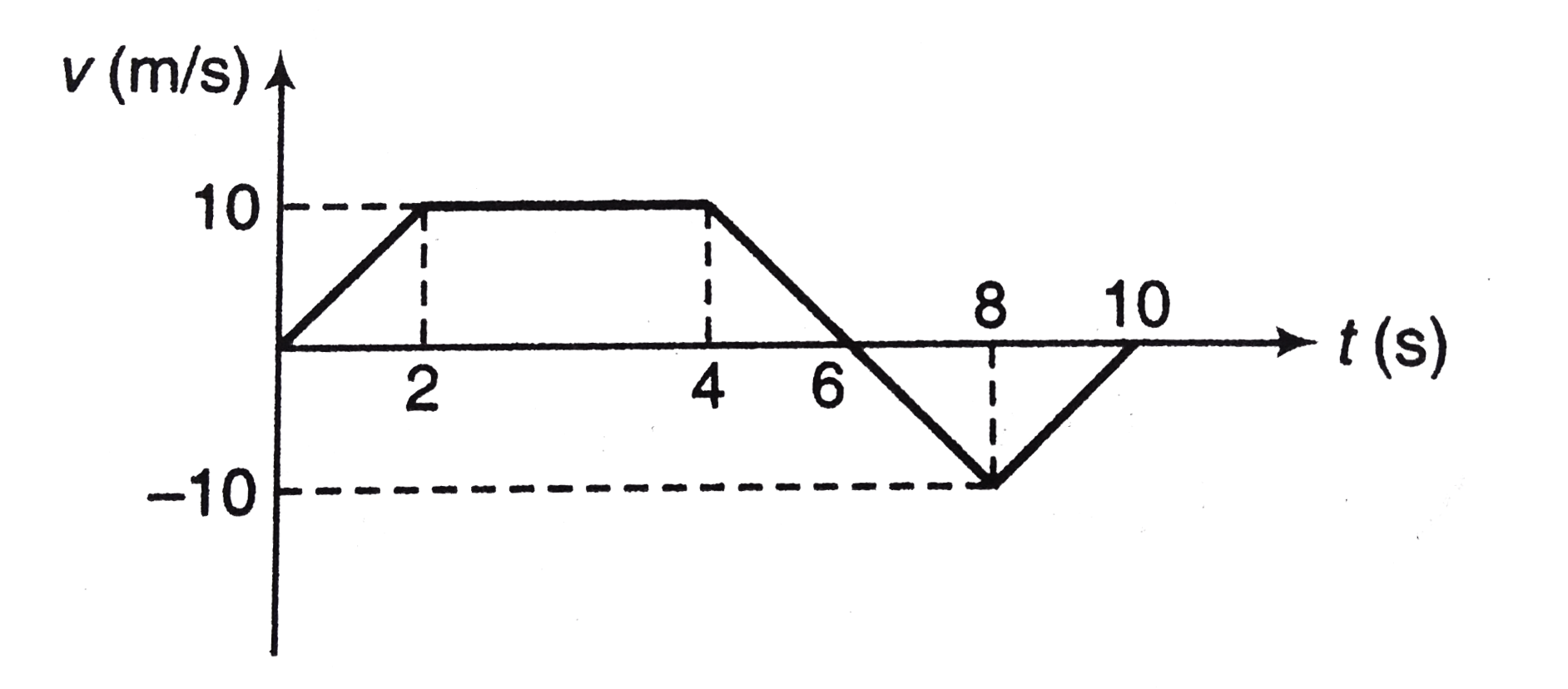
(a) average acceleration in a time interval from t=6s to t=12s,
(b) velocity of the particle at t=14s.
View SolutionA particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
View SolutionAn object of mass m, initially at rest under the action of a constant force F attains a velocity v in time t. Then, the average power supplied to mass is
View SolutionA particle of mass m is driven by a machine that delivers a constant power k watts. If the particle starts from rest the force on the particle at time t is
View SolutionA particle moves rectilinearly possessing a parabolic s-t graph. Find the average velocity of the particle over a time interval from t=12s→t=1.5s.
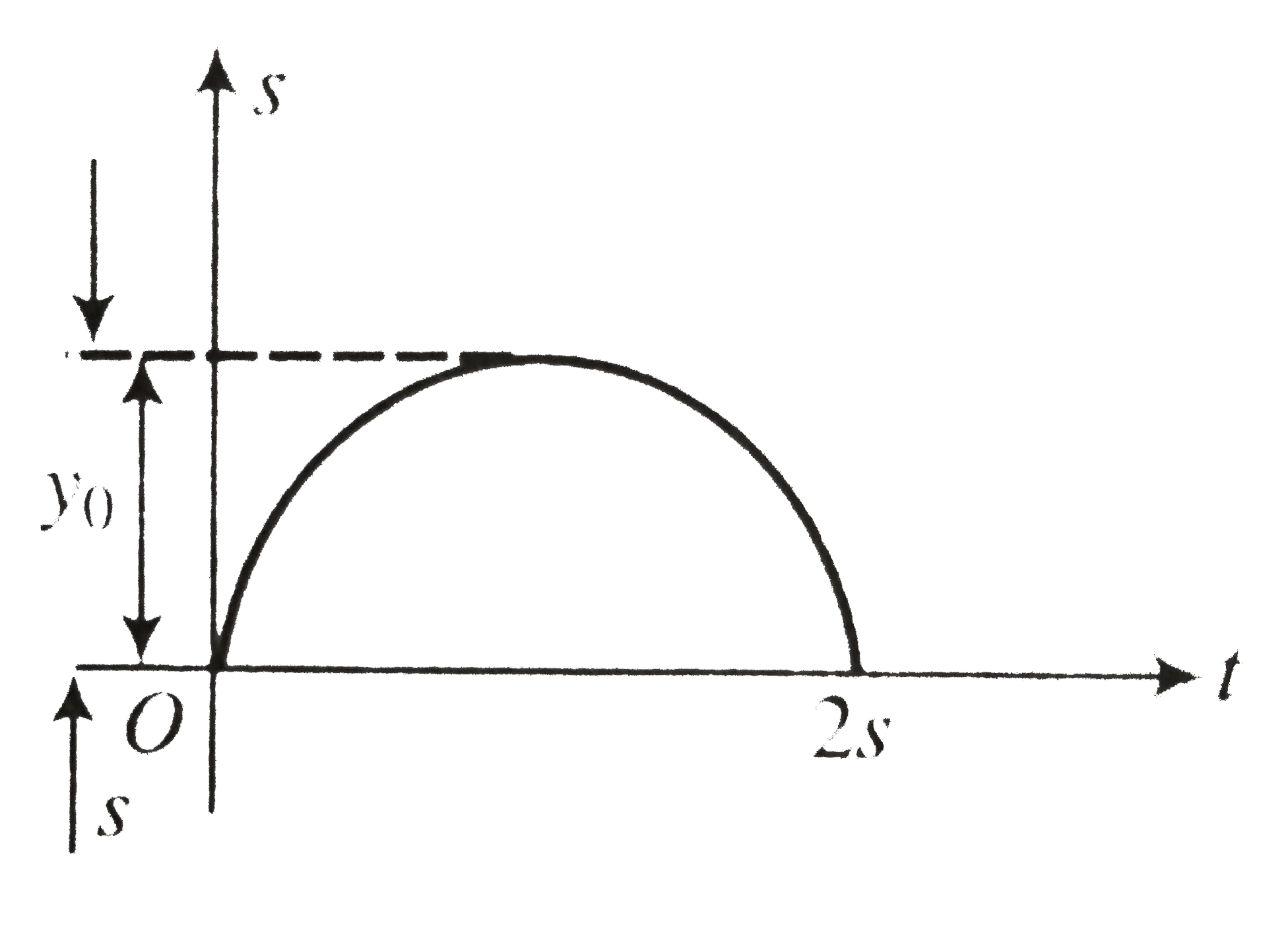
View SolutionA constant power P is applied on a particle of mass m. find kintic energy, velocity and displacement of particle as function of time t.
View SolutionA particle is moving in a circle of radius 4cm with constant speed of 1cm/s. Find
(a) time period of the particle.
(b) average speed, average velocity and average acceleration in a time interval from t=0 to t=T4. Here, T is the time period of the particle. Give only their magnitudes.
View SolutionA particle is kept at rest at the origin. A constant force →F starts acting on it at t=0. Find the speed of the particle at time t.
View Solution
