A particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
The correct Answer is:A, B
To find the average velocity of a particle moving rectilinearly with an initial velocity u and constant acceleration a over a time interval from t=0 to t=t seconds, we can follow these steps:
Step 1: Understand the formula for average velocity
The average velocity vavg is defined as the total displacement s divided by the total time t:
vavg=st
Step 2: Determine the expression for total displacement
The total displacement s of the particle can be calculated using the kinematic equation:
s=ut+12at2
where:
- u is the initial velocity,
- a is the constant acceleration,
- t is the time interval.
Step 3: Substitute the expression for displacement into the average velocity formula
Now, we substitute the expression for s into the average velocity formula:
vavg=ut+12at2t
Step 4: Simplify the expression
We can simplify the equation:
vavg=utt+12at2t
This simplifies to:
vavg=u+12at
Final Result
Thus, the average velocity of the particle over the time interval from t=0 to t=t seconds is:
vavg=u+12at
---
The average velocity vavg is defined as the total displacement s divided by the total time t:
vavg=st
The total displacement s of the particle can be calculated using the kinematic equation:
s=ut+12at2
where:
- u is the initial velocity,
- a is the constant acceleration,
- t is the time interval.
Now, we substitute the expression for s into the average velocity formula:
vavg=ut+12at2t
We can simplify the equation:
vavg=utt+12at2t
This simplifies to:
vavg=u+12at
Thus, the average velocity of the particle over the time interval from t=0 to t=t seconds is:
vavg=u+12at
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.65videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.73videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.42videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
Similar Questions
A particle moves rectilinearly possessing a parabolic s-t graph. Find the average velocity of the particle over a time interval from t=12s→t=1.5s.
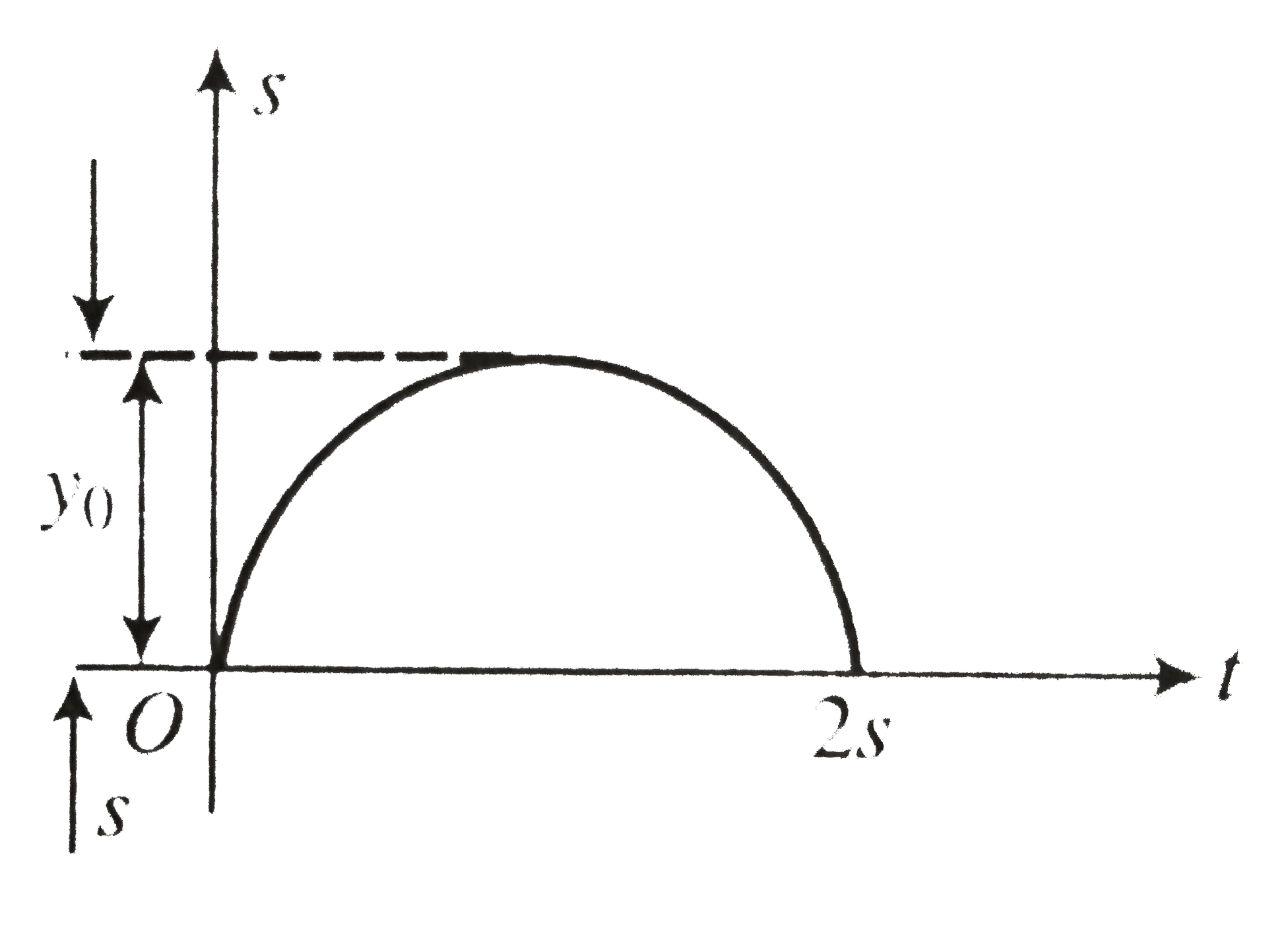 View Solution
View SolutionA particle moves with initial velocity v0 and retardation αv, where v is velocity at any instant t. Then the particle
View SolutionStatement-I : A particle moves in a straight line with constant acceleration. The average velocity of this particle cannot be zero in any time interval.
Statement-II : For a particle moving in straight line with constant acceleration, the average velocity in a time interval is u+v2, where u and v are initial and final velocity of the particle of the given time interval.View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionIf a particle starts moving with initial velocity u=1ms−1 and acceleration a=2ms−2, the veloctiy of the particle at any time is given by v=u+at=1+2t. Plot the velocity-time graph of the particle.
View SolutionA particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View SolutionA particle having initial velocity u moves with a constant acceleration a for a time t. a. Find the displacement of the particle in the last 1 second . b. Evaluate it for u=5m/s,a=2m/s2andt=10s.
View SolutionA particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View SolutionA particle starts from rest at t=0 and undergoes an acceleration as shown in figure.
Draw the velocity-time graph of the motion of the particle during the interval from zero to 4 second.
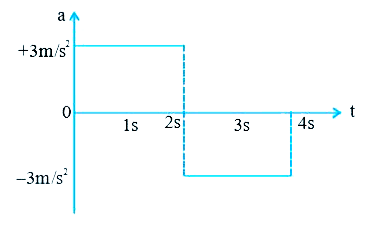 View Solution
View SolutionA particle is moving in a straight line with initial velocity u and uniform acceleration f. If the sum of the distances travelled in tthand(t+1)th seconds is 100cm, then its velocity after t seconds, in cm/s, is.
View Solution

