A particle of mass m starts to slide down from the top of the fixed smooth sphere. What is the tangential acceleration when it break off the sphere ?
2g3
√5g3
g
g3
The correct Answer is:B
To find the tangential acceleration of a particle of mass m when it breaks off from the surface of a fixed smooth sphere, we can follow these steps:
Step 1: Understand the Problem
The particle starts at the top of the sphere and slides down. We need to determine the tangential acceleration at the moment it leaves the surface of the sphere.
Step 2: Identify Forces Acting on the Particle
At any point while sliding down, the forces acting on the particle are:
- The gravitational force acting downward, which is mg.
- The normal force exerted by the surface of the sphere, which acts perpendicular to the surface.
Step 3: Resolve the Weight into Components
When the particle is at an angle θ from the vertical:
- The component of gravitational force acting tangential to the sphere is mgsin(θ).
- The component of gravitational force acting normal to the sphere is mgcos(θ).
Step 4: Use Energy Conservation to Find Velocity
Using the conservation of energy, we can find the velocity of the particle at the point just before it leaves the surface:
- The height h from which the particle falls is given by \( h = r - r \cos(θ) = r(1 - \cos(θ)) \).
- Using the energy conservation equation:
\(
\frac{1}{2}mv^2 = mg h
\)
Substituting for h gives:
\(
\frac{1}{2}mv^2 = mg(r(1 - \cos(θ)))
\)
Simplifying this, we find:
\(
v^2 = 2g r(1 - \cos(θ))
\)
Step 5: Find the Condition for Leaving the Surface
The particle will leave the surface when the normal force becomes zero. Therefore, we set up the equation:
\(
mg \cos(θ) = \frac{mv^2}{r}
\)
Substituting for v2 from the previous step:
\(
mg \cos(θ) = \frac{m(2g r(1 - \cos(θ)))}{r}
\)
This simplifies to:
\(
g \cos(θ) = 2g(1 - \cos(θ))
\)
Dividing by g and rearranging gives:
\(
\cos(θ) = \frac{2}{3}
\)
Step 6: Calculate Tangential Acceleration
The tangential acceleration at at the moment of leaving the surface is given by:
\(
a_t = g \sin(θ)
\)
Using the identity \( \sin^2(θ) + \cos^2(θ) = 1 \):
\(
\sin(θ) = \sqrt{1 - \cos^2(θ)} = \sqrt{1 - \left(\frac{2}{3}\right)^2} = \sqrt{1 - \frac{4}{9}} = \sqrt{\frac{5}{9}} = \frac{\sqrt{5}}{3}
\)
Thus,
\(
a_t = g \cdot \frac{\sqrt{5}}{3}
\)
Final Result
The tangential acceleration when the particle breaks off the sphere is:
\(
a_t = \frac{g \sqrt{5}}{3}
\)
The particle starts at the top of the sphere and slides down. We need to determine the tangential acceleration at the moment it leaves the surface of the sphere.
At any point while sliding down, the forces acting on the particle are:
- The gravitational force acting downward, which is mg.
- The normal force exerted by the surface of the sphere, which acts perpendicular to the surface.
When the particle is at an angle θ from the vertical:
- The component of gravitational force acting tangential to the sphere is mgsin(θ).
- The component of gravitational force acting normal to the sphere is mgcos(θ).
Using the conservation of energy, we can find the velocity of the particle at the point just before it leaves the surface:
- The height h from which the particle falls is given by \( h = r - r \cos(θ) = r(1 - \cos(θ)) \).
- Using the energy conservation equation:
\(
\frac{1}{2}mv^2 = mg h
\)
Substituting for h gives:
\(
\frac{1}{2}mv^2 = mg(r(1 - \cos(θ)))
\)
Simplifying this, we find:
\(
v^2 = 2g r(1 - \cos(θ))
\)
The particle will leave the surface when the normal force becomes zero. Therefore, we set up the equation:
\(
mg \cos(θ) = \frac{mv^2}{r}
\)
Substituting for v2 from the previous step:
\(
mg \cos(θ) = \frac{m(2g r(1 - \cos(θ)))}{r}
\)
This simplifies to:
\(
g \cos(θ) = 2g(1 - \cos(θ))
\)
Dividing by g and rearranging gives:
\(
\cos(θ) = \frac{2}{3}
\)
The tangential acceleration at at the moment of leaving the surface is given by:
\(
a_t = g \sin(θ)
\)
Using the identity \( \sin^2(θ) + \cos^2(θ) = 1 \):
\(
\sin(θ) = \sqrt{1 - \cos^2(θ)} = \sqrt{1 - \left(\frac{2}{3}\right)^2} = \sqrt{1 - \frac{4}{9}} = \sqrt{\frac{5}{9}} = \frac{\sqrt{5}}{3}
\)
Thus,
\(
a_t = g \cdot \frac{\sqrt{5}}{3}
\)
The tangential acceleration when the particle breaks off the sphere is:
\(
a_t = \frac{g \sqrt{5}}{3}
\)
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 More Than One Correct
5 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 Comprehension Based
5 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Level 1 Subjective
13 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A particle of mass m being to slide down a fixed smooth sphere from the top as shown. If its acceleration when it breaks off the sphere is 2a. The value of a is :
View SolutionA point mass m starts to slide from rest from the top of a smooth solid sphere of radius r. Calculate the angle at which the mass flies off the sphere. If there is friction between the mass and sphere, does the mass fly off at a greater or lesser angle ?
View Solution
Knowledge Check
A particle of mass m begins to slide down on a fixed smooth sphere from the top, when it breaks off the sphere, the tangential accleration will be
A2g/3BgC√53gDg2A particle of mass m is kept at the top of a smooth fixed sphere. It is given a horizontal velocity v then:
Ait will start moving along a circular path if v<√gRBit will start moving along a circular path if v>√gRCit will start moving along a parabolic path if v<√gRDit will start moving along a parabolic path if v>√gRIf a particle of mass m start from along curved circular path shown in figure with constant tangential acceleration a, then net force at B on the particle has the magnitude :
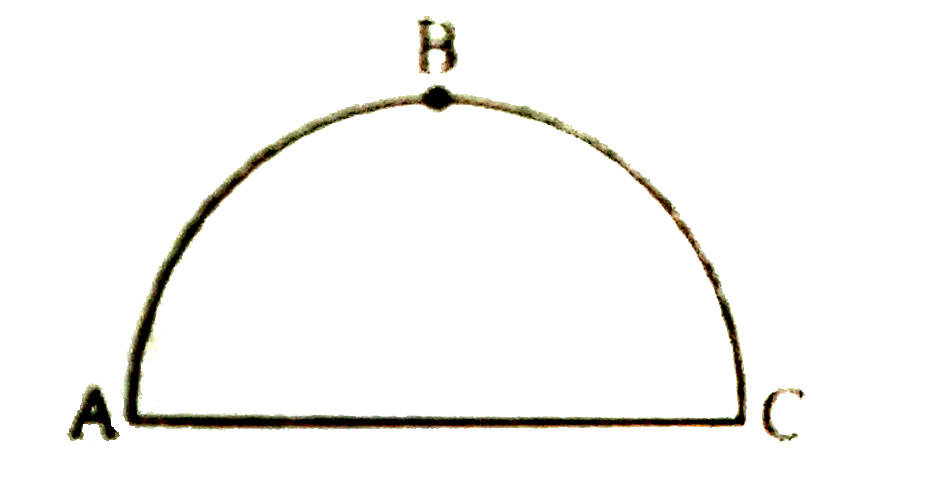 AzeroB2ma√1+π2Cma√1+π2Dmaπ√1+π
AzeroB2ma√1+π2Cma√1+π2Dmaπ√1+π
A particle mass m begins to slide down a fixed smooth sphere from the top of its vertical diameter. Calculate its tangential acceleration, radial acceleration and total acceleration when it breaks off.
View SolutionA particle of mass m slides down from the vertex of semi- hemisphere, without any initial velocity. At what height from horizontal will the particle leave the sphere-
View SolutionA uniform ball of radius r rolls without slipping down from the top of a sphere of radius R Find the angular velocity of the ball at the moment it breaks off the sphere. The initial velocity of the ball is negligible.
View SolutionA small body of mass m=0.30kg starts sliding down from the top of a smooth sphere of radius R=1.00m. The sphere rotates with a constant angular velocity ω=6.0rad/s about a vertical axis passing through its centre. Find the centrifugal force of inertia and the Coriolis force at the moment when the body breaks off the surface of the sphere in the reference frame fixed to the sphere.
View SolutionA particle is projected with velocity u horizontally from the top of a smooth sphere of radius a so that it slides down the outside of the spher. If the particle leaves the outsides down th eoutside of the sphere. If the particle leaves the sphere when it has fallen a height a4 , the value of u is
View Solution
