A particle of mass m1=4kg moving at 6ˆims−1 collides perfectly elastically with a particle of mass m2=2 moving at 3ˆims−1
Velocity of centre of mass (CM) is 5ˆims−1
The velocities of the particles relative to the centre of mass have same magnitude.
Speed of individual particle before and after collision remains same.
The velocity of particles relative to CM after collision are →v1f/cm=−ˆims−1,→v2f/cm=2ˆims−1
The correct Answer is:A, D
To solve the problem step by step, we will analyze the given information about the two particles, apply the relevant formulas, and check each statement provided.
Given:
- Mass of particle 1, m1=4kg
- Initial velocity of particle 1, →v1=6^im/s
- Mass of particle 2, m2=2kg
- Initial velocity of particle 2, →v2=3^im/s
Step 1: Calculate the velocity of the center of mass (COM)
The velocity of the center of mass (→Vcm) is given by the formula:
→Vcm=m1→v1+m2→v2m1+m2
Substituting the values:
→Vcm=(4kg⋅6^im/s)+(2kg⋅3^im/s)4kg+2kg
Calculating the numerator:
=24^i+6^i6kg=30^i6=5^im/s
Step 2: Check Statement A
Statement A claims that the velocity of the center of mass is 5^im/s. From our calculation, we found that this is true.
Step 3: Calculate the relative velocities of the particles with respect to the center of mass
The relative velocity of particle 1 with respect to the center of mass is:
→v1,cm=→v1−→Vcm=6^i−5^i=1^im/s
The relative velocity of particle 2 with respect to the center of mass is:
→v2,cm=→v2−→Vcm=3^i−5^i=−2^im/s
Step 4: Check Statement B
Statement B claims that the velocities of the particles relative to the center of mass have the same magnitude. We found that:
- Magnitude of →v1,cm=1m/s
- Magnitude of →v2,cm=2m/s
Since the magnitudes are not the same, Statement B is false.
Step 5: Calculate final velocities after the elastic collision
Using the formulas for perfectly elastic collisions, we can find the final velocities:
For particle 1:
→v′1=m1−m2m1+m2→v1+2m2m1+m2→v2
Substituting the values:
→v′1=4−24+2⋅6^i+2⋅26⋅3^i
Calculating:
=26⋅6^i+46⋅3^i=2^i+2^i=4^im/s
For particle 2:
→v′2=2m1m1+m2→v1+m2−m1m1+m2→v2
Substituting the values:
→v′2=2⋅46⋅6^i+2−46⋅3^i
Calculating:
=86⋅6^i−26⋅3^i=8^i−1^i=7^im/s
Step 6: Check Statement C
Statement C claims that the speeds of individual particles before and after the collision remain the same. We found:
- Speed of particle 1 before = 6 m/s, after = 4 m/s
- Speed of particle 2 before = 3 m/s, after = 7 m/s
Since the speeds are not the same, Statement C is false.
Step 7: Check Statement D
For Statement D, we need to find the relative velocities after the collision:
Relative velocity of particle 1 with respect to the center of mass after collision:
→v1,cm′=→v′1−→Vcm=4^i−5^i=−1^im/s
Relative velocity of particle 2 with respect to the center of mass after collision:
→v2,cm′=→v′2−→Vcm=7^i−5^i=2^im/s
Thus, Statement D is true.
Final Conclusion
- Statement A: True
- Statement B: False
- Statement C: False
- Statement D: True
- Mass of particle 1, m1=4kg
- Initial velocity of particle 1, →v1=6^im/s
- Mass of particle 2, m2=2kg
- Initial velocity of particle 2, →v2=3^im/s
- Magnitude of →v2,cm=2m/s
- Speed of particle 2 before = 3 m/s, after = 7 m/s
- Statement B: False
- Statement C: False
- Statement D: True
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Linked Comprehension
105 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Single Correct
141 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A partical of mass m moving with velocity 1m/s collides perfectly elastically with another particle of mass 2m. If the incident particle is deflected by 90∘. The heavy mass will make and angle θ with the initial direction of m equal to:
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 3 m at rest. Loss of KE of system in the collision is
View Solution
Knowledge Check
A particle of mass 1 g moving with a velocity →v1=3ˆi−2ˆjms−1 experiences a perfectly in elastic collision with another particle of mass 2 g and velocity →v2=4ˆj−6ˆkms−1. The velocity of the particle is
A2.3ms−1B4.6ms−1C9.2ms−1D6ms−1
A particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass 2 kg moving with a speed of 6 m/s collides elastically with another particle of mass 4 kg travelling in same direction with a speed of 2m/s. The maximum possible deflection of the 2 kg particle is
View SolutionA particle of mass m and momentum p moves on a smooth horizontal table and collides directly and elastically with a similar particle (of mass m) having momentum −2p. The loss (−) or gain (+) in the kinetic energy of the first particle in the collision is
View SolutionA particle of mass m moving with speed V collides elastically with another particle of mass 2m. Find speed of smaller mass after head on collision
View SolutionA particle of mass m is moving along the x-axis with initial velocity ui . It collides elastically with a particle of mass 10 m at rest and then moves with half its initial kinetic energy (see figure).If sinθ1=√nsinθ2 then value of n is
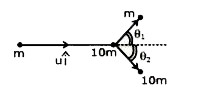
View SolutionA particle of mass m moving with speed v in position x - direction collides perfectly inelastically with another identical particle moving with same speed in positive y - direction . Find final velocity of the combination.
View Solution
