A particle with a velcoity (u) so that its horizontal ange is twice the greatest height attained. Find the horizontal range of it.

A

$\frac{4 v^{2}}{5 g}$

B

$\frac{v^{2}}{g}$

C

$\frac{v^{2}}{2 g}$

D

$\frac{2 v^{2}}{3 g}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Given, a particle having horizontal range is twice the greatest height attained by it, i.e.

$R = 2 H$
$\frac{v^{2} sin θ}{g} = \frac{2 v^{2} {sin}^{2} θ}{2 g}$
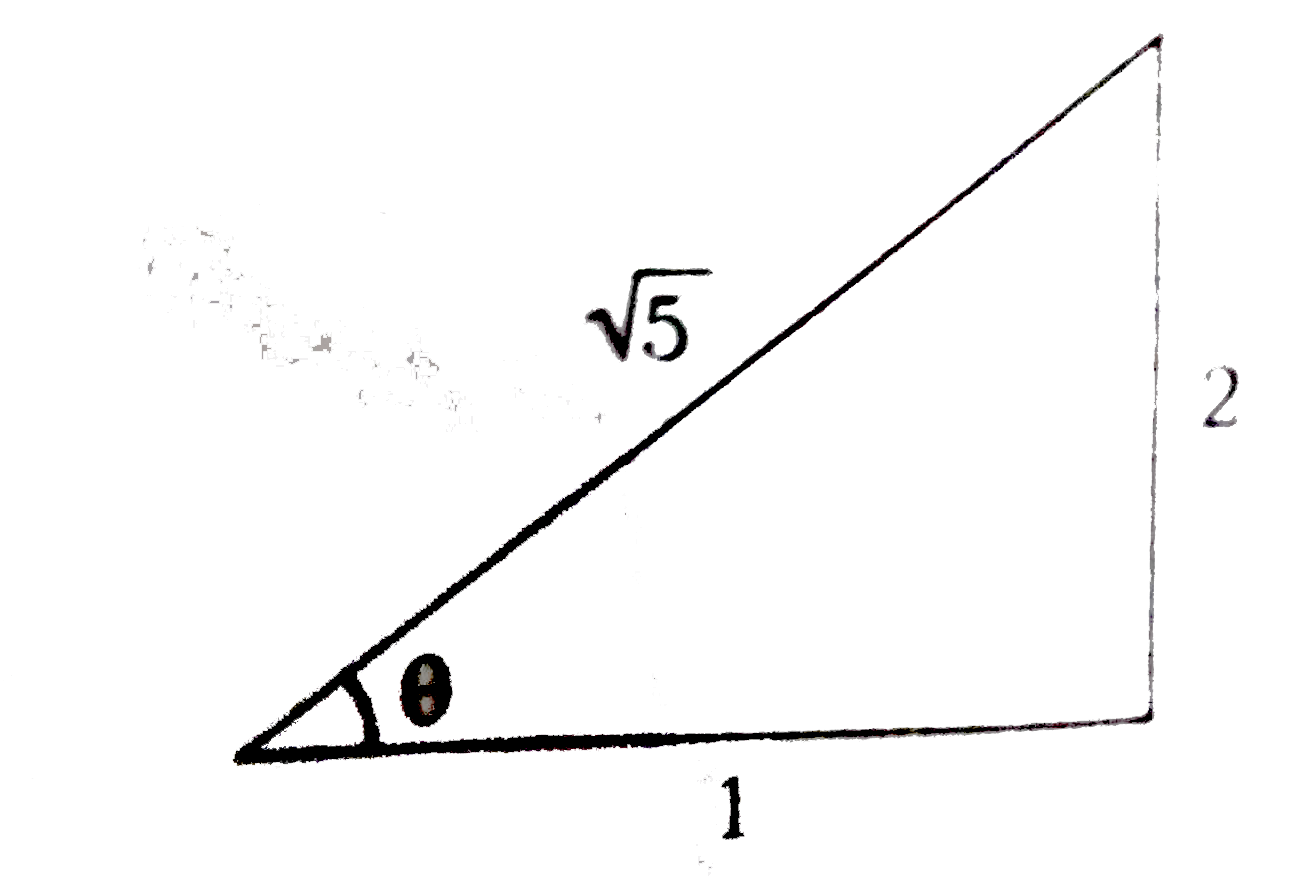
$\Rightarrow 2 sin θ cos θ = {sin}^{2} θ \Rightarrow tan θ = 2$
$\Rightarrow sin θ = \frac{2}{\sqrt{5}} \Rightarrow cos θ = \frac{1}{\sqrt{5}}$
$∴ R = \frac{v^{2} sin 2 θ}{g} = \frac{v^{2} \times 2 sin θ cos θ}{g}$
$\Rightarrow R = v^{2} \times \frac{2}{g} \times \frac{2}{\sqrt{5}} \times \frac{1}{\sqrt{5}}$
i.e., $R = \frac{4 v^{2}}{5 g}$

|

Updated on:21/07/2023

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A body is projected at such an angle that the horizontal range is three times the greatest height. The-angle of projection is
A $25^{\circ} 8'$
B $33^{\circ} 7'$
C $42^{\circ} 8'$
D $53^{\circ} 8'$

DC PANDEY ENGLISH-MOTION-Medical entrances gallery

Home
Ask Doubt
Profile