A physical pendulum performs small oscillations about the horizontal axis with frequency ω1=15.0s−1. When a small body of mass m=50g is fixed to the pendulum at a distance l=20cm below the axis, the oscillation frequency becomes equal to ω2=10.0s−1. Find the moment of inertia of the pendulum relative to the oscillation axis.
Let, moment of inertia of the pendulum, about the axis, concerned is I, then writing Nz=Iβz, for th ependulum
−mgxsinaθ=I.θor .θ=−mgxIθ ( For small θ)
which is the required equation for S.H.M. So, the frequency of oscillation,
ω1=√MgxI or x=IMg√ω21...(1)
Now, when the mass m is attached to the pendulum, at a distance l below the oscillating axis,
−Mgxsinθ′−mglsinθ′=(I+mg2)d2θ′dt2
or −g(Mx+ml)(I+ml2)θ′=d2θdt2, (For small θ)
which is again the equation of S.H.M.,So, the new frequency,
ω2=√g(Mx+ml)(I+ml2)...(2)
Solving Eqns (1) and (2)
ω2=
⎷g((I/g)ω21+ml)(I+ml2)
or ω22=Iω21+mglI+ml2
or, I(ω22−ω21)=mgl−mω22l2
and hence, I=ml2(ω22−g/l)/(ω21−ω22)=0.8g.m2
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations
56 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
Two physical pendulums perform small oscillations about the same horizontal axis with frequencies ω1 and ω2 . Their moments of inertia relative to the given axis are equal to I1 and I2 respectively. In a state of stable equilibium the pendulums were fastened rigifly together. What will be the frequency of small oscillations of the compound pendulum ?
View SolutionA body of mass 0.2 kg oscillates about a horizental axis at a distance 20 cm from its centre ofr gravity. Ifd the length of the equivalent simple pendulem be 0.35 m, find the moment of inertia about the axis of suspension.
View Solution
Knowledge Check
When a mass m is connected individually to two springs S1 and S2, the oscillation frequencies are v1 and v2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
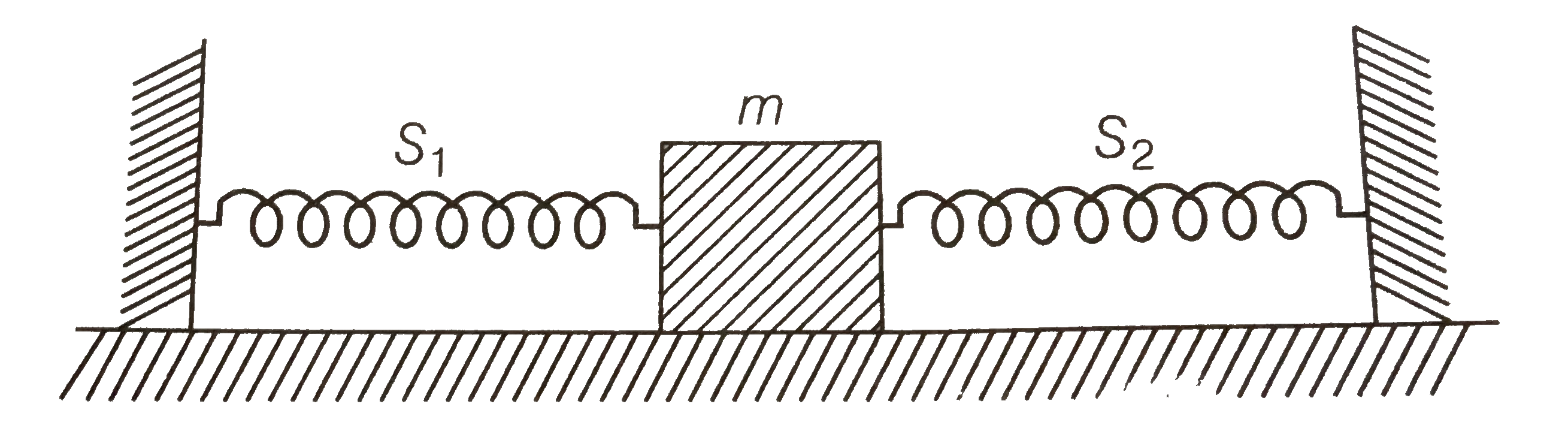 Av1+v2B√v21+v22C(1v1+1v2)−1D√v21−v22
Av1+v2B√v21+v22C(1v1+1v2)−1D√v21−v22
A body of mass 200g oscillates about a horizontal axis at a distance of 20cm from its centre of gravity . If the length of the equivalent simple pendulum is 35cm, find its moment of inertia about the point of suspension.
View SolutionA horizontal disc rotating about a vertical axis passing through its centre makes 180 rpm. A small piece of wax of mass 10 g falls vertically on the disc and adheres to it at a distance of 8 cm from its axis. If the frequency is thus reduced to 150 rpm, calculate the moment of inertia of the disc.
View SolutionA magnetic needle of dipole moment 0.067JT−1 oscillates with a period of 2/3 s in a uniform magnetic field of 100 G . Find the moment of inertia of the needle about the axis of oscillations.
View SolutionA particle performs harmonic oscillations along the x axis about the equilibrium position x=0. The oscillation frequency is ω=4.00s−1. At a certain moment of time the particle has a coordinatex0=25.0cm and its velocity is equal to vx0=100cm/s.
Find the coordinate x and the velocity vx of the particle t=2.40s after that moment.
View SolutionA uniform rod of length l performs small oscillations about the horizontal axis OO′ perpendicular to the rod and passing through one of its points. Find the distance the centre of inertia of the rod and the axis OO′ at which the oscillation period is the shortest. What is it equal to ?
View SolutionThe forced harmonic oscillations have equal displacement amplitude at frequencies ω1=400s−1 and ω2=600s−1. Find the resonance frequency at which the displacement amplitude is maximum.
View SolutionA smooth horizontal disc rotates about the vertical axis O (figure) with a constant angular velocity ω. A thin uniform rod AB of length l performs small oscillation about the vertical axis A fixed to the disc at a distance a from the axis of the disc. Find the frequency ω0 of these oscillations.

View Solution
