A point mass starts moving in straight line with constant acceleration a from rest at t=0. At time t=2s, the acceleration changes the sign remaining the same in magnitude. The mass returns to the initial position at time t=t0 after start of motion. Here t0 is
4s
(4+2√2)s
(2+2√2)s
(4+4√2)s
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of the point mass under the given conditions.
Step 1: Understand the motion of the mass
The mass starts from rest and accelerates with a constant acceleration a for the first 2 seconds. After 2 seconds, the acceleration changes direction (becomes −a), meaning the mass will decelerate.
Step 2: Calculate the displacement during the first 2 seconds
Using the equation of motion, the displacement s during the first 2 seconds can be calculated as:
s=ut+12at2
Since the initial velocity u=0 (the mass starts from rest), we have:
s=0+12a(2)2=2a
Step 3: Determine the velocity at t=2 seconds
The velocity v at t=2 seconds can be calculated using:
v=u+at
Again, since u=0:
v=0+a⋅2=2a
Step 4: Analyze the motion after t=2 seconds
After 2 seconds, the mass starts decelerating with an acceleration of −a. Let t1 be the time taken to return to the initial position after t=2 seconds.
Step 5: Write the equation for displacement after t=2 seconds
The displacement during the time t1 can be expressed as:
Displacement=Initial Displacement+Displacement during t1
Since the mass returns to the initial position, the total displacement must equal zero:
−2a=(2a)t1−12at21
This simplifies to:
−2a=2at1−12at21
Step 6: Simplify the equation
Dividing through by a (assuming a≠0):
−2=2t1−12t21
Rearranging gives:
12t21−2t1−2=0
Multiplying through by 2 to eliminate the fraction:
t21−4t1−4=0
Step 7: Solve the quadratic equation
Using the quadratic formula t=−b±√b2−4ac2a:
t1=4±√(−4)2−4⋅1⋅(−4)2⋅1=4±√16+162=4±√322=4±4√22=2±2√2
Since time cannot be negative, we take:
t1=2+2√2
Step 8: Calculate the total time t0
The total time t0 from the start until the mass returns to the initial position is:
t0=2+t1=2+(2+2√2)=4+2√2
Final Answer
Thus, the total time t0 is:
4+2√2
The mass starts from rest and accelerates with a constant acceleration a for the first 2 seconds. After 2 seconds, the acceleration changes direction (becomes −a), meaning the mass will decelerate.
Using the equation of motion, the displacement s during the first 2 seconds can be calculated as:
s=ut+12at2
Since the initial velocity u=0 (the mass starts from rest), we have:
s=0+12a(2)2=2a
The velocity v at t=2 seconds can be calculated using:
v=u+at
Again, since u=0:
v=0+a⋅2=2a
After 2 seconds, the mass starts decelerating with an acceleration of −a. Let t1 be the time taken to return to the initial position after t=2 seconds.
The displacement during the time t1 can be expressed as:
Displacement=Initial Displacement+Displacement during t1
Since the mass returns to the initial position, the total displacement must equal zero:
−2a=(2a)t1−12at21
This simplifies to:
−2a=2at1−12at21
Dividing through by a (assuming a≠0):
−2=2t1−12t21
Rearranging gives:
12t21−2t1−2=0
Multiplying through by 2 to eliminate the fraction:
t21−4t1−4=0
Using the quadratic formula t=−b±√b2−4ac2a:
t1=4±√(−4)2−4⋅1⋅(−4)2⋅1=4±√16+162=4±√322=4±4√22=2±2√2
Since time cannot be negative, we take:
t1=2+2√2
The total time t0 from the start until the mass returns to the initial position is:
t0=2+t1=2+(2+2√2)=4+2√2
Thus, the total time t0 is:
4+2√2
Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - MCQ_TYPE
27 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE
19 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
A point mass starts moving in a straight line with constant acceleration. After time t0 the acceleration changes its sign, remaining the same in magnitude. Determine the time T from the beginning of motion in which the point mass returns to the initial position.
View SolutionA particle, initially at rest, starts moving in a straight line with an acceleration a=6t+4m/s2. The distance covered by it in 3 s is
View SolutionA particle, starting from rest, moves in a straight line with constant acceleration a0 for a time interval t0.Then is accelerates at a constant rate 2a0 for time interval 2t0 .The distance travelled by the particle in time 3t0 from the starts is S . Then Sa0t20=
View SolutionA particle starts from rest at t=0 and moves in a straight line with an acceleration as shown below. The velocity of the particle at t=3 s is

View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionA particle starts moving from rest on a straight line. Its acceleration a verses time t is shown in the figure. The speed of the particle is maximum at the instant
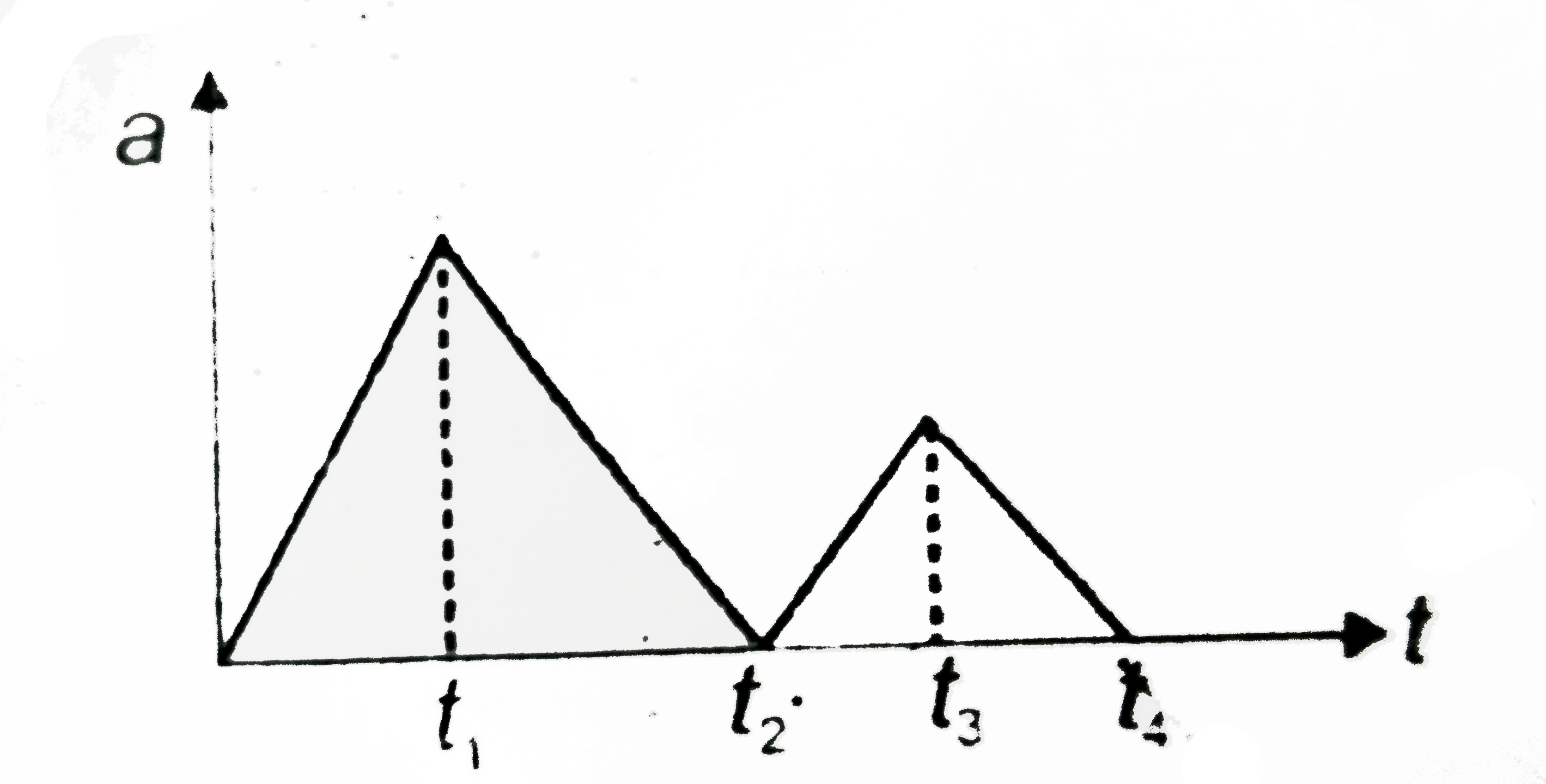
View SolutionA particle is moving in a straight line whose acceleration versus time graph is given. Assume that initial velocity is in the direction of acceleration. Then
which of the statement is correct between time t=0 to t=t0.
 .
.
View SolutionA particle starts moving from position of rest under a constant acceleration. If it travels a distance x in t second, what distance will it travel in next t second?
View SolutionA particle starts from rest at time t=0 and moves on a straight line with acceleration as plotted in fig. The speed of the particle will be maximum after time.

View SolutionThe figure shows the v−t graph of a particle moving in a straight line. Starting from rest, the time after which the particle returns to its starting position is
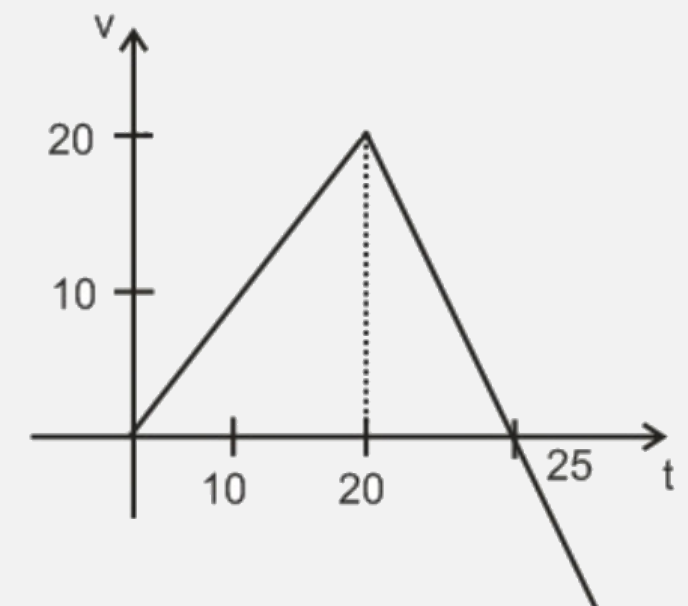
View Solution