A pulse is propagating on a long stretched string along its length taken as positive x-axis. Shape of the string at t = 0 is given by y=√a2−x2when |x|≤a=0 when |x|≥a . What is the general equation of pulse after some time 't' , if it is travelling along positive x-direction with speed V?
y(x,t)=√a2−(x+Vt)2 when |x+Vt|≤a=x+Vt when |x+Vt|≥a
y(x,t)=√a2+(x−Vt)2 when |x−Vt|≤a=a when |x+Vt|≥0
y(x,t)=√a2−(x−Vt)2 when |x−Vt|≤a=0 when |x−Vt|≥a
y(x,t)=√a2+(x+Vt)2 when |x+Vt|≤a=a when (x+Vt)≥a
The correct Answer is:C
To find the general equation of the pulse after some time t, we start with the initial shape of the pulse given by:
y=√a2−x2for |x|≤a
y=0for |x|>a
This shape represents a semicircle with radius a centered at the origin.
Step 1: Understand the propagation of the pulse
The pulse is traveling in the positive x-direction with speed V. The general form of a wave traveling in the positive x-direction can be expressed as:
y(x,t)=f(x−Vt)
where f is the shape of the wave at t=0.
Step 2: Substitute the initial shape into the wave equation
From the problem, we know that at t=0:
f(x)=√a2−x2for |x|≤a
f(x)=0for |x|>a
Thus, substituting x−Vt into f:
y(x,t)=√a2−(x−Vt)2for |x−Vt|≤a
y(x,t)=0for |x−Vt|>a
Step 3: Define the conditions for the pulse
The conditions for the pulse to exist can be expressed as:
|x−Vt|≤a
This means:
−a≤x−Vt≤a
Step 4: Rewrite the conditions
Rearranging the inequalities gives:
Vt−a≤x≤Vt+a
Final Equation
Thus, the general equation of the pulse after some time t is:
y(x,t)=√a2−(x−Vt)2for Vt−a≤x≤Vt+a
y(x,t)=0for |x−Vt|>a
y=0for |x|>a
The pulse is traveling in the positive x-direction with speed V. The general form of a wave traveling in the positive x-direction can be expressed as:
From the problem, we know that at t=0:
f(x)=0for |x|>a
y(x,t)=0for |x−Vt|>a
The conditions for the pulse to exist can be expressed as:
Rearranging the inequalities gives:
Thus, the general equation of the pulse after some time t is:
y(x,t)=0for |x−Vt|>a
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - LECTURE SHEET (EXERCISE-I (LEVEL-II(ADVANCED)MORE THAN ONE CORRECT ANSWER TYPE QUESTIONS))4videos
WAVE MOTION
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - LECTURE SHEET (EXERCISE-I (LEVEL-II(ADVANCED)LINKED COMPREHENSION TYPE QUESTIONS))3videos
WAVE MOTION
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SHEET ADVANCED (INTEGER TYPE QUESTIONS)10videos
WAVE MOTION
- BOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -366videos
UNITS AND MEASUREMENTS
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)97videos
WAVE MOTION AND SOUND
Similar Questions
the equation of a wave travelling along the positive x - axis ,as shown in figure at t = 0 is given by
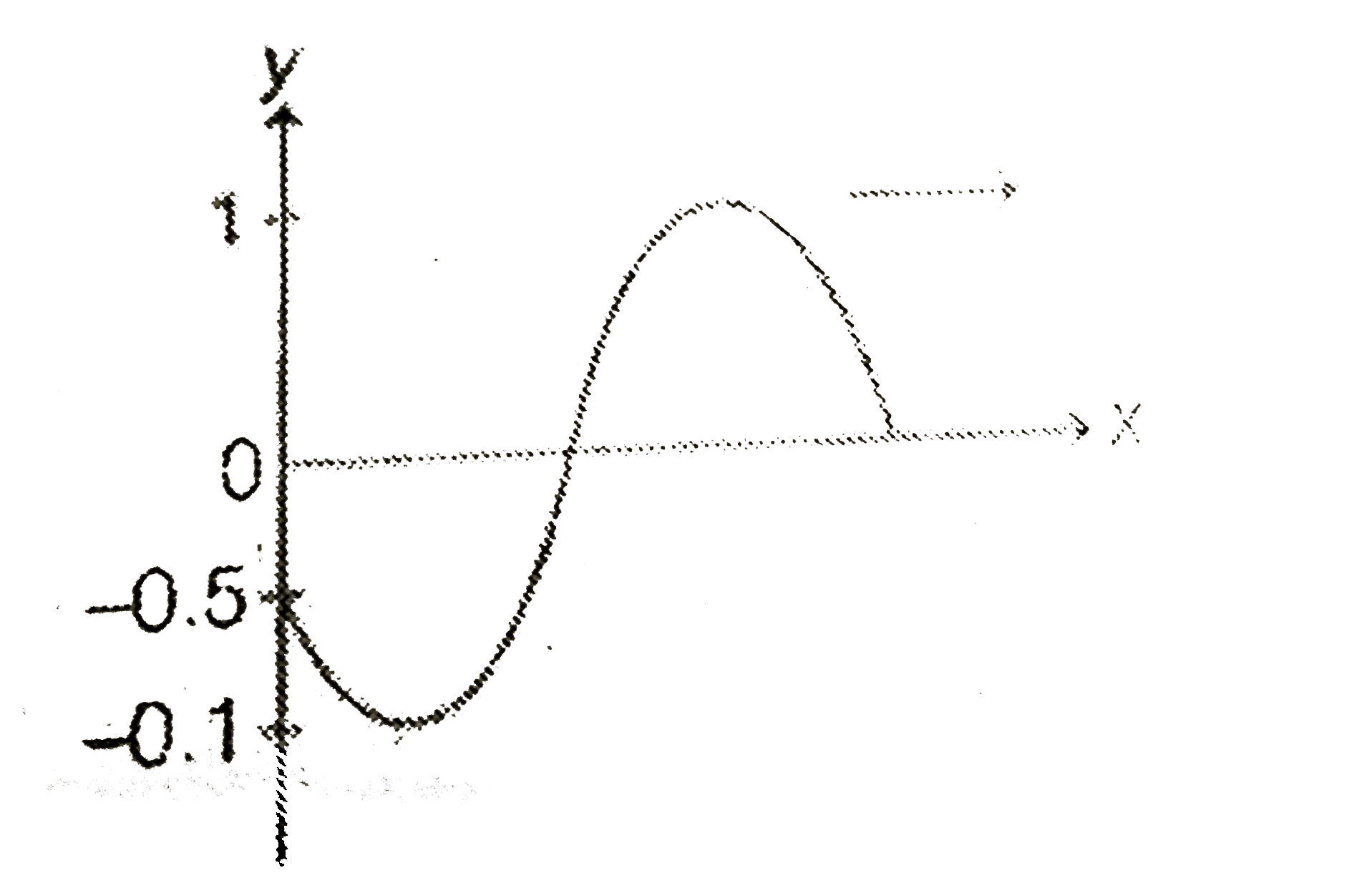 View Solution
View SolutionThe equation of a wave travelling along the positive x-axis, as shown in figure at t = 0 is given by
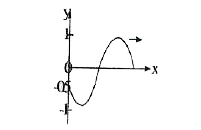 View Solution
View SolutionA wave pulse is travelling on a string with a speed v towards the positive X-axis. The shape of the string at t = 0 is given by g(x)=Asin(xa), where A and a are constants. (a) What are the dimensions of A and a ? (b) Write the equation of the wave for a general time 1, if the wave speed is v.
View SolutionA wave is propagating on a long stretched string along its length taken as the positive x-axis. The wave equation is given as
y=y0e−(tT−xλ)2
where y0=4mm,T=1.0sandλ=4cm.(a) find the velocity of wave. (b) find the function f(t) giving the displacement of particle at x=0. (c) find the function g(x) giving the shape of the string at t=0.(d) plot the shape g(x) of the string at t=0 (e) Plot of the shape of the string at t=5s.View SolutionIf at t=0, a travelling wave pulse in a string is described by the function,
y=10(x2+2)
Hence, xandy are in meter and t in second. What will be the wave function representing the pulse at time t, if the pulse is propagating along positive x-axix with speed 2m/s?View SolutionA wave propagates in a string in the positive x-direction with velocity v. The shape of the string at t=t0 is given by
f(x,t0)=Asin(x2a2). Then the wave equation at any instant t is given byView SolutionThe position x of a particle with respect to time t along the x-axis is given by x=9t2−t3 where x is in meter and t in second. What will be the position of this particle when it achieves maximum speed along the positive x direction
View SolutionAt t=0, transverse pulse in a wire is described by the function
y=6x2+3
where x and y are in metres. Write the function y(x,t) that describe this plus if it is travelling in the positive x-direction with a speed of 4.50m/s.View SolutionA travelling wave on a long stretched string along the positIve x-axis is given by y=5mme(t5s−x5cm)2. Using this equation answer the following questions.
At t = 0, x = 0, the displacement of the wave isView SolutionA wave is represented by the equation y=0.5sin(10t−x)m. It is a travelling wave propagating along the + x direction with velocity
View Solution
