A radioactive substance emits n beat particles in the first 2 s and 0.5 n beta particles in the next 2 s. The mean life of the sample is
4 s
2 s
2(1n2)
2 (1n 2) s
The correct Answer is:C
To find the mean life of the radioactive substance, we can follow these steps:
Step 1: Understand the emission of beta particles
In the first 2 seconds, the substance emits n beta particles. In the next 2 seconds, it emits 0.5n beta particles.
Step 2: Determine the remaining particles
After the first 2 seconds, the number of remaining beta particles is:
N=n−n=0
After the next 2 seconds, the number of remaining particles is:
N=0−0.5n=−0.5n
However, since the number of particles cannot be negative, we can interpret this as the substance has decayed significantly.
Step 3: Relate the decay to half-life
The half-life (T1/2) can be determined from the decay process. The first emission of n particles indicates that the substance has undergone a significant decay in the first 2 seconds. The remaining particles after the first 2 seconds can be considered as half of the original amount.
Step 4: Calculate the half-life
From the problem, we can assume that the half-life is the time taken for the number of particles to reduce to half its initial value. Since we see that the number of particles reduces from n to 0.5n in 2 seconds, we can say:
T1/2=2 seconds
Step 5: Relate half-life to mean life
The mean life (τ) is related to half-life by the formula:
τ=T1/2ln2
Substituting the value of T1/2:
τ=2ln2
Step 6: Final answer
Thus, the mean life of the sample is:
τ=2ln2
Conclusion
The mean life of the radioactive substance is 2ln2.
---
In the first 2 seconds, the substance emits n beta particles. In the next 2 seconds, it emits 0.5n beta particles.
After the first 2 seconds, the number of remaining beta particles is:
N=n−n=0
After the next 2 seconds, the number of remaining particles is:
N=0−0.5n=−0.5n
However, since the number of particles cannot be negative, we can interpret this as the substance has decayed significantly.
The half-life (T1/2) can be determined from the decay process. The first emission of n particles indicates that the substance has undergone a significant decay in the first 2 seconds. The remaining particles after the first 2 seconds can be considered as half of the original amount.
From the problem, we can assume that the half-life is the time taken for the number of particles to reduce to half its initial value. Since we see that the number of particles reduces from n to 0.5n in 2 seconds, we can say:
T1/2=2 seconds
The mean life (τ) is related to half-life by the formula:
τ=T1/2ln2
Substituting the value of T1/2:
τ=2ln2
Thus, the mean life of the sample is:
τ=2ln2
The mean life of the radioactive substance is 2ln2.
Topper's Solved these Questions
Similar Questions
A radioactive substance emit 100 beta particfles in the first 2 s and 50 beta particles in the next 2s. The mean life of the sample is
View SolutionA radioactive element decays by β−emission. A detector records n beta particles in 2 s and in next 2 s it records 0.75 n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
View Solution(a) Calculate the activity of one mg sample .28Sr90 whose half life period is 28 years.
(b) An experiment is performed to determine the half-life of a radioactive substance which emits one beta particle for each decay process. Observation shown that an average of 8.4β−particles are emitted each second by 2.5mg of the substance. the atomic weight of the substance is 230. calculate the half0life of the substance.
(c) Determine the quantity of .84Po210 necessary to provide a source of alpha particle of 5mCi strength (T1/2 for Po =138 day).
View SolutionFrom a radioactive substance x numbers of α-particles any y numbers of β-particles are emitted. As a result atomic number decreases by n and mass number by m. Then, match the following two columns.
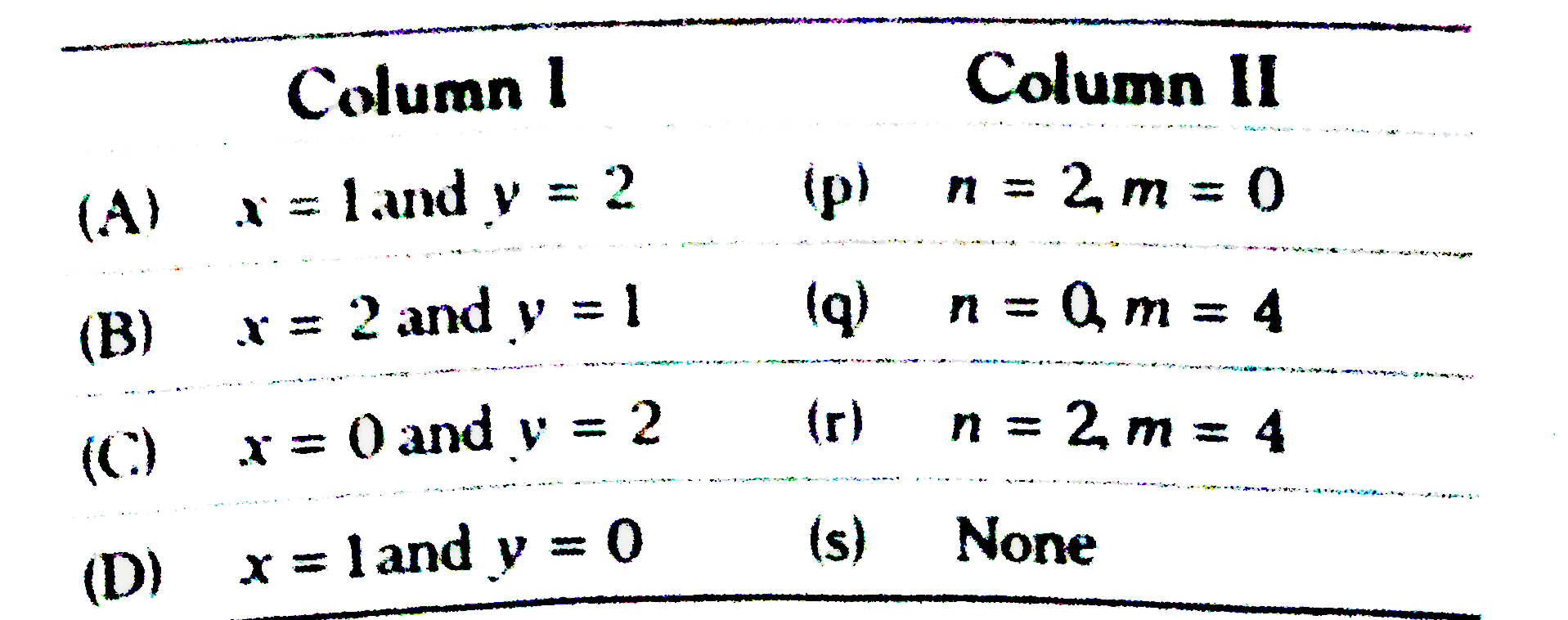
View SolutionFrom a radioactive substance n1 nuclei decay per second at an instant when total number of nuclei are n2. Find half-life of the radioactive substance.
View SolutionA radioactive element decays by β-emission. A detector records n-beta particles in 2 s and in next 2 s it records 0.45 n beta particles. Find mean life.
View SolutionA radioactive element decays by β−emission. A detector racords n-beta particles in 2 sec and in next 2 sec it records 0.65n-beta particles. Find mean life.
View SolutionIn a sample of a radioactive substance, what fraction of the initial nuceli will remain undecayed after a time t=T/2 , where T=half -life of radioactive substance?
View SolutionA nucleus .mnX emits one α−particle and two β−particles. The resulting nucleus is
View SolutionA sample has two isotopes A and B having masses 50g and 30g, respectively. A is radioactive and B is stable.A decays to A' by emitting α-particles. The half-life of A is 2h. Find the mass of the sample after 4hand number of α-particles emitted.
View Solution
