A ray of light is incident on a prism ABC of μ=√3 as shown in Fig. Find the angle of incidence for which the deviation of light by the prism ABC is minimum.
By what angle should the second prism be rotated so that final ray suffers net minimum deviation ?
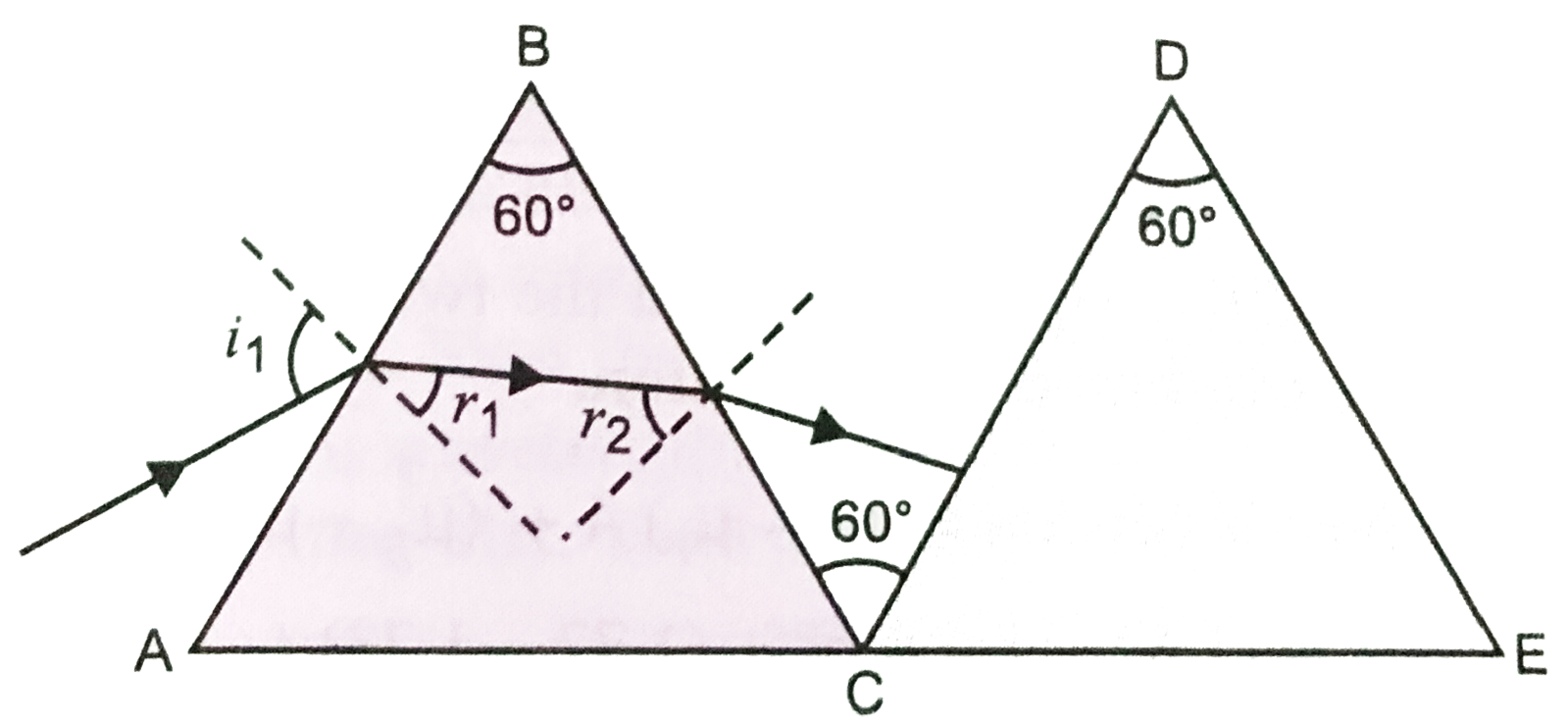
Let i1 be the angle of incidence of ray on face AB of the first prism and r1 corresponding angle of refraction on face AB. If r2 is angle of incidence on the face BC of the prism, then in the minimum deviation position,
r1=r2=A/2=60∘/2=30∘
According to Snell's Law
μ=sini1sinr1
sini1=μsinr1=√3sin30∘=√32
∴i1=60∘
For the final ray from the combination of prism to suffer net minimum deviation, the second prism CDE must be rotated anticlockwise through 60∘ so that opposite faces of the combination become parallel.
Topper's Solved these Questions
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Very short answer question
5 Videos
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - very short answer questions
1 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)
2 Videos
Similar Questions
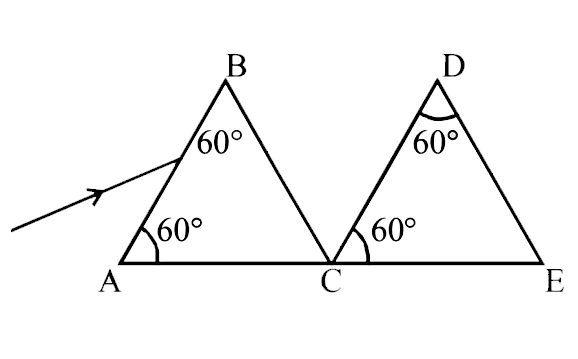
Two identical prism of index √3 are kept as shown in the figure. A light ray strikes the first prism at face AB. Find,
(a) the angle of incidence, so that the emergent ray from the first prism has minimum deviation.
(b) through what angle the prism DCE should be rotated about C so that the final emergent ray also has minimum deviation.
View SolutionA glass prism has a refracting angle of 60∘. The angle of minimum deviation is 40∘. Find the refractive index. At what angle should the ray be incident so as to suffer minimum deviation ?
View Solution
Knowledge Check
A ray of light is incident on a prism as shown in fig. Find the total deviation suffered by the ray if ∠BAC=1∘ and ∠CAD=2∘.
 A1∘B1.5∘C2.5∘D2∘
A1∘B1.5∘C2.5∘D2∘A ray of light is incident on a 60∘ prism at the minimum deviation position. The angle of refraction at the first face (i.e. incident face) of the prism is-
AzeroB30∘C45∘D60∘A ray of light is incident on a 60∘ prism at the minimum deviation position. The angle of refraction at the first face (i.e. incident face) of the prism is-
A30∘B45∘C60∘DZero
A ray of light, incident on an equilateral glass prism (μg=√3) moves parallel to the base line of the prism inside it. Find the angle of incidence for this ray.
View SolutionA ray of light passing through a prism (μ=√3)suffers minimum deviation. It is found that the angle of incidence is double the angle of refraction within the prism. Then, the angle of prism is ________ (in degrees)
View SolutionA ray of light suffers minimum deviation through a prism of refractive index √2. What is the angle of prism if the angle of incidence is double the angle of refraction within the prism?
View SolutionA ray of light is incident normally normallyh on one of the faces of a prism of apex angle 30∘ and refractive index √2. The angle of deviation of the ray is ________________ degrees.
View SolutionA ray of light passing through a prism having refractive index √2 suffers minimum devitation. It is found that the angle of incidence is double the angle of refraction within the prism. Then angle of prism is
View Solution
