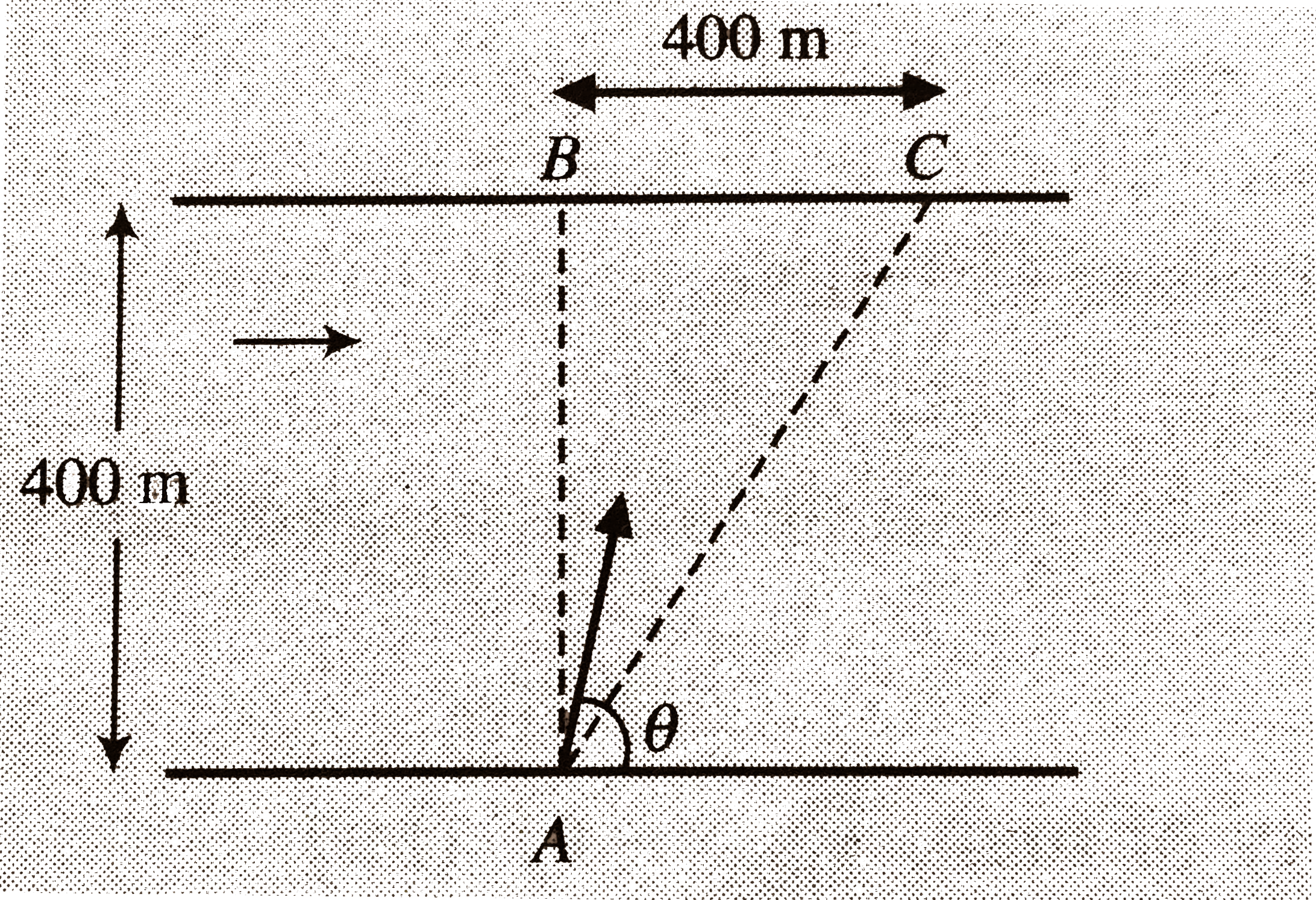
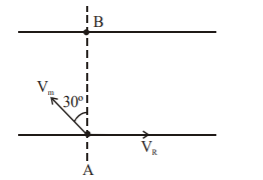
A river is flowing with a speed of 1kmh−1 A swimmer wants to go point C starting from A. He swims with a speed of 5kmh−1 at an angle θ w.r.t. the river flow. If AB=BC=400m, at what angle with the river bank should the swimmer swim ?
 .
.
Resultant path of the swimmer is at 45∘ with bank, therefore, x - and y - components of swimmer's resultant velocity must be equal.
Let velocity of swimmer |→vm|=v
vx=vr+vm,rcosθ
vy=vm,rsinθ
Condition for reaching the point C,
tan45∘=vyvx,vy=vx
(vr+vm,rcosθ)=vm,rsinθ
1+5cosθ=5sinθ
On squaring, 1+25cos2+10cosθ=25−25cos2θ
50cos2θ+10cosθ−24=0
On solving, we get θ=53∘.
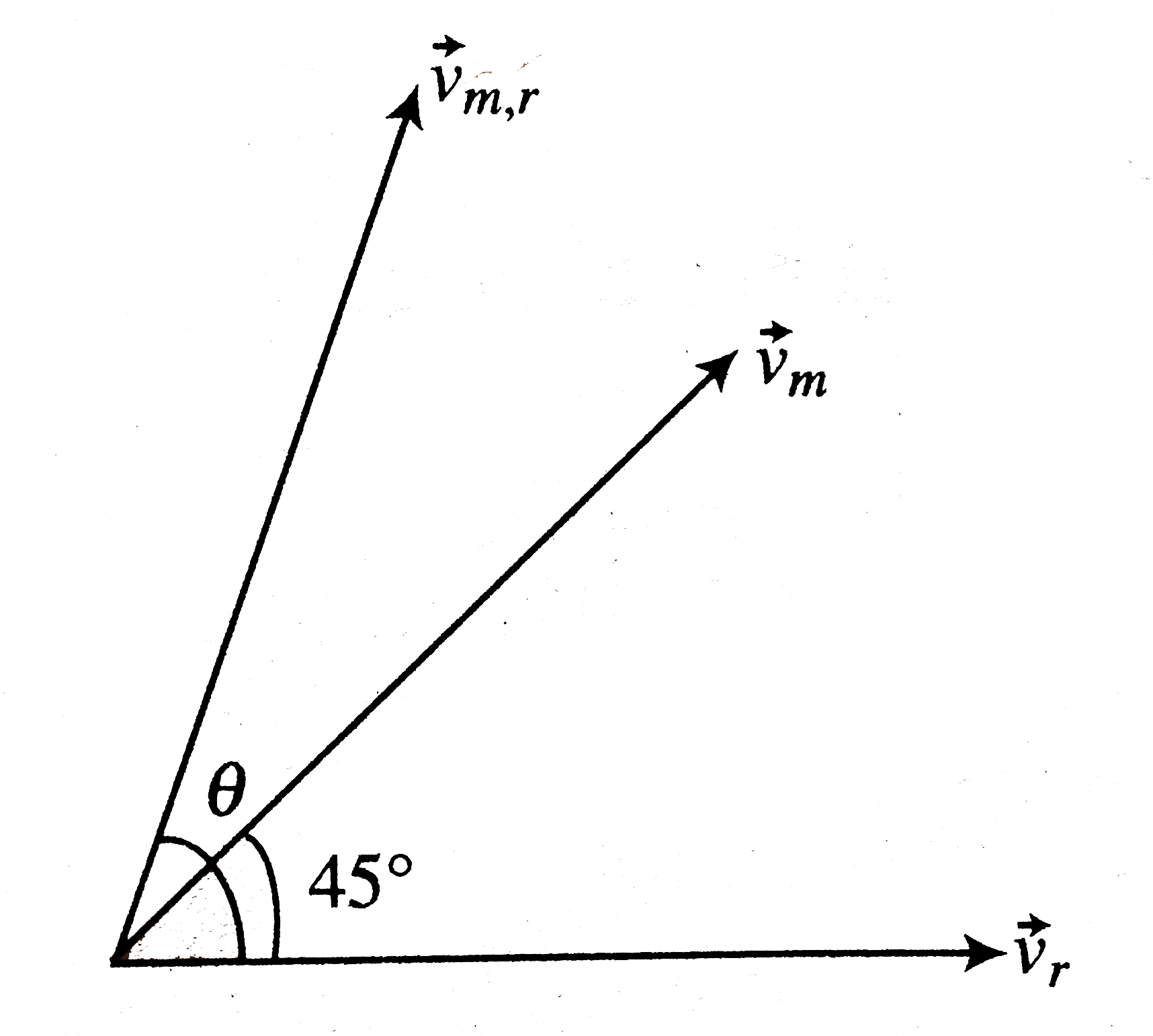 .
.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
A swimmer can swim in still water with a speed of 2.5 m/s. What is the shortest time taken by him to swim across the river?

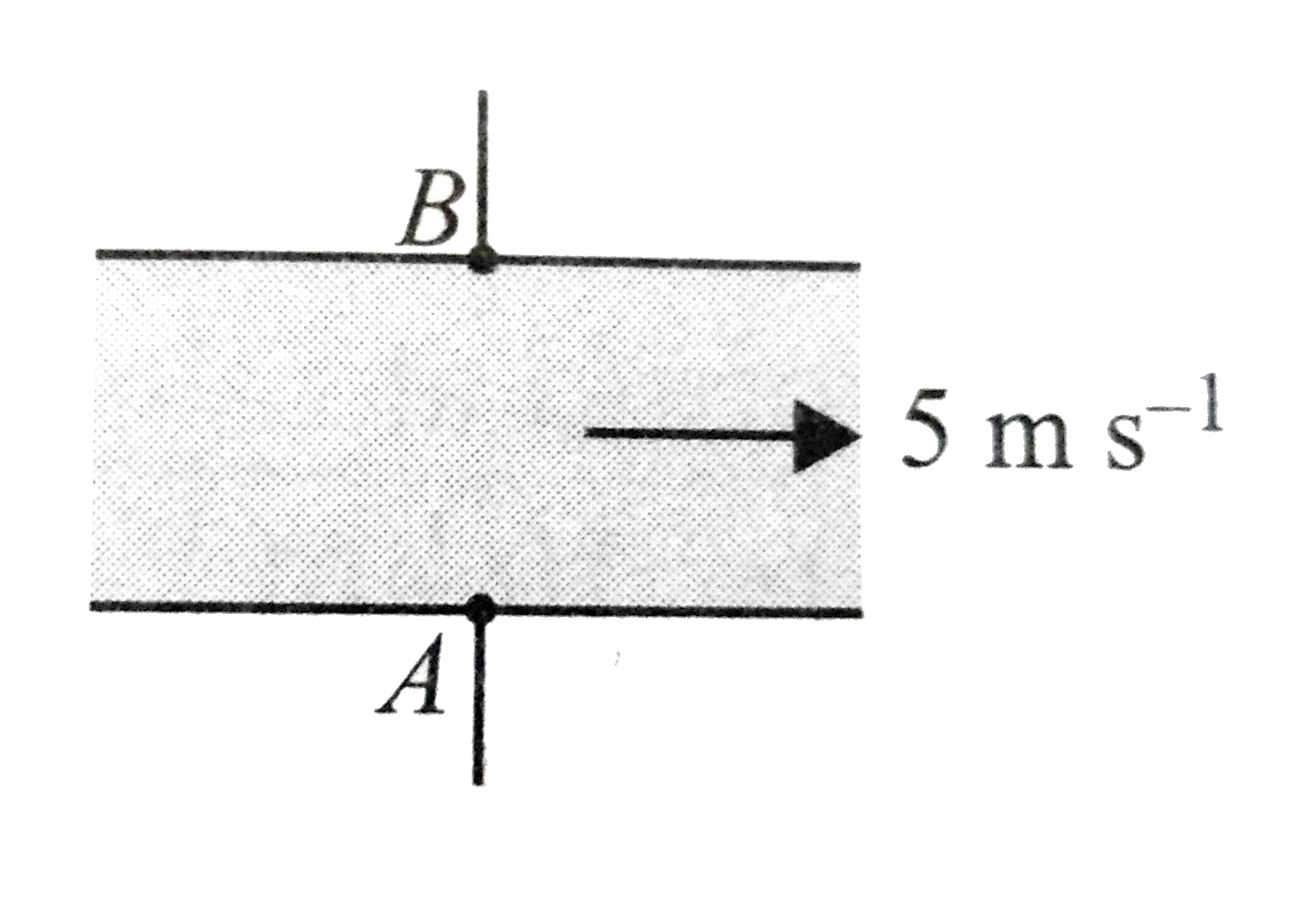
View SolutionA river is flowing from west to east with a speed 5 m s−1. A swimmer can swim in still water at a speed of 10 m s−1 .
If he wants to start from point A on south bank and reach opposite point B on north bank. In what direction direction should he swim ?
View Solution
Knowledge Check
A river is flowing with a speed of 1 km/hr. A swimmer wants to go to point C starting from A. He swims with a speed of 5km/hr. at an angle θ. W.r.t. the river flow. If AB=BC=400m at what angle with river tank shou ld swimmer swim?
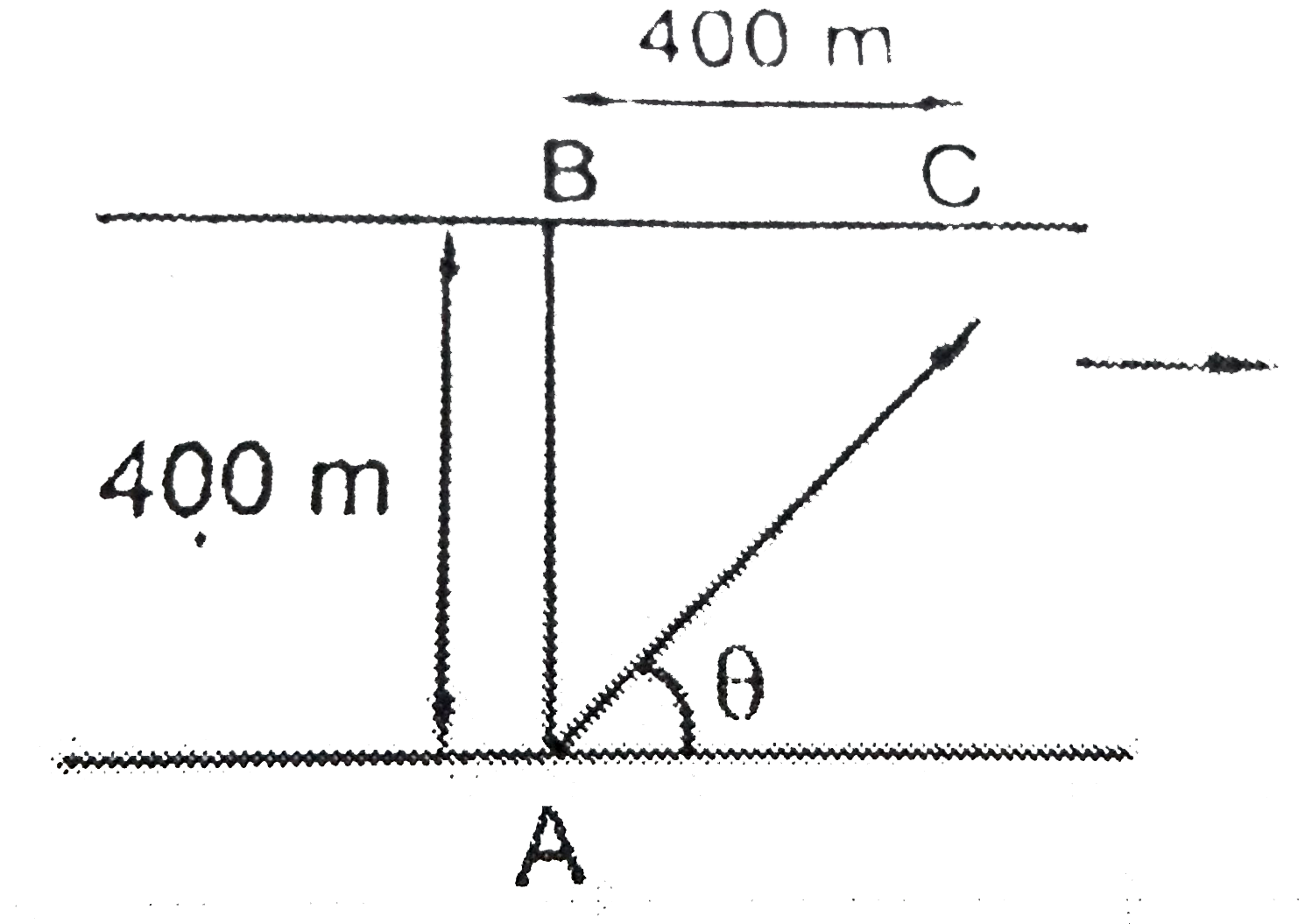 A37∘B53∘C0∘D90∘
A37∘B53∘C0∘D90∘The stream of a river is flowing with a speed of 2 km/h. A swimmer can swim at a speed of 4 km/h. What should be the direction of the swimmer with respect to the flow of the river to cross the river straight?
A90∘B60∘C120∘D150∘A river is flowing due east with a speed 3ms−1. A swimmer can swim in still water at a speed of 4ms−1. If swimmer starts swimming due north, then the resultant velocity of the swimmer is
A3ms−1B5ms−1C7ms−1D2ms−1
A river is flowing steradily with a speed 5kmh−1 . A boat man can row with a speed of 10kmh−1 in still water. He rows his boat in river at right angles to the bank of river, If width of river is 800m, (i) how much time the boat man will take to cross the river ? (ii) How far away from a point just opposite to the bank of river he will be reaching there ? (ii) What will be his effective speed gt
View SolutionA swimmer swims with a speed of 10ms at angle of 1200 from direction of river flow. Find velocity of river flow such that swimmer reach exactly opposite point of bank.
View SolutionA man wishes to cross a river flowing with velocity u swims at an angle θ with the river flow.If the man swims with speed vand if the width of the river is d, then the drift travelled by him is
View SolutionA man can swim at a speed of 3kmh−1 in still water. He wants to cross a 500−m wide river flowing at 2kmh−1. He keeps himself always at an angle to 120∘ with the river flow while swimming.
The time taken to cross the river is.
View SolutionA river is flowing at the rate of 6 km/hr. A swimmer swims across with a velocity of 9 km/hr w.r.t. water. The resultant velocity of the man will be in (km/hr):-
View Solution
