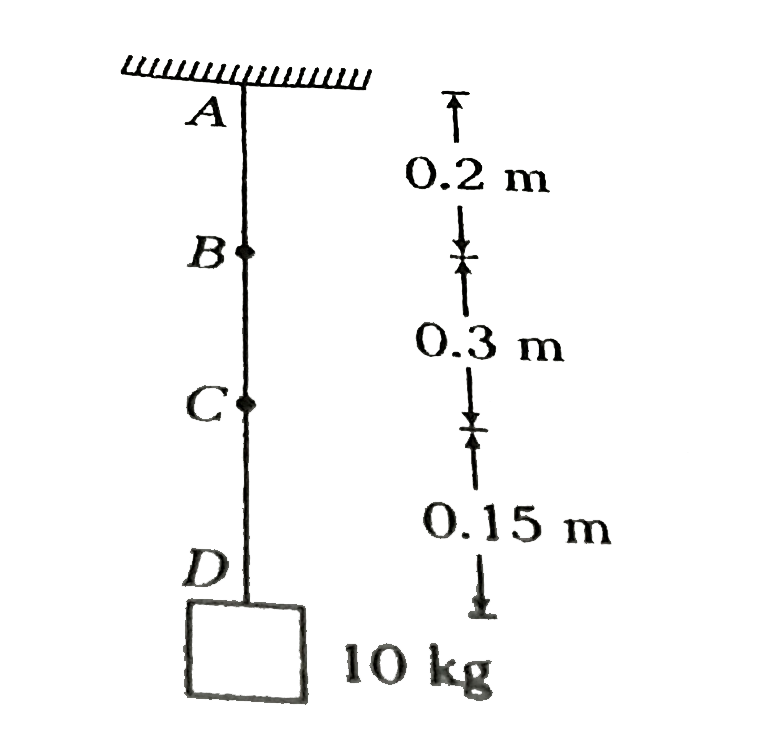
A rod AD consisting of three segments AB,BC and CD joined together is hanging vertically from a fixed support at A. The lengths of the segments are restectively 0.2 m , 0.3m and 0.15 m . The cross-section of the rod is uniformly 10−4m2 . A weight of 10 kg is hung from D.Calculate the displacement of points B,C and D if YAB=3.5×1010Nm−2,YBC=5×1010,YCD=2×1010Nm−2.
(Neglect the weight of the rod )
Given, area , A=10−14m2
YAB=3.5×1010Nm−2
YBC=5×1010Nm−2
YCD=2×1010Nm−2
Increase in length,
ΔL=FLAY=MgLAY=10×10L10−4Y=106LY
Now, increase in length of AB segment
(ΔLAB)=106×LABYAB=106×23.5×1010=5.7×10−6m
Increase in length of BC segment
(ΔL)BC=106×LBCYBC
=106×0.35×1010=6×10−6m
Increase in length of CD segment
(ΔL)CD=106LCDYCD=106×(0.15)×(2×1010)=7.5×10−6m
So, Displacement of B=LAB=5.7×10−6m
Displacement of C=ΔLAB+ΔLBC=11.7×10−6m
Displacement of D=ΔLAB+ΔLBC+ΔLCD=26.7×10−6m
Topper's Solved these Questions
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Solved Examples
6 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - MiscellaneousExamples
4 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
469 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
Similar Questions
Determine the force required to double the length of the steel wire of area of cross-section 5×10−5m2. Give Y for steel =2×1011Nm−2.
View SolutionA rod of uniform cross sectional area 5mm2 weighing5kg and length 1m is suspended vertically from a fixed support the elongation produced in the rod if [Young's modulus of material, Y=2x1011Nm2 and g=10ms−2]
View Solution
Knowledge Check
A light rod of length 2 m is suspended from ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 0.1 cm sq. and the other of brass of cross-section 0.2 cm sq. Along the rod at what distance a weight may be hung to produce equal stresses in the wires?
(YSteel=2×1011Nm−2,YBass=1×1011Nm−2)A43m from steel wireB43m from brass wireC1 m from steel wireD14m from brass wire
_S01_065_Q01.png)
The graph shows the extension of a wire of length 1m suspended from the top of a roof at one end and with a load W connected to the other end. If the cross sectional area of the wire is 1mm2, then the young's modulus of the material of the wire is
(a). 2×1011Nm−2
(b). 2×1010Nm−2
(c). 12×1011Nm−2
(d). none of these
View SolutionA light rod of length L=2m is suspended horizontally from the ceiling by two wires A and B of equal lengths. The wire A is made of steel with the area of cross section AS=1×10−5m2, while the wire B is made of brass of cross sectional area Ab=2×10−5m2. A weight W is suspended at a distance x from the wire A as shown in figure.
Take, Young's modulus of steel and brass as Ys=2×1011Nm−2 and Yb=1×1011Nm−2.
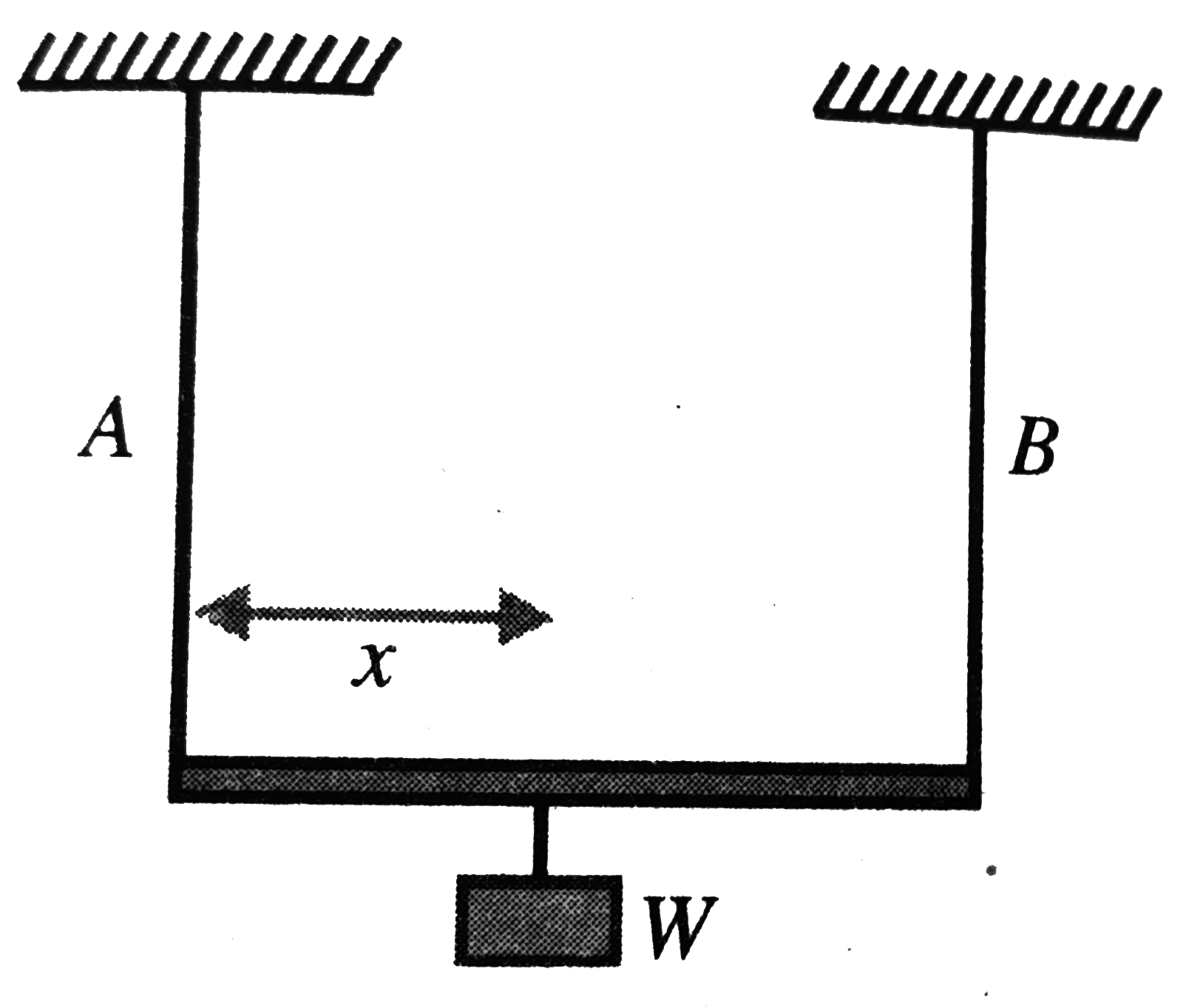
Determine the value of x so that equal stresses are produced in each wire.
View SolutionA light rod of length L=2m is suspended horizontally from the ceiling by two wires A and B of equal lengths. The wire A is made of steel with the area of cross section AS=1×10−5m2, while the wire B is made of brass of cross sectional area Ab=2×10−5m2. A weight W is suspended at a distance x from the wire A as shown in figure.
Take, Young's modulus of steel and brass as Ys=2×1011Nm−2 and Yb=1×1011Nm−2.
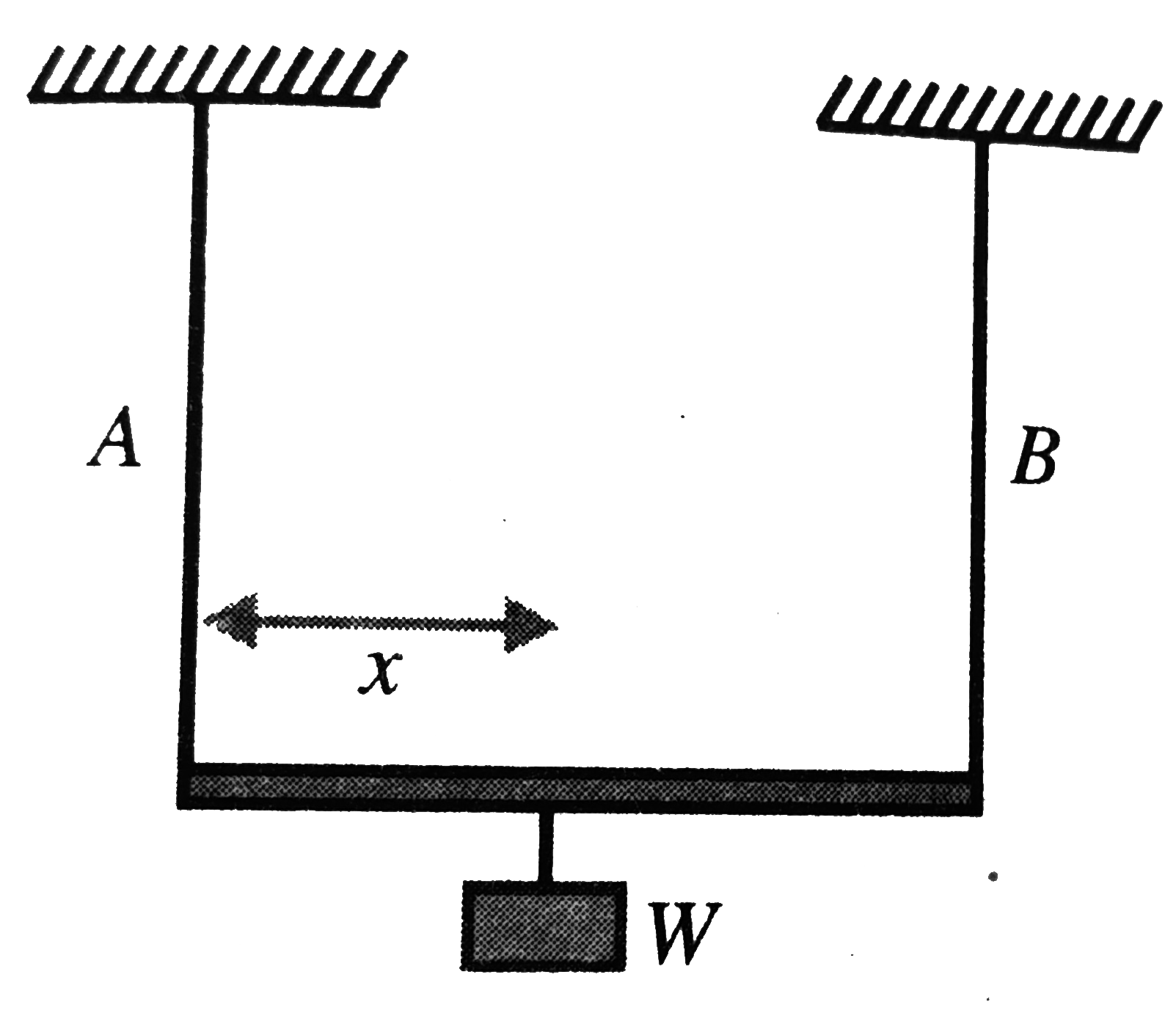
Determine the value of x so that equal strains are produced in each wire
View SolutionDetermine the elongation of the steel bar 1m long and 1.5cm2 cross-sectional area when subjected to a pull of 1.5×104N.
(TakeY=2.0×1011N/m2).
View SolutionDetermine the elongation of the steel bar 1m long and 1.5cm2 cross-sectional area when subjected to a pull of 1.5×104N.
(Take Y=2.0×1011N/m2).
View SolutionThe adjacent graph shows the extra extension (Δx) of a wire of length 1m suspended from the top of a roof at one end with an extera load Δw connected to the other end If the cross sectional area of the wire is 10−5m2 calculate the Young's modulus of the meterial of the wire
(A) 2×1011N/m2 (B) 2×10−11N/m2 (c) 3×10913)N/m3 (D) 2×1016N/m2
 .
.
View SolutionTwo rods 'A' & 'B' of equal free length hang vertically 60cm apart and support a rigid bar horizontally. The bar remains horizontal when carrying a load of 5000kg at 20cm from 'A'. If the stress in 'B' is 50N/mm2m, find the stress in 'A' and the areas of 'A' and 'B'
Given YB=9×104N/m2,YA=2×105N/mm2,g=10m/sec2
View Solution
