A rod of length l is rotated with angular speed ω about an axis passing through the centre of rod and perpendicular to its length. A uniform magnetic field B is applied parallel to the axis of rotation, Potential difference developed between the ends of the rod is
Blω22
Bl(ω2)
Blω28
Zero
The correct Answer is:D
To find the potential difference developed between the ends of a rotating rod in a uniform magnetic field, we can follow these steps:
1. Understand the Setup:
- We have a rod of length L that is rotating about its center with an angular speed ω.
- A uniform magnetic field B is applied parallel to the axis of rotation.
2. Identify the Points:
- Let’s denote the ends of the rod as point A and point B. The center of the rod is point O.
3. Determine the Length of Each Segment:
- Since the rod is rotating about its center, the distance from the center to each end (A and B) is L2.
4. Calculate the Linear Velocity:
- The linear velocity v of any point on the rod can be calculated using the formula:
v=r⋅ω
- For the ends of the rod (points A and B), r=L2, so:
vA=vB=L2⋅ω
5. Use the Formula for Potential Difference:
- The potential difference VAB between the ends of the rod in a magnetic field can be calculated using the formula:
VAB=B⋅L′⋅ω
- Here, L′ is the effective length of the rod contributing to the potential difference, which is L2 for each half of the rod.
6. Calculate the Potential Difference:
- Since both ends of the rod are at the same distance from the center, we can write:
VAB=B⋅(L2)⋅ω
- Therefore, the total potential difference developed across the ends of the rod is:
VAB=B⋅L⋅ω2
Final Answer:
The potential difference developed between the ends of the rod is:
VAB=B⋅L⋅ω2
- We have a rod of length L that is rotating about its center with an angular speed ω.
- A uniform magnetic field B is applied parallel to the axis of rotation.
- Let’s denote the ends of the rod as point A and point B. The center of the rod is point O.
- Since the rod is rotating about its center, the distance from the center to each end (A and B) is L2.
- The linear velocity v of any point on the rod can be calculated using the formula:
v=r⋅ω
- For the ends of the rod (points A and B), r=L2, so:
vA=vB=L2⋅ω
- The potential difference VAB between the ends of the rod in a magnetic field can be calculated using the formula:
VAB=B⋅L′⋅ω
- Here, L′ is the effective length of the rod contributing to the potential difference, which is L2 for each half of the rod.
- Since both ends of the rod are at the same distance from the center, we can write:
VAB=B⋅(L2)⋅ω
- Therefore, the total potential difference developed across the ends of the rod is:
VAB=B⋅L⋅ω2
The potential difference developed between the ends of the rod is:
VAB=B⋅L⋅ω2
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (MULTIPLE CHOICE QUESTIONS) (AIPMT/NEET & Other State Boards for Medical Entrance)
8 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (MULTIPLE CHOICE QUESTIONS) (JEE (Main) & Other State Boards for Engineering Entrance)
38 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Numerical Problems)
16 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A metallic rod of length lis hinged at one end and the other end is rotated with a constant angular speed ω about an axis passing through the hinge. A constant and uniform magnetic field B, parallel to the axis of rotation exists everywhere. The induced emf between the two ends of rod is 12Blω2.
View SolutionA rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
View Solution
Knowledge Check
A conducting rod rotates with a constant angular velocity 'ω' about the axis which passes through point 'O' and perpendicular to its length . A uniform magnetic field 'B' exists parallel to the axis of the rotation . Then potential difference between the two ends of the rod is :-
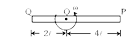 A6Bωl2BBωl2C10Bωl2DZero
A6Bωl2BBωl2C10Bωl2DZeroThe radius of gyration of an uniform rod of length L about an axis passing through its centre of mass and perpendicular to its length is.
AL√2BL2√12CL√3DL√2A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
ABl2ω2BzeroC(Bl2ω8)D2Bl2ω
(a) A metal rod of length L rotates about an end with a uniform angular velocity ω . A uniform magnetic field B exists in the direction of the axis of rotation. Calculate the emf induced between the ends of the rod. Neglect the centripetal force acting on the free electrons as they move in circular paths.
(b) A rod of length L rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. Find the potential difference (i) between the centre of rod and one end and (ii) between the two ends of the rod.
View SolutionA rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
View SolutionA uniform rod of length l is rotating in a gravity free region about an axis passing through its one end and perpendicular to it length. Choose graph between stress developed and distance the axis of roation.
View SolutionThe radius of gyration of a uniform rod of length l about an axis passing through one of its ends and perpendicular to its length is
View SolutionThe M.I. of thin uniform rod of mass 'M' and length 'l' about an axis passing through its centre and perpendicular to its length is
View Solution
