A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
zero
The correct Answer is:A
To solve the problem of finding the potential difference between the two ends of a rotating rod in a magnetic field, we can follow these steps:
1. Understand the Setup:
- We have a rod of length L rotating about its perpendicular bisector with an angular velocity ω.
- A uniform magnetic field B exists parallel to the axis of rotation.
2. Identify the Linear Velocity:
- The linear velocity v of a point at a distance x from the center of the rod is given by:
v=ωx
3. Consider a Differential Element:
- Take a small strip of the rod at a distance x from the center with a width dx.
- The induced electromotive force (emf) dE across this strip can be expressed as:
dE=B⋅v⋅dx=B⋅(ωx)⋅dx
4. Integrate to Find Total EMF:
- To find the total potential difference E between the two ends of the rod, integrate dE from −L2 to L2:
E=∫L2−L2Bωxdx
5. Perform the Integration:
- Factor out constants B and ω:
E=Bω∫L2−L2xdx
- The integral of x is:
∫xdx=x22
- Evaluating the integral from −L2 to L2:
E=Bω(x22)L2−L2=Bω⎛⎝(L2)22−(−L2)22⎞⎠
- Both terms yield the same value, thus:
E=Bω(L28−L28)=0
6. Conclusion:
- The potential difference E between the two ends of the rod is:
E=0
- We have a rod of length L rotating about its perpendicular bisector with an angular velocity ω.
- A uniform magnetic field B exists parallel to the axis of rotation.
- The linear velocity v of a point at a distance x from the center of the rod is given by:
v=ωx
- Take a small strip of the rod at a distance x from the center with a width dx.
- The induced electromotive force (emf) dE across this strip can be expressed as:
dE=B⋅v⋅dx=B⋅(ωx)⋅dx
- To find the total potential difference E between the two ends of the rod, integrate dE from −L2 to L2:
E=∫L2−L2Bωxdx
- Factor out constants B and ω:
E=Bω∫L2−L2xdx
- The integral of x is:
∫xdx=x22
- Evaluating the integral from −L2 to L2:
E=Bω(x22)L2−L2=Bω⎛⎝(L2)22−(−L2)22⎞⎠
- Both terms yield the same value, thus:
E=Bω(L28−L28)=0
- The potential difference E between the two ends of the rod is:
E=0
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 210videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises89videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Short Answer13videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises75videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises9videos
ELECTROMAGNETIC WAVES
Similar Questions
A rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
View Solution(a) A metal rod of length L rotates about an end with a uniform angular velocity ω . A uniform magnetic field B exists in the direction of the axis of rotation. Calculate the emf induced between the ends of the rod. Neglect the centripetal force acting on the free electrons as they move in circular paths.
(b) A rod of length L rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. Find the potential difference (i) between the centre of rod and one end and (ii) between the two ends of the rod.View Solution
Knowledge Check
- Question 1 - Select One
A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
AzeroB12ωBI2CωBI2D2ωBI2 - Question 2 - Select One
A conducting rod of length 2l is rotating with constant angular speed ω about is perpendicular bisector. A uniform magnetic field →B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
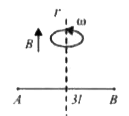 A12Bωl2B18Bωl2CZeroDBωl2
A12Bωl2B18Bωl2CZeroDBωl2 - Question 3 - Select One
A conducting rod of length 2l is rotating with constant angular speed w about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
 ABωl2B12Bωl2C18Bωl2Dzero
ABωl2B12Bωl2C18Bωl2Dzero
A conducting disc of radius r rotates with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View SolutionA metal rod length l rotates about on end with a uniform angular velocity ω. A uniform magnetic field →B exists in the direction of the axis of rotation. Calculate the emf induced between the ends of the rod. Neglect the centripetal force acting on the free electrons as they money in circular paths.
View SolutionA metallic rod of length lis hinged at one end and the other end is rotated with a constant angular speed ω about an axis passing through the hinge. A constant and uniform magnetic field B, parallel to the axis of rotation exists everywhere. The induced emf between the two ends of rod is 12Blω2.
View SolutionA conducting rod rotates with a constant angular velocity 'ω' about the axis which passes through point 'O' and perpendicular to its length . A uniform magnetic field 'B' exists parallel to the axis of the rotation . Then potential difference between the two ends of the rod is :-
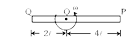 View Solution
View SolutionA rod of length l is rotated with angular speed ω about an axis passing through the centre of rod and perpendicular to its length. A uniform magnetic field B is applied parallel to the axis of rotation, Potential difference developed between the ends of the rod is
View Solution

