A rod of mass 5 kg is connected to the string at point B. The span of rod is along horizontal. The other end of the rod is hinged at point A. If the string is massless, then the reaction of hinge at the instant when string is cut, is (Take, )
10.1 N
12.5 N
5 N
15 N
The correct Answer is:B
To solve the problem step by step, we will analyze the forces and torques acting on the rod when the string is cut.
Step 1: Understand the System
We have a horizontal rod of mass m=5kg hinged at point A and connected to a massless string at point B. When the string is cut, the only forces acting on the rod are its weight and the reaction force at the hinge.
Step 2: Identify Forces
The weight of the rod acts downward at its center of mass, which is located at a distance L/2 from point A. The weight W can be calculated as:
W=mg=5kg×10m/s2=50N
Step 3: Calculate Torque
When the string is cut, the rod will start to rotate about point A due to the torque created by the weight of the rod. The torque τ about point A due to the weight of the rod is given by:
τ=W×perpendicular distance=W×L2
Substituting the value of W:
τ=50N×L2
Step 4: Moment of Inertia
The moment of inertia I of the rod about point A (the hinge) is given by:
I=13mL2
Step 5: Angular Acceleration
Using Newton's second law for rotation, we have:
τ=Iα
Substituting the expressions for torque and moment of inertia:
50×L2=13mL2α
This simplifies to:
25L=53L2α
Cancelling L (assuming L≠0):
25=53Lα
Solving for α:
α=25×35L=15L
Step 6: Linear Acceleration of the Center of Mass
The linear acceleration a of the center of mass (which is at L/2) can be related to angular acceleration α by:
a=α⋅L2=15L⋅L2=152m/s2
Step 7: Apply Newton's Second Law
Now, we can apply Newton's second law to the vertical motion of the center of mass:
Net Force=m⋅a
The forces acting on the rod are its weight mg downward and the reaction force R upward at the hinge:
mg−R=ma
Substituting the values:
50−R=5⋅152
Calculating the right side:
50−R=5⋅7.5=37.5
Thus:
R=50−37.5=12.5N
Conclusion
The reaction force at the hinge when the string is cut is:
R=12.5N
We have a horizontal rod of mass m=5kg hinged at point A and connected to a massless string at point B. When the string is cut, the only forces acting on the rod are its weight and the reaction force at the hinge.
The weight of the rod acts downward at its center of mass, which is located at a distance L/2 from point A. The weight W can be calculated as:
W=mg=5kg×10m/s2=50N
When the string is cut, the rod will start to rotate about point A due to the torque created by the weight of the rod. The torque τ about point A due to the weight of the rod is given by:
τ=W×perpendicular distance=W×L2
Substituting the value of W:
τ=50N×L2
The moment of inertia I of the rod about point A (the hinge) is given by:
I=13mL2
Using Newton's second law for rotation, we have:
τ=Iα
Substituting the expressions for torque and moment of inertia:
50×L2=13mL2α
This simplifies to:
25L=53L2α
Cancelling L (assuming L≠0):
25=53Lα
Solving for α:
α=25×35L=15L
The linear acceleration a of the center of mass (which is at L/2) can be related to angular acceleration α by:
a=α⋅L2=15L⋅L2=152m/s2
Now, we can apply Newton's second law to the vertical motion of the center of mass:
Net Force=m⋅a
The forces acting on the rod are its weight mg downward and the reaction force R upward at the hinge:
mg−R=ma
Substituting the values:
50−R=5⋅152
Calculating the right side:
50−R=5⋅7.5=37.5
Thus:
R=50−37.5=12.5N
The reaction force at the hinge when the string is cut is:
R=12.5N
Topper's Solved these Questions
Similar Questions
A rod PQ of mass M and length L is hinged at end P. The rod is kept horizontal by a massless string tied to point Q as shown in the figure. When string is cut, the initial angular accleration of the rod is.

View SolutionA rod PQ of mass M and length L is hinged at end P. The rod is kept horizontal by a massless string tied to point Q as shown in figure. When string is cut, the intial angular acceleration of the rod is

View SolutionTwo particle of masses 10 kg and 35 kg are connected with four strings at points B and D as shown in fig.

Determine the tension in various segments of the string.
View SolutionA string 1 m long can just support a weight of 16 kg. Amass of 1 kg is attached to one of its ends. The body is revolved in a horizontal circle about the other fixed end of the string. Find the greatest number of revolutions made by the mass per second ? (Take g = 10ms−2)
View SolutionA uniform rod of mass m is hinged at one end and other end is connected to a light extensible string having length L.If the Youngs modulus and crosssectional area of the wire is Y and A respectively then the elongation in the string is
View Solution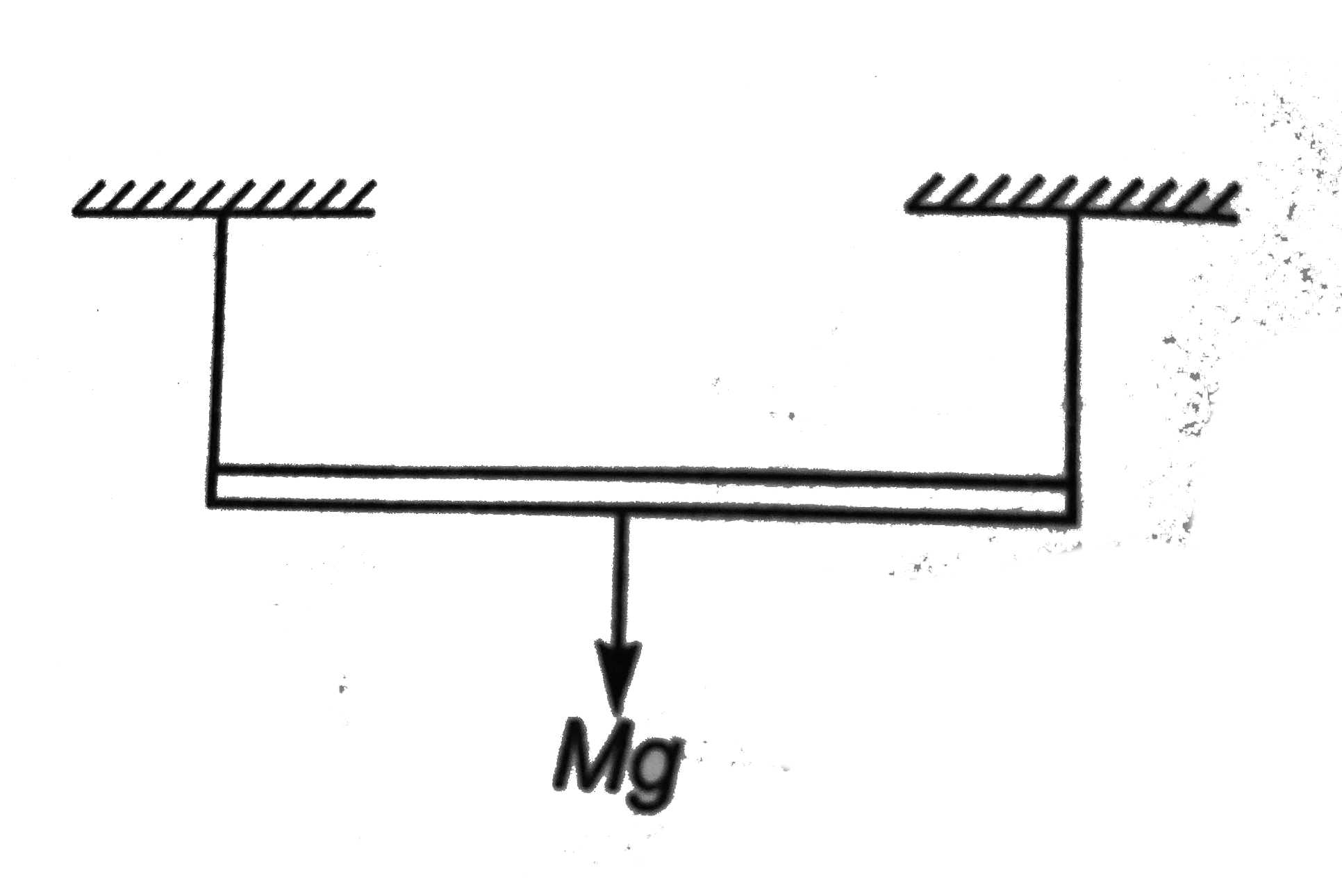
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.
View SolutionA uniform rod of length 1m and mass 2kg is suspended. Calculate tension T (in N) in the string at the instant when the right string snaps (g=10m/s2).

View SolutionA uniform rod length is l hinged at A and suspended with vertical string as shown in figure it string is cut then acceleration of centre of mass at this instant will be (g=10ms2)
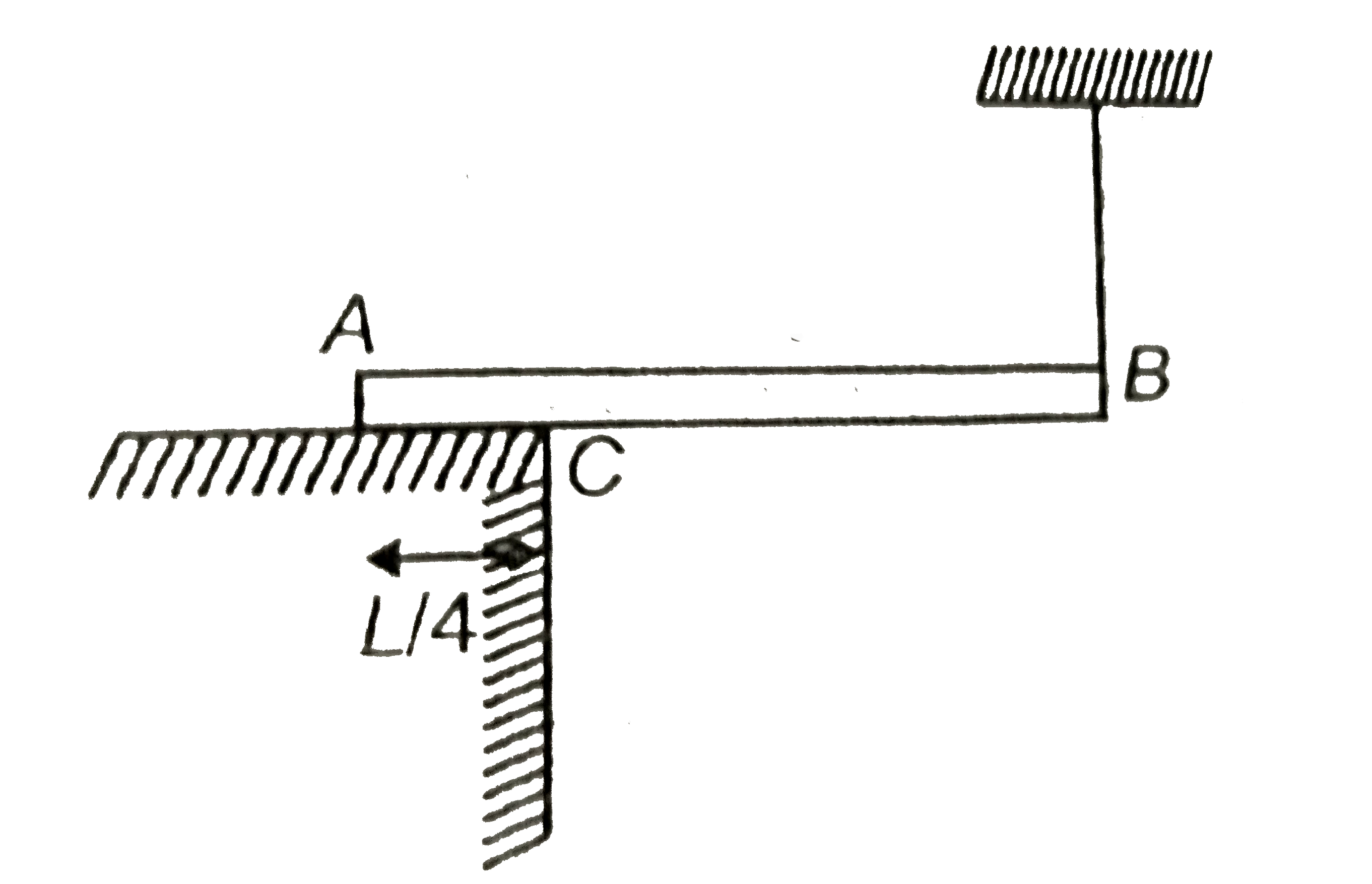
View SolutionA unifrom rod of mass m and length L is kept on a horizontal table with L4 length on the table. The end B is tied to a string as shown in the figure. The string attached to the end B is cut and the rod starts rotating about point C. Find the normal reaction from the table on the earth as soon as the string is cut.
View SolutionAn object of mass 10kg is connected to the lower end of a massless string of length 4m hanging from the ceiling. If a force F is applied horizontally at the mid-point of the string, the top half of the string makes an angle of 45∘ with the vertical , then the magnitude of F is
View Solution
