A rod of mass m and length 2R can rotate about an axis passing through O invertical plane.A disc of mass m and radius R/2 is hinged to the other end P ofthe rod and can freely rotate about P. When disc is at lowest point both rod and disc has angular velocity ω. If rod rotates by maximum angleθ=60∘ with downward vertical, then ω interms of R and g will be(all hinges are smooth)
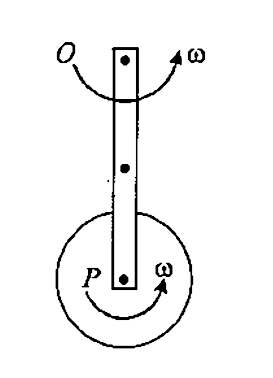
√9g16R
√3g23R
13√gR
None of these
The correct Answer is:A
Step by step video, text & image solution for A rod of mass m and length 2R can rotate about an axis passing through O invertical plane.A disc of mass m and radius R//2 is hinged to the other end P ofthe rod and can freely rotate about P. When disc is at lowest point both rod and disc has angular velocity omega. If rod rotates by maximum angletheta=60^(@) with downward vertical, then omega interms of R and g will be(all hinges are smooth) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - MCQ
16 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical
63 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Numerical MCQs Single Option Corrrect
39 Videos
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Unsolved Numerical Problem
81 Videos
- WORK, ENERGY AND POWERBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - unsolved
1 Videos
Similar Questions
Asphere, a disc and a ring of the same mass but of different density and radii are allowed to roll down on an inclined plane without slipping simultaneously, through the same height, then:
View SolutionTwo masses M and m are connect by a light string gong over a pulley of radis r. The pulley is free to rotate about its axis which is kept horizontal. The moment of inertia of the pulley about the axis is I. The system is releaed from rest. Find the angular momentum fo teh system when teh mass Mhas descended through a height h. The string does not slip over the pulley.
View SolutionThe moment of inertia of cylinder of radius a, mass M and height h about an axis parallel to the axis of the cylinder and distance b from its centre is :
View SolutionA packet of 150kg is released from an air plane travelling due east at an altitude of 7200 m with a ground speed of 100 m/s. The wind applies a constant force on the packet of 300 N directed horizontally in the opposite direction to the plane's flight path. Where and when does the packet hit the ground, (with respect to the release location and time)
View SolutionFigure shows as rod of length 20 cm pivoted near an end and which is made to rotate in a horizontal plane with a constant angular speed. A ball ofmass m is spuspended by a string angular also of length 20 cm from the other end of the rod. If the angle θ made by the stirng with the vertical is 300. find the anglular speed of the rotation. Take g=10ms2
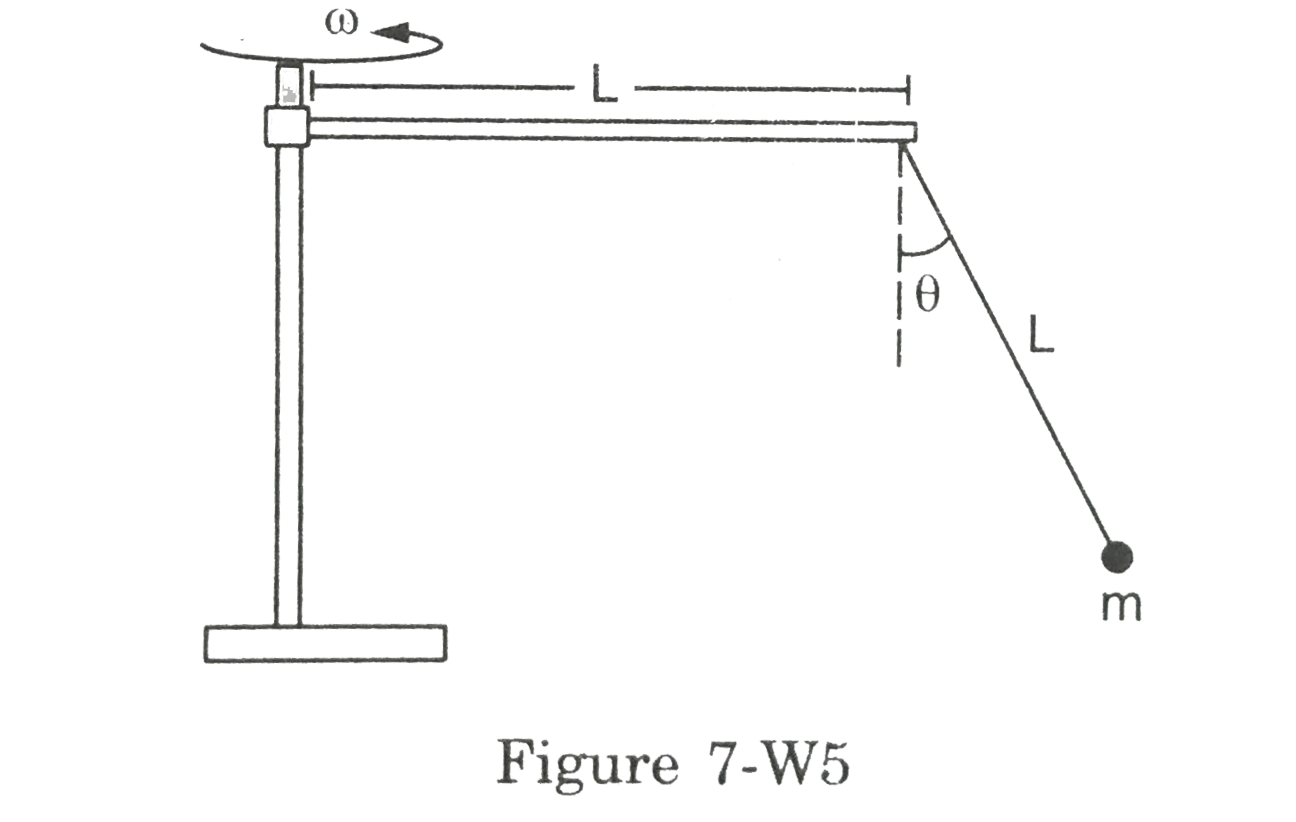
View Solution
A rod of mass 2 kg ad length 2 m is rotating about its one end O wth an angular velocity ω=4rad/s. Find angular momentum of the rod about the axis rotation.
View SolutionA uniform rod of mass m is rotated about an axis passing through point O as shown. Find angular momentum of the rod about rotational law.
View SolutionA conical pendulum, a thin uniform rod of length l and mass m , rotates uniformly about a vertical axis with angular velocity omega (the upper end of the rod is hinged). Find the angle theta between the rod and the vertical.
View SolutionIn the figure (i) a disc of mass M(kg) and radius R(m) is rotating smoothly about a fixed vertical axis AB with angular speed 26 rad//s A rod rod CD of length (R)/(2)(m) and mass M(kg) is hinged at one end at point 'D' on the disc. The rod remains in vertical position and rotates along with the disc about axis AB . At some moment the rod CD gets a very smal impulse at point 'C' due to air due to which the rod falls on the disc along one radius and sticks to the disc as shown in figure (ii) . Now find the angular velocity of the disc in rad//s .
View SolutionA uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is

View SolutionA mechanism consists of a part which is translated with a velocity u and a rod AB of length L and mass M hinged at A The rod rotates about axis A with angular velocity omega the kinetic energy of rod when it is vertical as shown is
View SolutionA rod of mass m and length l is rotating about a fixed point in the ceiling with an angular velocity omega as shown in the figure. The rod maintains a constant angle theta with the vertical. What is the rate of change of angular momentum of the rod ?
View SolutionA rod of mass m and length l is rotating about a fixed point in the ceiling with an angular velocity omega as shown in the figure. The rod maintains a constant angle theta with the vertical. What angle will the rod make with the vertical
View SolutionA uniform rod of mass M and length L lies radially on a disc rotating with angular speed ω in a horizontal plane about its axis. The rod does not slip on the disc and the centre of the rod is at a distance R from the centre of the disc. Then the kinetic energy of the rod is :

View SolutionA rod of mass 2 kg ad length 2 m is rotating about its one end O with an angular velocity omega=4rad//s . Find angular momentum of the rod about the axis rotation.
View Solution
