A rope of total mass m and length L is suspended vertically. Show that a transverse pulse travels the length of the rope in a time interval Δt=2√L/g. Sugestion: first find an expression for the wave speed at any point a distance x from the lower end by considering the rope's tension as resulting from the weight of the segment below that point.
To solve the problem, we need to find the time interval Δt for a transverse pulse to travel the length of a vertically suspended rope. We will derive the expression step by step.
Step 1: Understanding the Tension in the Rope
The tension T in the rope at a distance x from the lower end is due to the weight of the segment of the rope below that point. The mass of the segment of the rope below point x can be expressed as:
mx=mL⋅(L−x)
where m is the total mass of the rope and L is its total length.
The weight of this segment, which contributes to the tension, is:
T=mx⋅g=(mL⋅(L−x))⋅g=mg(L−x)L
Step 2: Finding the Wave Speed
The wave speed v in a medium is given by:
v=√Tμ
where μ is the linear mass density of the rope, defined as:
μ=mL
Substituting the expression for tension T:
v=
⎷mg(L−x)LmL=√g(L−x)
Step 3: Setting Up the Time Calculation
To find the time Δt for the pulse to travel from the bottom of the rope (at x=0) to the top (at x=L), we need to integrate the time taken to travel an infinitesimal distance dx:
Δt=∫L0dxv=∫L0dx√g(L−x)
Step 4: Evaluating the Integral
Substituting v=√g(L−x):
Δt=∫L0dx√g(L−x)=1√g∫L0dx√L−x
Now, we can use the substitution u=L−x, which gives dx=−du:
Δt=1√g∫0L−du√u=1√g∫L0du√u=1√g⋅2√L
Step 5: Final Expression for Time Interval
Thus, we find:
Δt=2√Lg
This shows that the time interval for a transverse pulse to travel the length of the rope is:
Δt=2√Lg
The tension T in the rope at a distance x from the lower end is due to the weight of the segment of the rope below that point. The mass of the segment of the rope below point x can be expressed as:
mx=mL⋅(L−x)
where m is the total mass of the rope and L is its total length.
T=mx⋅g=(mL⋅(L−x))⋅g=mg(L−x)L
The wave speed v in a medium is given by:
v=√Tμ
where μ is the linear mass density of the rope, defined as:
μ=mL
v=
⎷mg(L−x)LmL=√g(L−x)
To find the time Δt for the pulse to travel from the bottom of the rope (at x=0) to the top (at x=L), we need to integrate the time taken to travel an infinitesimal distance dx:
Δt=∫L0dxv=∫L0dx√g(L−x)
Substituting v=√g(L−x):
Δt=∫L0dx√g(L−x)=1√g∫L0dx√L−x
Δt=1√g∫0L−du√u=1√g∫L0du√u=1√g⋅2√L
Thus, we find:
Δt=2√Lg
Δt=2√Lg
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Example9videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Exercise 5.19videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
Derive an expression for the magnetic field at a point on the axis located at a distance x from the centre of a magnetic dipole of length 2l and magnetic moment M.
View SolutionA metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
View Solution
Knowledge Check
- Question 1 - Select One
A rope of length L is pulled by a constant force F. What is the tension in the rope at a distance x from the end where the force is applied ?
AFL/xBF(L−x)/LCFL/(L−x)DFx/(L−x)
A uniform rope of mass (m) and length (L) placed on frictionless horizontal ground is being pulled by two forces FAandFB at its ends as shown in the figure. As a result, the rope accelerates toward the right.
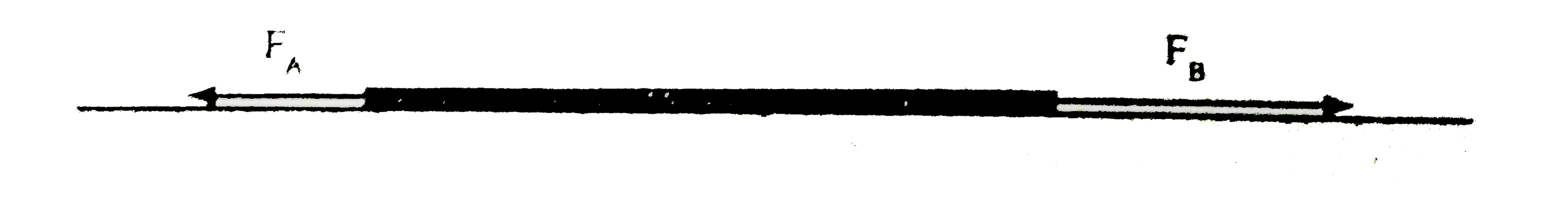
Expression Tx of tension at a point at distance x from the end A isView SolutionA uniform rope of mass m and length L hangs from a celling. (a) Show that the speed of a transverse wave on the rope is a function of y, the distance from the lower end, and is given by t=2√L/g.
View SolutionA rope of length L and mass m hangs freely from the ceiling. The velocity of transverse wave as a function of position x-along the rope is proportional to
View SolutionA uniform rope of mass M length L hangs vertically from the ceiling, with lower end free. A distbance on the rope trvelling upwards starting from the lower end has a velocity v. At a point P at distance x from the lower end.
View SolutionA uniform rope of mass 0.1kg and length 2.45m hangs from a ceiling.
(a) Find the speed of transverse wave in the rope at a point 0.5m distant from the lower end.
(b) Calculate the time taken by a transverse wave to travel the full length of the rope.
 View Solution
View SolutionA rod of length L is suspended vertically from a point at a distance x from one end to oscillate under gravity. What should be x (approximately) so that it oscillates with minimum time period?
View SolutionA Uniform rope having mass m hags vertically from a rigid support. A transverse wave pulse is produced at the lower end. The speed v of wave pulse varies with height h from the lower end as
View Solution

