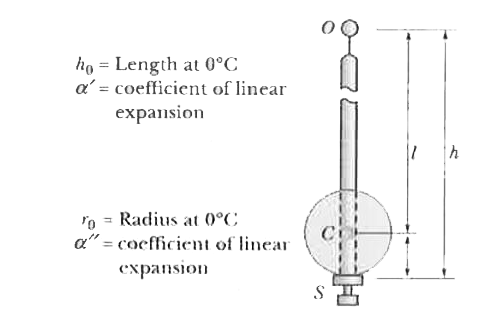
A second's pendulum clock has shown in Fig . It is compensated for temperature change by using differential expression of the light rod and the heavy bob. This keeps the center of oscillation of the pendulum at a fixed distance below the point of suspension. Find the length of the light rod, $h_{0}$ and radius $r_{0}$ , of the heavy bob of this pendulum . Given that $α^{''} > > α^{'}$

Text Solution

Verified by Experts

KEY IDEA
A second.s pendulum clock has the period 2 s. We can consider the centre of the bob C as approximately the centre of oscillation of the pendulum , the effective length of the pendulum is l = h = r . If expansion coefficient are so chosen that the difference of the length remains constant , then we will have constant , time period irrespective of the temperature change.
Calculations : Now ,
$h = h_{0} (1 + α^{.} Δ T)$
$r = r_{0} (1 + α^{. .} Δ T)$
Where $Δ α^{. .} > > α^{.}$ . Then , $l = h_{0} - r_{0} (h_{0} α^{.} - r_{0} α^{. .}) Δ T$ ,which will be independent of temperature if
$(h_{0} α^{.} - r_{0} α^{. .}) = 0$
Also, $l_{0} = h_{0} - r_{0}$ ,
Where $l_{0}$ is length that must be maintained . Solving these last two equations for $h_{0} and r_{0}$ , we get
$h_{0} = \frac{α^{. .} l_{0}}{a^{. .} - α^{.}}, r_{0} = \frac{α^{.} l_{0}}{α^{. .} - α^{.}}$
The period (2 s) of the pendulum is
$τ = 2 π \sqrt{\frac{l_{0}}{g}} \Rightarrow 2 = 2 π \sqrt{\frac{l_{0}}{g}} \Rightarrow l_{0} = \frac{g}{π^{2}}$
Finally , the dimension $(h_{0}, r_{0})$ of the pendulum becomes
$h_{0} = \frac{α^{. .} l_{0}}{α^{. .} - α^{.}} = \frac{α^{. .} (g / π^{2})}{α^{. .} - α^{.}}$ (Answer)
And $r_{0} = \frac{α^{.} (g / π^{2})}{α^{. .} - α^{.}}$ (Answer)
Note if we would have used same material , that is , $α^{. .} - α^{.} = 0$ the required dimensions would have become infinite or in other words , using same material will not ensure the temperature independence .

Updated on:21/07/2023