A pendulum bob is given an intial velocity v at this bottom most point and it is found that bob loses circular track at a certain point and hits the point of suspension . If l is length of the thread then find v .
Let v be the velocity of bob at the point where it loses circular track and assume that string makes an angle θ with the vertical at this point as shown in figure .
Let O be the point where bob leaves the point O is on the circular path hence we can apply circular dynamics for this point .
T+mgcosθ=mu2l
Bob loses circular track at this point hence we can sustitute T = 0 in above equation .
u=√glcosθ (i)
Let O the origin and axes are as shown in figure . Bob moves under gravity like a projectile after point O till it hits the point of suspension
Sx=uxt+12axt2⇒lsinθ=(ucosθ)t+12(0)t2
⇒ t=lsanθucosθ ...(ii)
Sy−uyt+12ayT2=lcosθ=(−usinθ)t+12gt2
Substituting value of t from equation (ii) we get
lcosθ=(−usinθ)(lsinθucosθ)+12g(lsinθucosθ)2
⇒ cosθ=(−sinθ)(sinθcosθ)+12gl(sinθucosθ)2
⇒ cosθ+sin2θcosθ=12gl(sin2θu2cos2θ)
⇒ 1cosθ=12gl(sin2θu2cos2θ)
⇒ 12gl(sin2θu2cosθ)=1
⇒12(glsin2θglcosθ×cosθ)=1
⇒ tan2θ=2⇒tanθ=√2 ....(i)
We can now apply conservation of mechnical energy between the bottom most point and the point where it loses the circular track .
Loss of kinetic energy = gain in gravitational potential energy .

Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Textbook Exercise)
23 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Additional Exercise)
7 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Conceptual Questions
20 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
The bob of a simple pendulum of length l is released from a point in the same horizontal line as the point of suspension and at a distance l from it Calculate the tension in the string at the lowest point of its swing
(b) If the string of the pendulum is catched by a nail located verticaly below the point of suspensions and the bob just swings around a complete circle around the nail, find the distance of the nail from the point of suspension
(c) If the string of the pendulum is made of rubber then show that it will be stretched by 3 mg/k on reaching the bob at the lowest point. Here k is the force constant of the string
View SolutionA simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
View Solution
Knowledge Check
A simple pendulum has a length l . What minimum velocity should be imparted to its bob at the mean position so that bob reaches a height equal to l above the point of suspension?
A√grB√5glC√2glD√lgA simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
A3mgB4mgC5mgD6mgA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
AmL√2gLBmL√gLCmL√3gLDnone
A bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
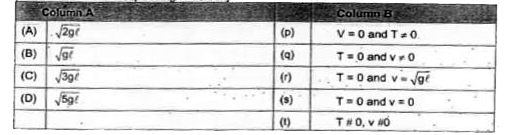
View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II

View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionThe bob of a simple pendulum is given a sharp hit impart it a horizontal speed of √3gL. Find an angle made by the string with the upper verticle before it becomes slack. Also, calculate the maximum height attained by the bob above the point of suspension. L is length of the string.
View SolutionA simple pendulum has a string of length l and bob of mass m. When the bob is at its lowest position , it is given the minimum horizontal speed necessary for it to move in a circular path about the point point of suspension. The tension in the string at the lowest position if the bob is
View Solution
