A skier plane to ski a smooth fixed hemisphere of radius R . He starts from rest from a curve smooth surface of height (R4) . The angle θ at which he leaves the hemisphere is 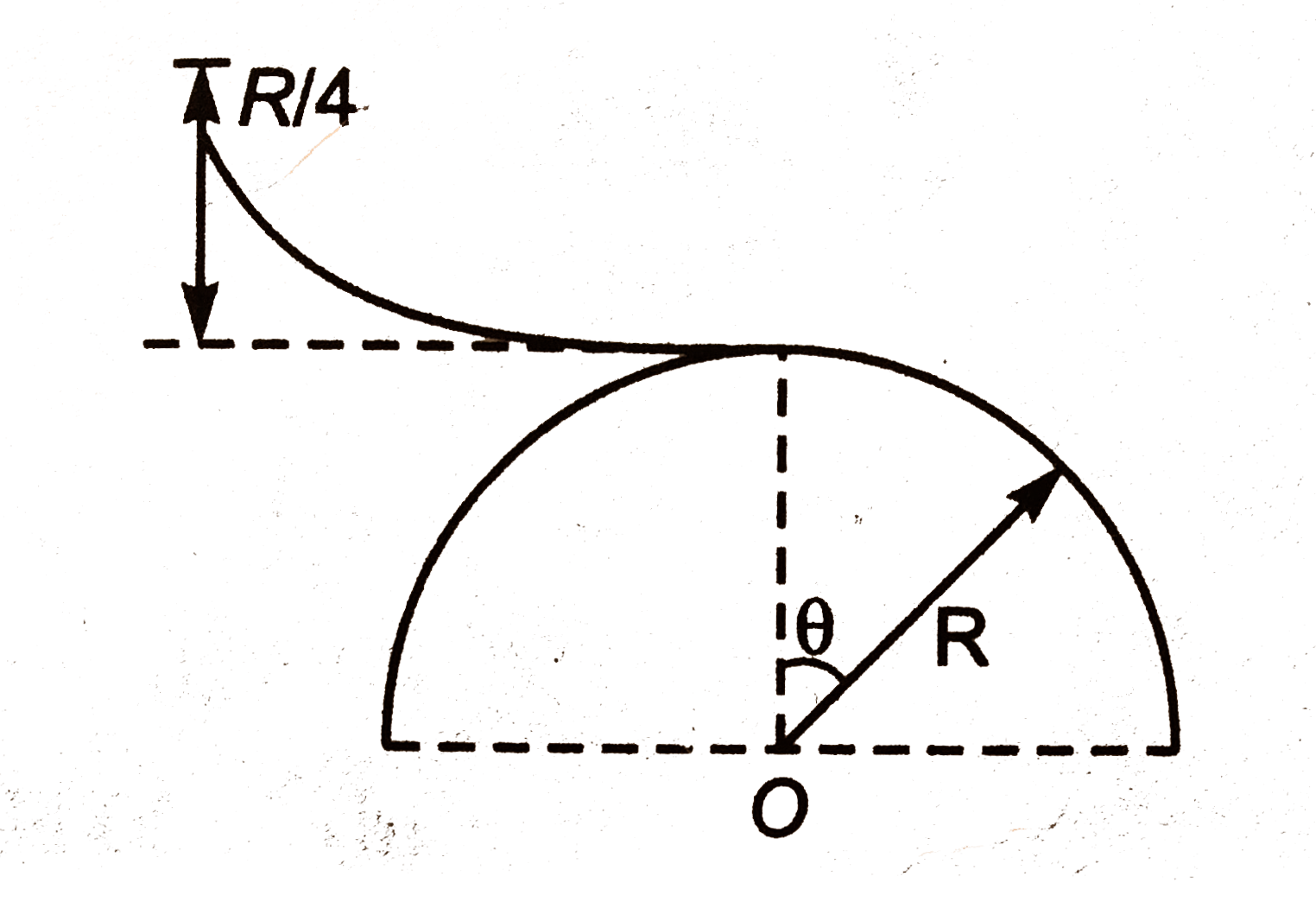
cos−1(23)
cos−1(5)√3
cos−1(56)
cos−1[52√3]
The correct Answer is:C
At the time of leaving contact
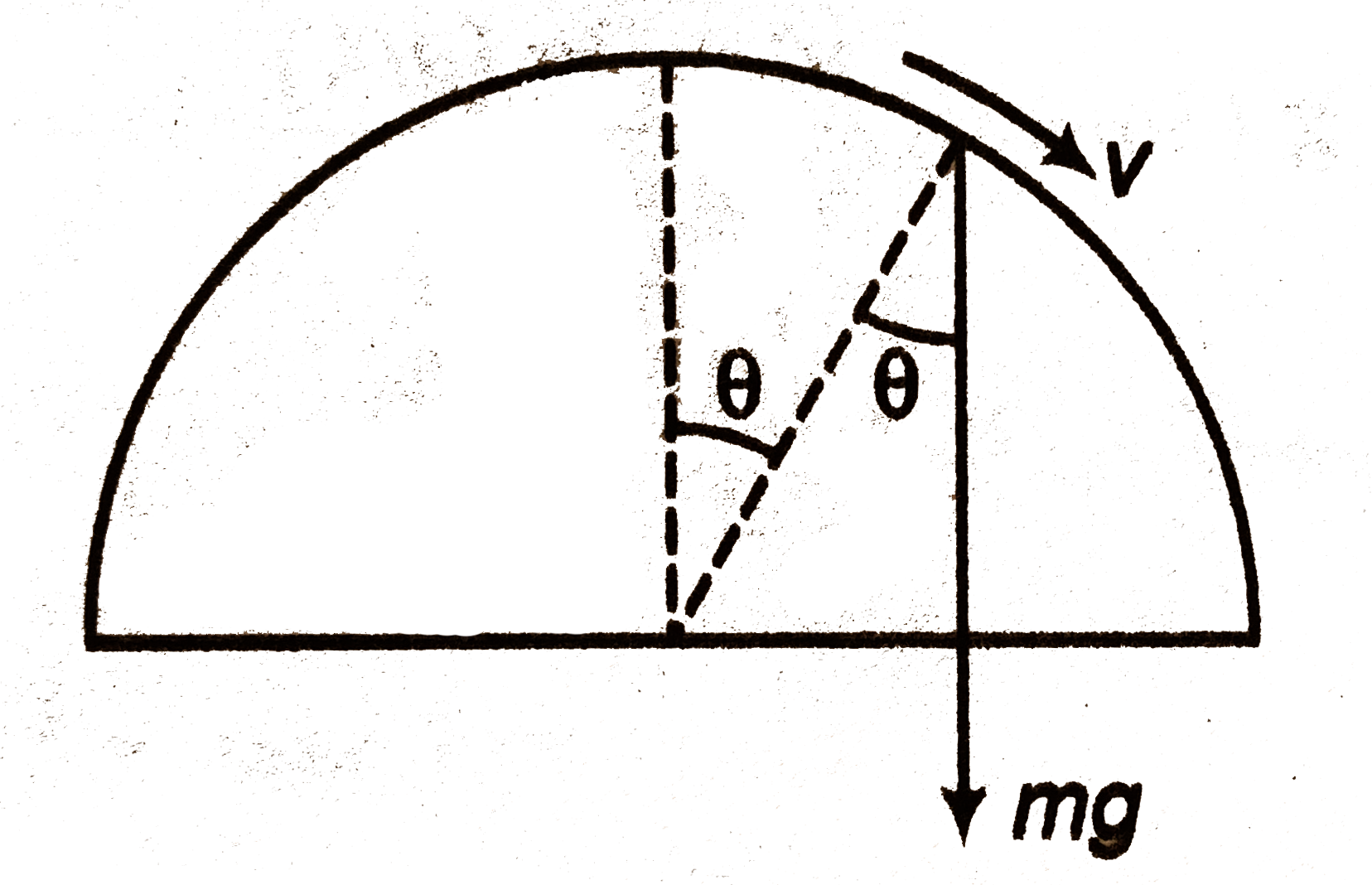
N=0
:. mgcosθ=mv2R=m(2gh)R
:. cosθ=2hR=[R4+R(1−cosθ)]R
On solving this equation, we get
cosθ=5/6 or θ=cos−1(56)
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 More Than One Correct
5 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 Comprehension Based
5 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Level 1 Subjective
13 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A particle of mass m is placed in equlibrium at the top of a fixed rough hemisphere of radius R. Now the particle leaves the contact with the surface of the hemisphere at angular position θ with the vertical wheere cosθ35. if the work done against frictiion is 2mgR10x, find x.
View SolutionParticle slides down the surface of a smooth fixed sphere of radius r starting from rest at the highest point. Find where it will leave the sphere. Show that it strike the horizontal plane through the lowest point of the sphere at a distance equal to 5[√5+4√2](r/27) from the vertical diameter.
View Solution
Knowledge Check
A skier plans to ski a smooth fixed hemisphere of radius R He starts from rest from a curved smooth surface of height (R/4) the angle θ at which he leaves the hemisphere is
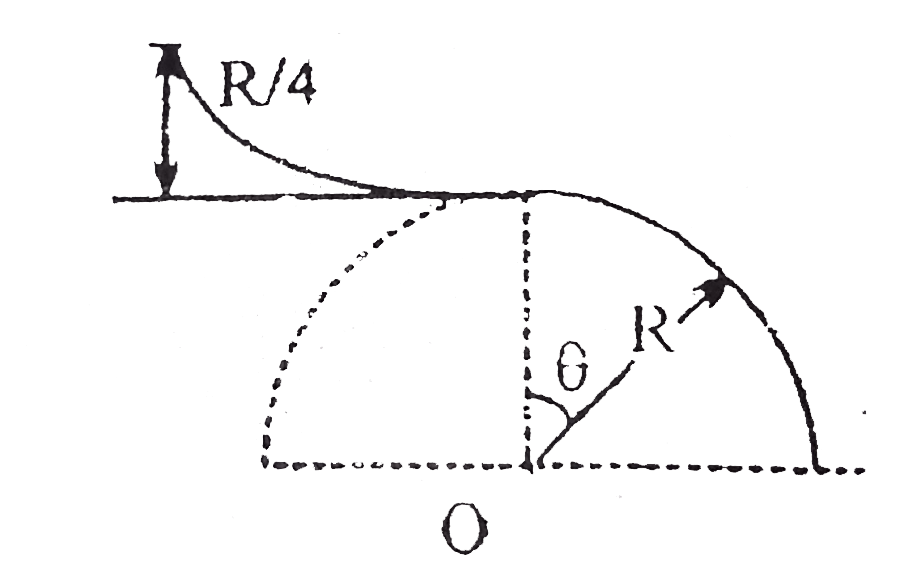 Acos−1(2/3)Bcos−1(5/√3Ccos−1(5/6)Dcos−1(5/2√3
Acos−1(2/3)Bcos−1(5/√3Ccos−1(5/6)Dcos−1(5/2√3A small block of mass m projected horizontally from the top of the smooth and fixed hemisphere of radius r with speed u as shown. For values of u≥u0(u0=√gr) it does not slide on the hemisphere. l i.e., leaves the surface at the top itself.
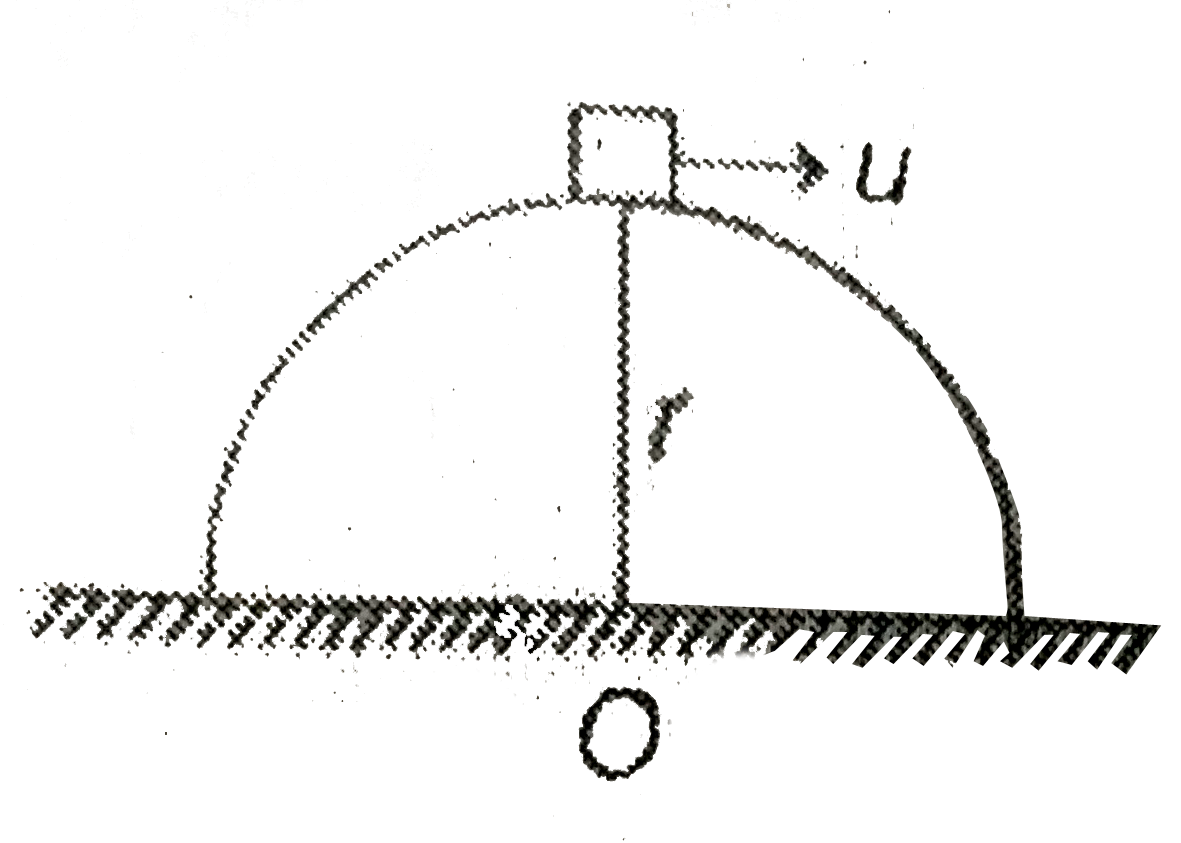
For u=u0/3, Find the height from the ground at which it leaves the hemisphereA19r9B19r27C10r9D10r27A body of mass Mkg is on the top point of a smooth hemisphere of radius 5m . It is released to slide down the surface of hemisphere it leaves the surface when its velocity is 5ms−1 At this instant the angle made by the radius vector of the body with the vertical is ( Take g=10m/s2)
A30∘B60∘C45∘D20∘
Find the CG of :(a) a cone of height h from the base,(b) a hemisphere of radius r from its flat surface.
View SolutionA small block of mass m projected horizontally from the top of the smooth and fixed hemisphere of radius r with speed u as shown. For values of u≥u0(u0=√gr) it does not slide on the hemisphere. l i.e., leaves the surface at the top itself.
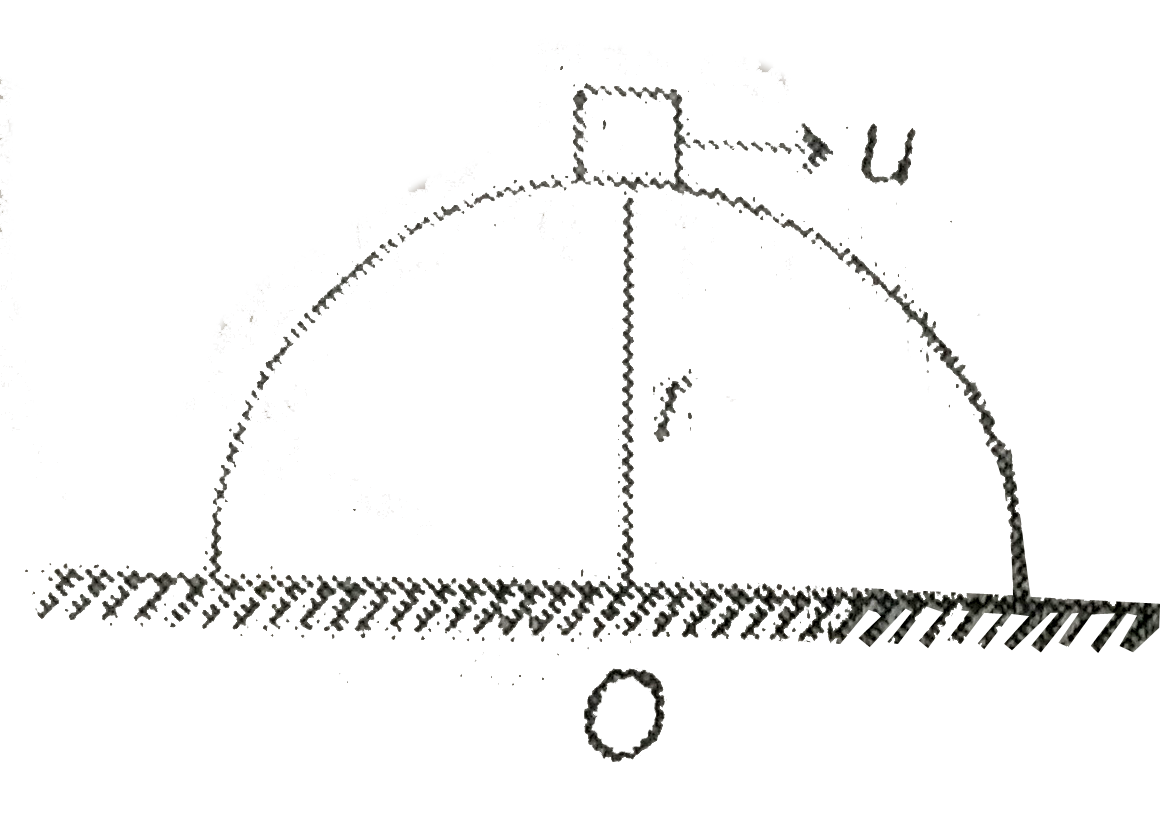
In the above problem find its net acceleration at the instant it levels the hemisphere.
View SolutionA small body of mass m slides without friction from the top of a hemisphere of radius r. At what height will the body be detached from the surface of hemisphere?

View SolutionA partcle rests on the top of a hemisphere of radius R. Find the smallest horizontal velocity that must be imparted to the particle if it is to leave the hemisphere without sliding down :
View SolutionA body rest on the top of a hemisphere of radius R. What will be the least horizontal velocity imparted to it , if it has to leave the hemisphere without sliding down?
View Solution
