Question

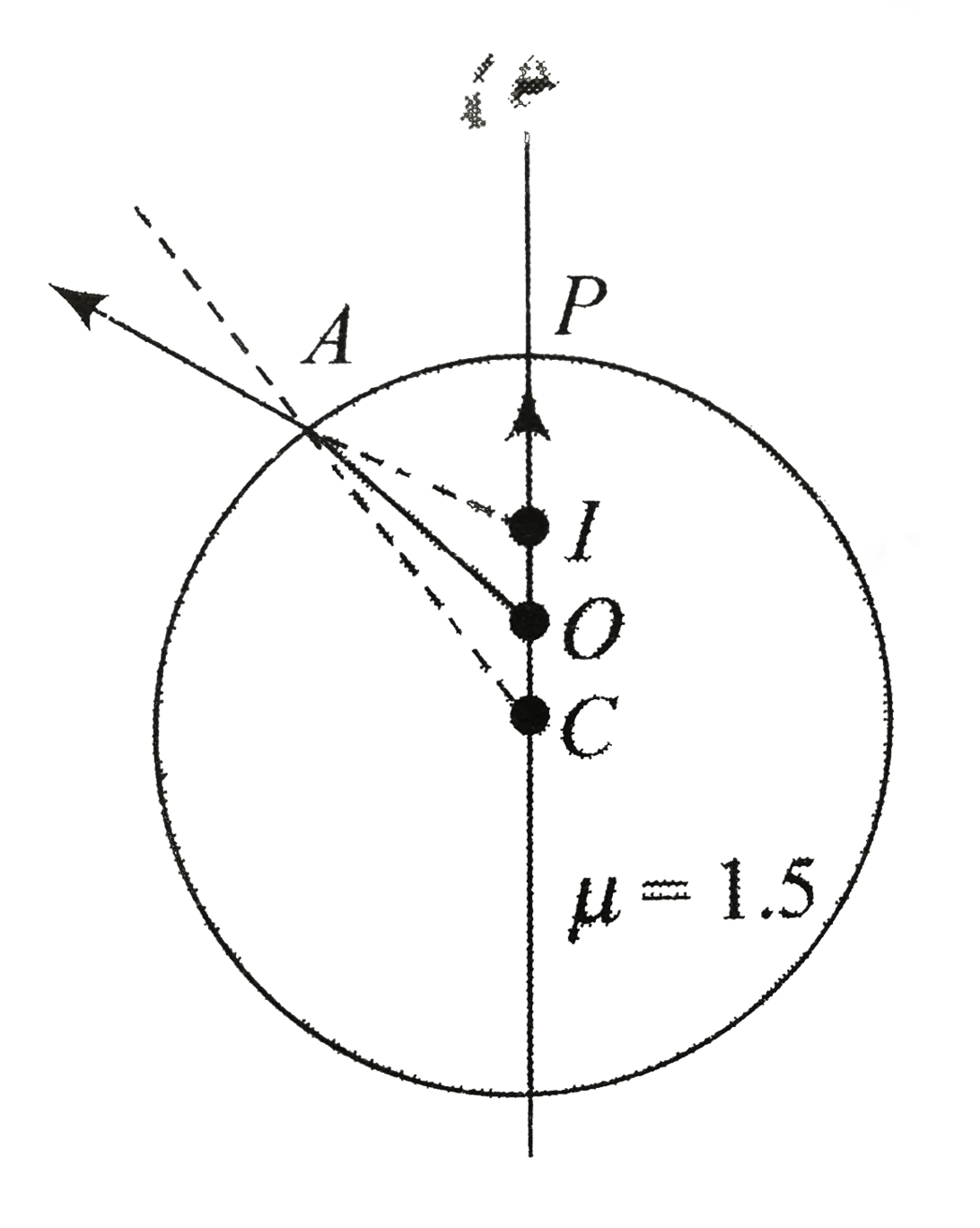
A small air bubble in a sphere of glass with radius 4 cm appears to be 1 cm from the surface when observed along a diameter. Find the true positino of the air bubble.

Explanation

To solve the problem of finding the true position of the air bubble in a glass sphere, we will follow these steps:

1. Understanding the Problem:
- We have a glass sphere with a radius of 4 cm.
- An air bubble inside the sphere appears to be 1 cm from the surface when viewed along a diameter.

2. Setting Up the Coordinate System:
- Let the center of the sphere be at the origin (0, 0).
- The radius of the sphere is 4 cm, so the surface of the sphere is at x = ±4 cm.
- The air bubble appears to be at a distance of 1 cm from the surface, which means it appears to be at x = 4 cm - 1 cm = 3 cm from the center of the sphere.

3. Identifying the Image Distance (V):
- Since the bubble appears to be at 3 cm from the center, we can denote this as the image distance (V) from the center of the sphere.
- Therefore, V = -1 cm (as it is in the negative x-direction).

4. Using the Lens Maker's Formula:
- The lens maker's formula for refraction at a spherical surface is given by:
\( \frac{\mu_2}{V} - \frac{\mu_1}{u} = \frac{\mu_2 - \mu_1}{R} \)
- Here, \(\mu_1\) is the refractive index of glass (approximately 1.5), \(\mu_2\) is the refractive index of air (approximately 1), \(R\) is the radius of curvature (which is -4 cm for the surface of the sphere), and \(u\) is the object distance we want to find.

5. Substituting Values into the Formula:
- Substituting the known values:
\( \frac{1}{-1} - \frac{1.5}{u} = \frac{1 - 1.5}{-4} \)
- This simplifies to:
\( -1 - \frac{1.5}{u} = \frac{-0.5}{-4} \)
- Further simplifying gives:
\( -1 - \frac{1.5}{u} = \frac{0.5}{4} \)
- Which simplifies to:
\( -1 - \frac{1.5}{u} = 0.125 \)

6. Rearranging the Equation:
- Rearranging gives:
\( -\frac{1.5}{u} = 0.125 + 1 \)
- Thus:
\( -\frac{1.5}{u} = 1.125 \)

7. Solving for u:
- Multiplying both sides by -u gives:
\( 1.5 = -1.125u \)
- Therefore:
\( u = \frac{1.5}{-1.125} = -\frac{12}{9} = -\frac{4}{3} \text{ cm} \)

8. Conclusion:
- The true position of the air bubble is \(-\frac{4}{3}\) cm from the center of the sphere.

Final Answer:
The true position of the air bubble is \(-\frac{4}{3}\) cm from the center of the sphere.

---