A glass sphere of diameter 50 cm and μ=1.5 has a small air bubble. Looking from outside the diameter, the bubble appears to be at a distance 10 cm the surface. Find the apparent position of the bubble when it is viewed from the diametrically opposite position.
real image, at the pole of 2 nd face
real image, at the pole of 1st face
virtual image, at the pole of 1st face
virtual image, at the centre of the sphere
The correct Answer is:C
To solve the problem, we need to find the apparent position of the air bubble when viewed from the diametrically opposite position of the glass sphere. We will use the lens maker's formula and the concept of refraction to find the position.
1. Identify Given Values:
- Diameter of the glass sphere (D) = 50 cm
- Radius of the sphere (R) = D/2 = 25 cm
- Refractive index of glass (μ) = 1.5
- Apparent distance of the bubble from the surface (v1) = 10 cm
2. Determine the Object Distance:
- The object (air bubble) is inside the sphere. The distance from the center of the sphere to the bubble can be calculated as:
u=R−v1=25 cm−10 cm=15 cm
- Here, u is taken as negative because the object is inside the sphere.
3. Use the Lens Formula:
The lens formula is given by:
1f=1v−1u
where:
- f = focal length
- v = image distance (which we need to find)
- u = object distance
4. Calculate the Focal Length:
The focal length (f) for a spherical interface can be calculated using the formula:
f=R2(μ−1)
Substituting the values:
f=25 cm2(1.5−1)=25 cm2×0.5=25 cm1=25 cm
5. Apply the Lens Formula:
Now we can apply the lens formula:
125=1v−1−15
Rearranging gives:
1v=125−115
Finding a common denominator (75):
1v=375−575=−275
Thus:
v=−752=−37.5 cm
6. Interpret the Result:
The negative sign indicates that the image is virtual and located on the same side as the object (inside the sphere). The apparent position of the bubble when viewed from the diametrically opposite position is 37.5 cm from the center of the sphere.
Final Result:
The apparent position of the bubble when viewed from the diametrically opposite position is 37.5 cm from the center of the sphere.
- Diameter of the glass sphere (D) = 50 cm
- Radius of the sphere (R) = D/2 = 25 cm
- Refractive index of glass (μ) = 1.5
- Apparent distance of the bubble from the surface (v1) = 10 cm
- The object (air bubble) is inside the sphere. The distance from the center of the sphere to the bubble can be calculated as:
u=R−v1=25 cm−10 cm=15 cm
- Here, u is taken as negative because the object is inside the sphere.
The lens formula is given by:
1f=1v−1u
where:
- f = focal length
- v = image distance (which we need to find)
- u = object distance
The focal length (f) for a spherical interface can be calculated using the formula:
f=R2(μ−1)
Substituting the values:
f=25 cm2(1.5−1)=25 cm2×0.5=25 cm1=25 cm
Now we can apply the lens formula:
125=1v−1−15
Rearranging gives:
1v=125−115
Finding a common denominator (75):
1v=375−575=−275
Thus:
v=−752=−37.5 cm
The negative sign indicates that the image is virtual and located on the same side as the object (inside the sphere). The apparent position of the bubble when viewed from the diametrically opposite position is 37.5 cm from the center of the sphere.
The apparent position of the bubble when viewed from the diametrically opposite position is 37.5 cm from the center of the sphere.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (ADVANCED) (MORE THAN ONE CORRECT TYPE QUESTIOS)
4 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (ADVANCED) (LINKED COMPREHENSION CORRECT TYPE QUESTIOS)
7 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE -I (LEVEL-II LECTURE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
4 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
10 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
Similar Questions
A small air bubble in a sphere of glass with radius 4 cm appears to be 1 cm from the surface when observed along a diameter. Find the true positino of the air bubble.
View SolutionA small object stuck on the surface of a glass sphere (n=1.5) is viewed from the diametrically opposite position. Find transverse magnification.
View Solution
Knowledge Check
A small air bubble in a glass block when seen from above appears to be raised because of :
Arefraction of lightBreflection of lightCreflection and refraction of lightDnone of the above
A small air bubble is situated at a distance of 3 cm from the center of a glass sphere of radius 9 cm. When viewed from the nearest side, the bubble appears to be at a distance of 5 cm from the surface. Its apparent distance when viewed from the farthest side is n×5 cm where n is?
View SolutionThere is a small air bubble inside a glass sphere (μ=1.5) of radius 10 cm. The bubble is 4.0cm below the surface and is viewd normally from the outside. Find the apparent dipth of the bubble.
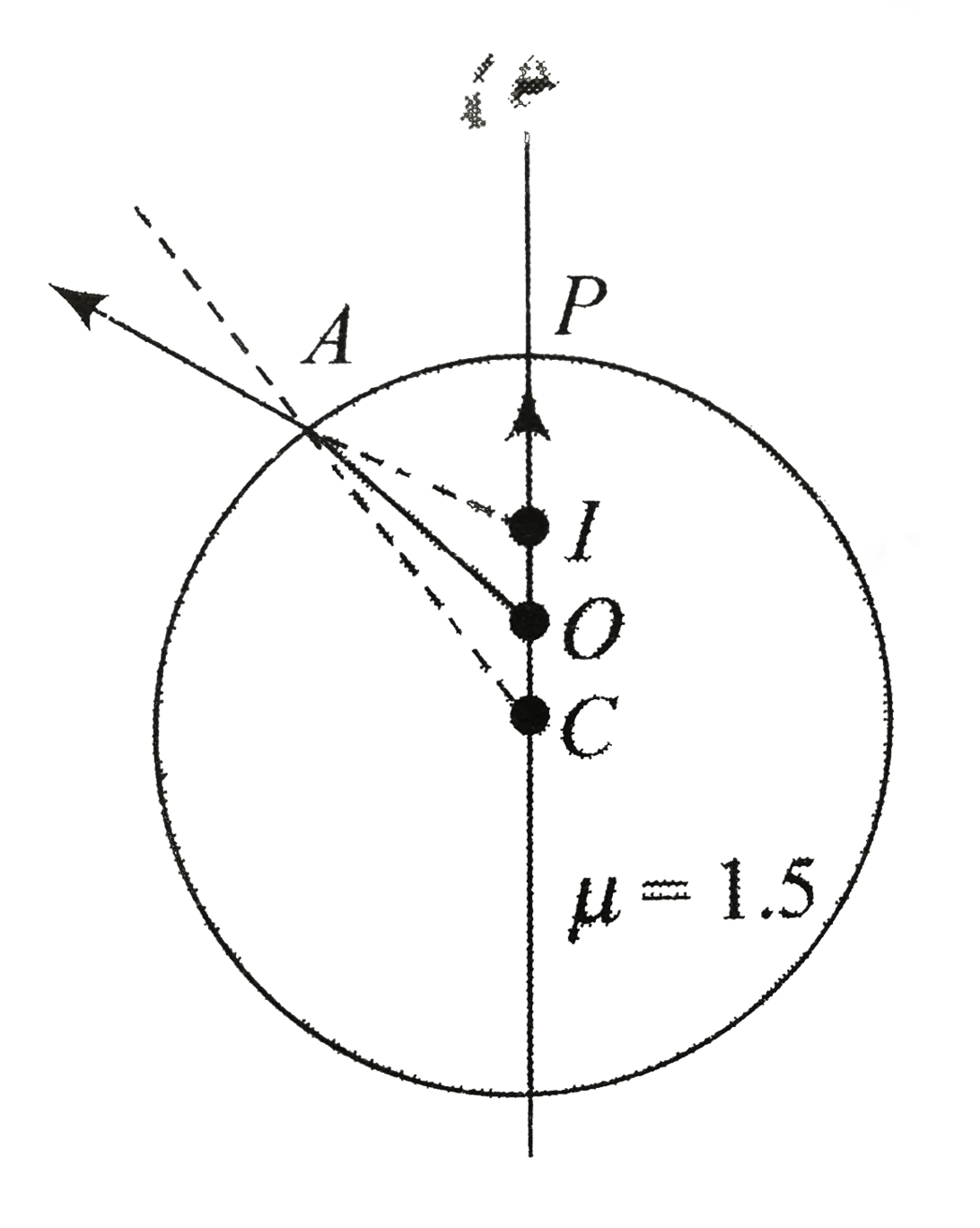
View SolutionA glass sphere (μ=1.5) of radius 20 cm has a small air bubble 4 cm below its centre. The sphere is viewed from outside and along a vertical line through the bubble. The apparent depth of the bubble below the surface of sphere is (in cm)
View SolutionA point object lies inside a transpatent solid sphere of radius 20cm and of refractive index n=2. When the object is viewed form air through the nearest surface it is seen at a distance 5cm form the surface. Find the apparent distance of object when its seen through the farthest curved surface
View SolutionA sphere made of transparent material of refractive index (μ=32 )and of radius 50 cm has a small air bubble 10 cm below the surface. The apparent depth of the bubble if viewed from outside normally is
View SolutionA glass sphere of (μ=1.5) with a radius of 15.0cm has a tiny air bubble 5cm above its centre. The sphere is viewed looking down along the extended radius containing the bubble. What is the apparent depth of the bubble below the surface of the sphere?
View SolutionA glass hemisphere of radius 10cm and μ=1.5 is silvered over its curved surface. There is an air bubble in the glass 5cms from the plane surface along the axis. Find the position of the images of this bubble seen by observer looking along the axis into the flat surface of the atmosphere.
View Solution
