A small ball rolls off the top landing of a staircase. It strikes the mid point of the first step and then mid point of the second step. The steps are smooth & identical in height & width. The coefficient of restitution between the ball & the first step is :
1
The correct Answer is:B
To solve the problem, we need to determine the coefficient of restitution (e) between the ball and the first step after the ball rolls off the top landing of the staircase and strikes the midpoint of the first step.
1. Understanding the Motion of the Ball:
- The ball rolls off the top landing of the staircase and falls vertically downwards to strike the first step.
- The height of each step is denoted as h.
2. Calculating the Time Taken to Fall:
- The time taken for the ball to fall a height h can be calculated using the second equation of motion:
t=√2hg
where g is the acceleration due to gravity.
3. Determining the Vertical Velocity Before Impact:
- The vertical velocity v of the ball just before it strikes the first step can be found using:
v=√2gh
4. Applying the Coefficient of Restitution:
- Let e be the coefficient of restitution. According to the definition, it relates the relative velocities before and after the collision:
e=Velocity after collisionVelocity before collision
- Before the collision, the vertical velocity is v (downward). After the collision, the velocity becomes ev (upward).
5. Using the Second Equation of Motion for the Ball's Motion:
- The ball travels a vertical distance of h after the collision. We can apply the second equation of motion again:
−h=ev⋅2t−12g(2t)2
- Substituting t from step 2:
−h=ev⋅2√2hg−2h
6. Substituting for v:
- Replace v with √2gh:
−h=e⋅√2gh⋅2√2hg−2h
- Simplifying gives:
−h=2e⋅2hg−2h
7. Rearranging the Equation:
- Rearranging the terms:
2h=2e⋅2hg+h
- Dividing through by h (assuming h≠0):
2=4e/g+1
8. Solving for e:
- Rearranging gives:
4e/g=1
e=g4
- Since we need the coefficient of restitution in terms of a numerical value, we can derive that:
e=34
Final Answer:
The coefficient of restitution between the ball and the first step is 34.
---
- The ball rolls off the top landing of the staircase and falls vertically downwards to strike the first step.
- The height of each step is denoted as h.
- The time taken for the ball to fall a height h can be calculated using the second equation of motion:
t=√2hg
where g is the acceleration due to gravity.
- The vertical velocity v of the ball just before it strikes the first step can be found using:
v=√2gh
- Let e be the coefficient of restitution. According to the definition, it relates the relative velocities before and after the collision:
e=Velocity after collisionVelocity before collision
- Before the collision, the vertical velocity is v (downward). After the collision, the velocity becomes ev (upward).
- The ball travels a vertical distance of h after the collision. We can apply the second equation of motion again:
−h=ev⋅2t−12g(2t)2
- Substituting t from step 2:
−h=ev⋅2√2hg−2h
- Replace v with √2gh:
−h=e⋅√2gh⋅2√2hg−2h
- Simplifying gives:
−h=2e⋅2hg−2h
- Rearranging the terms:
2h=2e⋅2hg+h
- Dividing through by h (assuming h≠0):
2=4e/g+1
- Rearranging gives:
4e/g=1
e=g4
- Since we need the coefficient of restitution in terms of a numerical value, we can derive that:
e=34
The coefficient of restitution between the ball and the first step is 34.
Topper's Solved these Questions
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Advance McQ
20 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - unsolved
110 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - Conceptual MCQ s single option correct
30 Videos
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.
83 Videos
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Unsolved Numerical Problem
81 Videos
Similar Questions
A staircase has each step of height h and width x. A ball strikes the centre point of a step with velocity v making an angle θ with the vertical. It rebounds and strikes the centre point of the next step. Once again it rebounds and hits the centre point of the next step and so on.
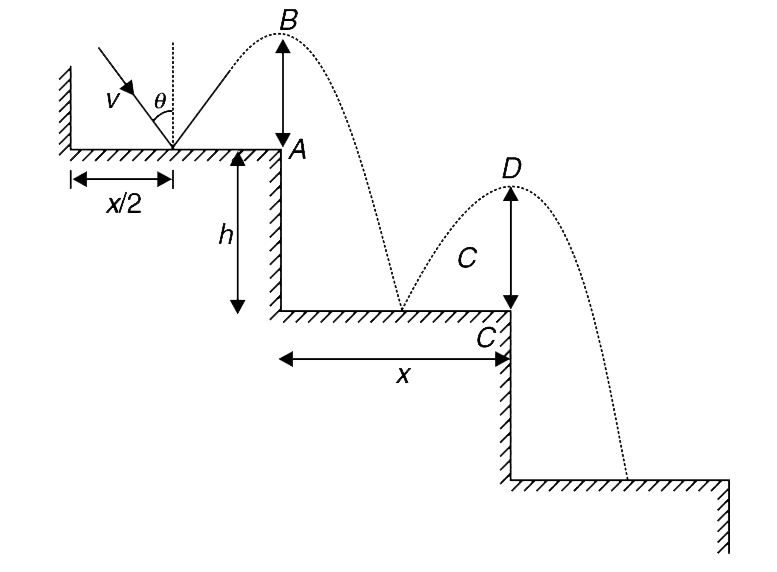
Assume that there is no friction between the ball and the steps and coefficient of restitution is e.
(a) Show that each time after hitting a step, the ball climbs to the same height (i.e., heights like AB and CD shown in figure are equal).
(b) Find h and x.
View SolutionA ball is thrown from a point at a distance c from a smooth vertical wall and after striking against the wall it returns to the point of projection. Take 'e' to be the coefficient of restitution between the ball and the wall. If α be the angle of projection with the horizontal and u the velocity of projection, then
u= ⎷{(1+e)gcesin2α} and time of flight
T= ⎷{2(1+e)ctanαeg}
View Solution
Knowledge Check
A ball released from a certain height strikes the ground after 2 second. After bouncing from the ground it rises to the highest point in 1 second. What is the coefficient of restitution?
A0.3B0.4C0.5D0.6A tennis ball bounces down a flight of stairs, striking each step in turn and rebounding to half to height of the step. The coefficient of restitution is
A1/2B1√2C(1√2)1/2D(1√2)1/4A ball is dropped on a horizontal surface from height h. If it rebounds upto h2 after first collision then coefficient of restitution between ball and surface is
A1√2B12C14D12+√2
A ball rolls off top of a staircase with a horizontal velocity ums−1. If the steps are h metre high and b mere wide, the ball will just hit the edge of nth step. Find the value of n.
View SolutionTwo identical balls are dropped from the same height onto a hard surface, the second ball being released exactly when the first ball bollides with the surface. If the first ball has made two more collisions by the time the second one collides. Then the coefficient of restitution between the ball and the surface satisfies :
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at some distance from the point of projection and returns on the same level. Coefficient of restitution between the ball and the wall is e, then time taken in path AB and maximum height attained by the ball is

View SolutionA ball rolls off the top of a stairway with a horizontal velocity u. If the height and width of steps are b and h respectively and the ball hits the edge of nth step, then n is equal to
View SolutionA ball is dropped on a horizontal surface from height h. If it rebounds upto height h2 after first collision then coefficient of restitution between ball and surface is
View Solution
