Question

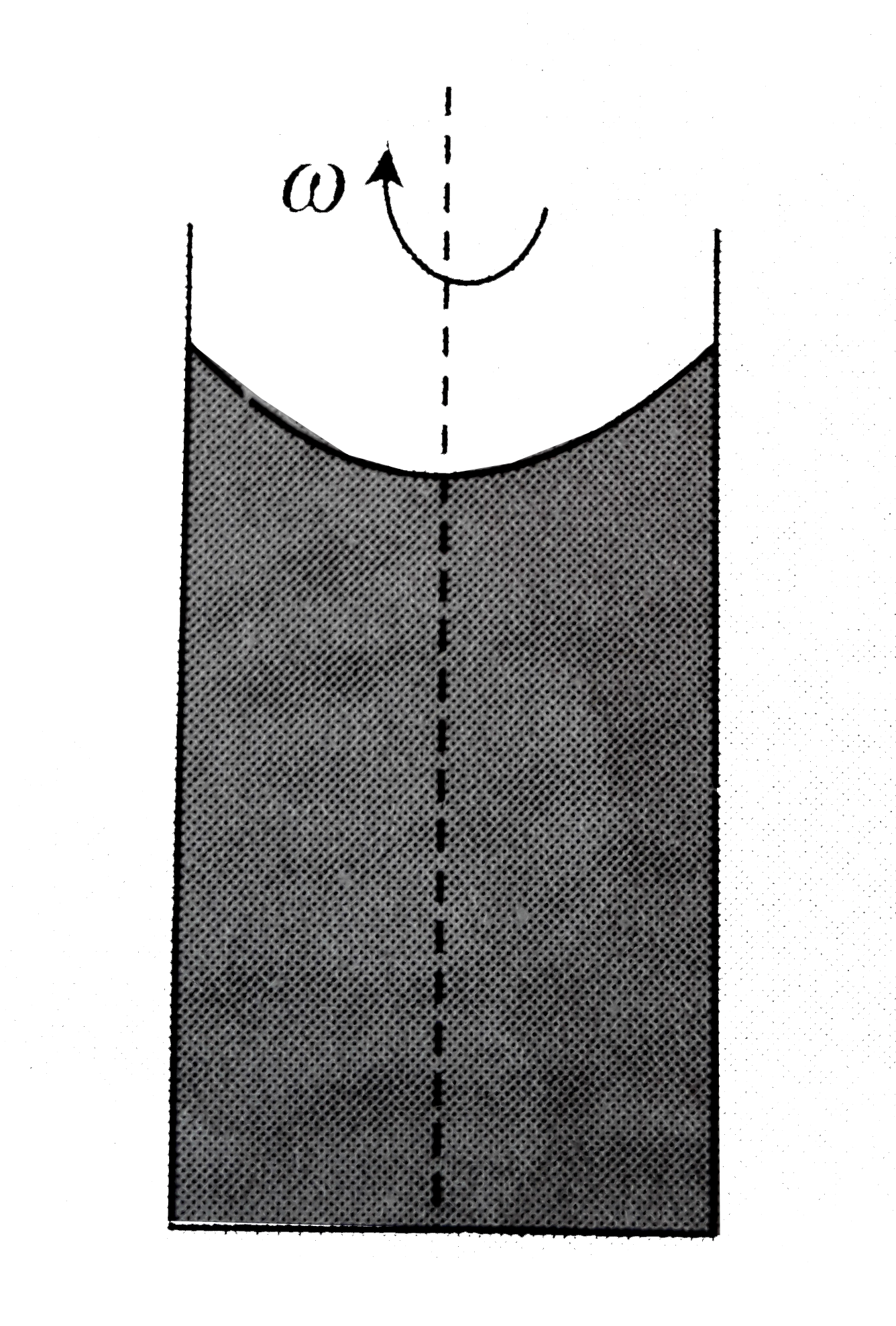
A small tank of cylindrical shape and of radius R is containing a liquid of density $\sigma .$ On rotating the vessel about its axis with angular velocity $\omega ,$ the liquid rises at the sides of vessel through height h. $P_1\text{and}{P}_2$ are pressure at sides and at the centre of tank respectively, then

Options

A. `P_(1) gt P_(2)`
B. `P_(1)=P_(2)`
C. `P_(2) gt P_(1)`
D. `h=(R^(2) omega^(2))/(2g)`

Answer

B::D

Explanation

To solve the problem, we need to analyze the situation of a cylindrical tank filled with liquid that is rotating about its axis. The key points we need to consider are the pressure at the sides of the tank (P1) and the pressure at the center of the tank (P2), as well as the height (h) to which the liquid rises at the sides.

1. Understanding the Setup:
- We have a cylindrical tank of radius \( R \) filled with a liquid of density \( \sigma \).
- The tank is rotating with an angular velocity \( \omega \).
- Due to the rotation, the liquid surface will not remain flat; instead, it will take the shape of a paraboloid, rising at the sides of the tank.

2. Defining the Height:
- Let \( h \) be the height to which the liquid rises at the sides of the tank.
- The height of the liquid at the center of the tank will be lower than at the sides.

3. Applying the Hydrostatic Pressure Equation:
- The pressure at a depth \( y \) in a liquid is given by the equation:
\( P = P_0 + \sigma g y \)
- Where \( P_0 \) is the atmospheric pressure, \( \sigma \) is the density of the liquid, \( g \) is the acceleration due to gravity, and \( y \) is the height of the liquid column above the point where pressure is being measured.

4. Pressure at the Center (P2):
- At the center of the tank (where \( x = 0 \)), the height of the liquid is \( y_{\text{min}} \).
- Therefore, the pressure at the center \( P_2 \) is:
\( P_2 = P_0 + \sigma g y_{\text{min}} \)

5. Pressure at the Side (P1):
- At the side of the tank (where \( x = R \)), the height of the liquid is \( y_{\text{max}} = y_{\text{min}} + h \).
- Thus, the pressure at the side \( P_1 \) is:
\( P_1 = P_0 + \sigma g (y_{\text{min}} + h) \)

6. Finding the Height (h):
- From the analysis of the forces acting on a fluid element in the rotating tank, we can derive that:
\( h = \frac{\omega^2 R^2}{2g} \)

7. Comparing Pressures (P1 and P2):
- Now, substituting \( h \) into the equations for \( P_1 \) and \( P_2 \):
\( P_1 = P_0 + \sigma g (y_{\text{min}} + \frac{\omega^2 R^2}{2g}) \)
\( P_2 = P_0 + \sigma g y_{\text{min}} \)
- Thus, we can see that:
\( P_1 = P_2 + \sigma g \frac{\omega^2 R^2}{2g} \)
- This shows that \( P_1 > P_2 \).

Conclusion:
- The final results are:
\( h = \frac{\omega^2 R^2}{2g} \)
\( P_1 > P_2 \)