A solid sphere of radius R made of a material of bulk modulus K is surrounded by a liquid in a cylindrical container. A massless pistion of area A floats on the surface of the liquid. When a mass M is placed on the piston to compress the liquid the fractional change in the radius of the sphere, δR/R, is .............
The correct Answer is:A, C
To solve the problem, we need to determine the fractional change in the radius of a solid sphere when a mass is placed on a piston that compresses the liquid surrounding the sphere. We will use the concept of bulk modulus and the relationship between pressure, volume change, and the radius of the sphere.
1. Understanding the Bulk Modulus:
The bulk modulus K is defined as the ratio of the change in pressure ΔP to the fractional change in volume ΔVV:
K=−ΔPΔVV
2. Change in Pressure:
When a mass M is placed on the piston, it exerts a force F=Mg on the liquid. The pressure change ΔP caused by this force over the area A of the piston is given by:
ΔP=FA=MgA
3. Volume Change of the Sphere:
The volume V of a sphere is given by:
V=43πR3
The change in volume ΔV due to a change in radius ΔR can be approximated using the derivative:
ΔV≈4πR2ΔR
4. Substituting into the Bulk Modulus Equation:
We can substitute ΔP and ΔV into the bulk modulus equation:
K=−ΔPΔVV=−MgA4πR2ΔR43πR3=−MgA⋅3R4ΔR
5. Rearranging for ΔR:
Rearranging the above equation gives:
ΔR=−3R⋅ΔP⋅A4K
Since we are interested in the fractional change ΔRR, we can express it as:
ΔRR=−3ΔP4K
6. Substituting ΔP:
Now substituting ΔP=MgA:
ΔRR=−3⋅MgA4K=−3Mg4AK
7. Final Result:
The negative sign indicates a decrease in radius, but we are interested in the magnitude:
ΔRR=3Mg4AK
Conclusion:
The fractional change in the radius of the sphere is:
ΔRR=3Mg4AK
The bulk modulus K is defined as the ratio of the change in pressure ΔP to the fractional change in volume ΔVV:
K=−ΔPΔVV
When a mass M is placed on the piston, it exerts a force F=Mg on the liquid. The pressure change ΔP caused by this force over the area A of the piston is given by:
ΔP=FA=MgA
The volume V of a sphere is given by:
V=43πR3
The change in volume ΔV due to a change in radius ΔR can be approximated using the derivative:
ΔV≈4πR2ΔR
We can substitute ΔP and ΔV into the bulk modulus equation:
K=−ΔPΔVV=−MgA4πR2ΔR43πR3=−MgA⋅3R4ΔR
Rearranging the above equation gives:
ΔR=−3R⋅ΔP⋅A4K
Since we are interested in the fractional change ΔRR, we can express it as:
ΔRR=−3ΔP4K
Now substituting ΔP=MgA:
ΔRR=−3⋅MgA4K=−3Mg4AK
The negative sign indicates a decrease in radius, but we are interested in the magnitude:
ΔRR=3Mg4AK
The fractional change in the radius of the sphere is:
ΔRR=3Mg4AK
Topper's Solved these Questions
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MECHANICAL PROPERTIES OF MATTER AND FLUIDSEXERCISE - Subjective Problems1videos
MECHANICAL PROPERTIES OF MATTER AND FLUIDS
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - LAWS OF MOTIONEXERCISE - JEE Main And Advanced79videos
LAWS OF MOTION
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOMENTUM & IMPULSEEXERCISE - JEE Main And Advanced30videos
MOMENTUM & IMPULSE
Similar Questions
A solid sphere of radius R made of a material of bulk modulus B is surrounded by a liquid in a cylindrical container. A massless piston of area A (the area of container is also A) floats on the surface of the liquid. When a mass M is placed on the piston to compress the liquid , fractional change in radius of the sphere is MgαAB. Find the value of α.
View SolutionA rubber ball of bulk modulus B is taken to a depth h of a liquid of density ρ. Find the fractional change in the radius of the ball.
View Solution
Knowledge Check
- Question 1 - Select One
A solid sphere of radius R made of a material of bulk modulus K is surrounded by a liquid in a cylindrical container. A massless pistion of area A floats on the surface of the liquid. When a mass M is placed on the piston to compress the liquid the fractional change in the radius of the sphere, δR/R, is .............
Amg3KaBmgKaCKamgDKa3mg - Question 2 - Select One
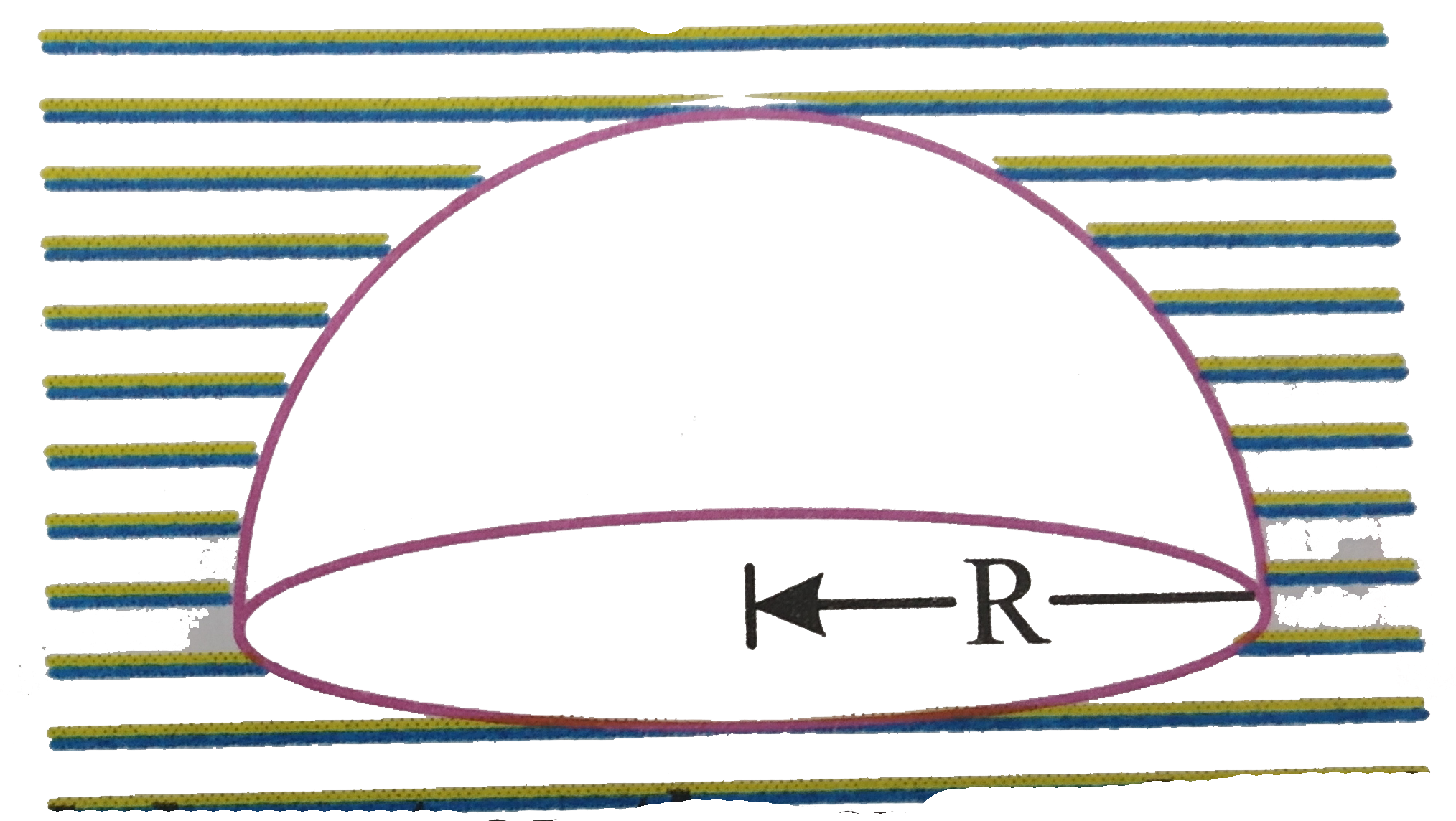
A solid hemisphere of radius R is made to just sink in a liquid of density ρ find theAπR3ρg3BπR3ρg2C0DπR3ρg - Question 3 - Select One
A sphere of radius R and made of material of relative density σ has a concentric cavit of radius r. It just floats when placed in a tank full of water. The value of the ratio R/r will be
A(σσ−1)1/3B(σ−1σ)1/3C(σ+1σ)1/3D(σ−1σ+1)1/3
Three solid sphere of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionFrom a solid sphere of mass M and radius R, a solid sphere of radius R/2 is removed as shown. Find gravitational force on mass m as shown
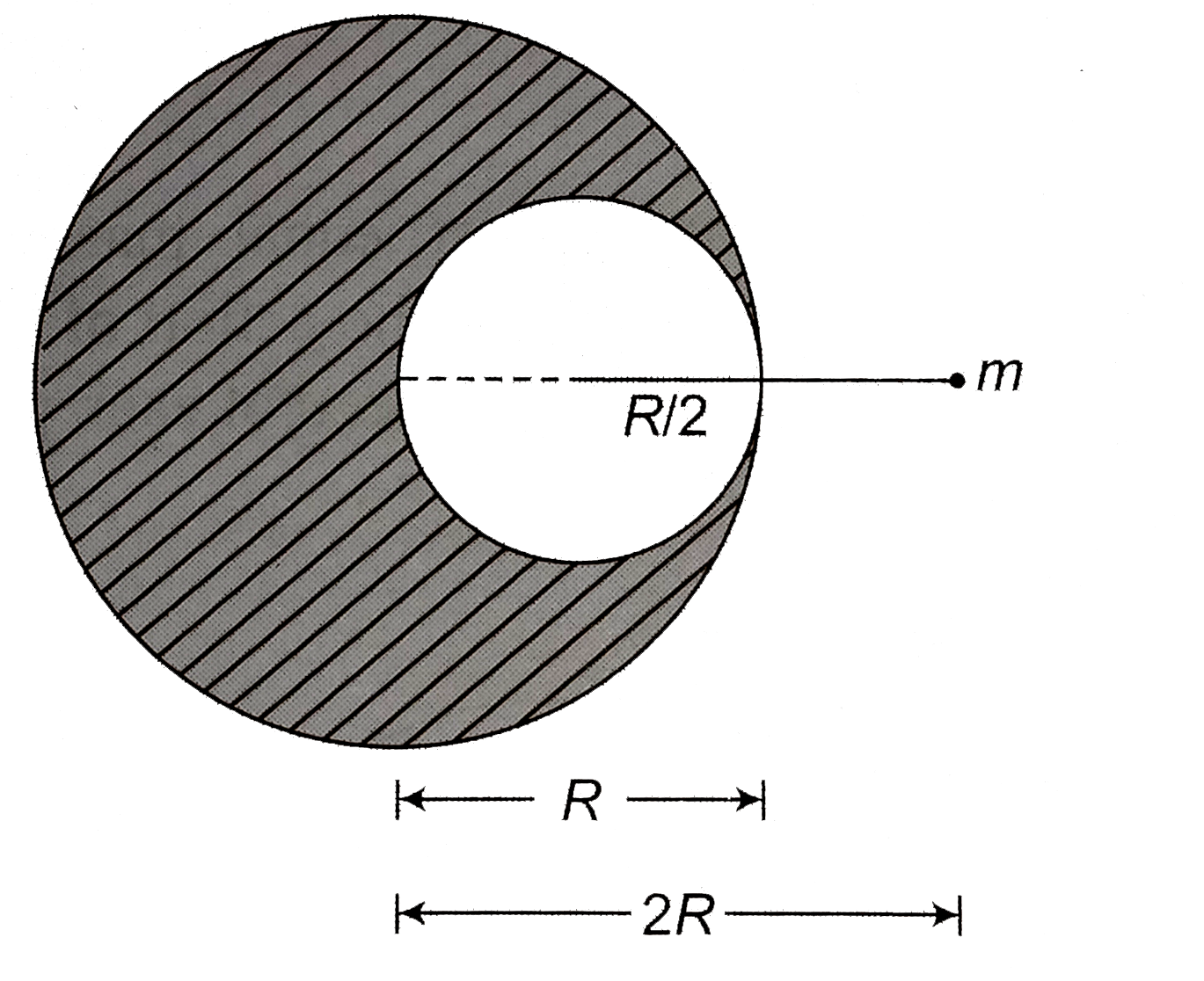 View Solution
View SolutionThree solid spheres of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionA uniform solid sphere of mass m and radius r is suspended symmetrically by a uniform thin spherical shell of radius 2r and mass m.
View SolutionA cylinder of radius R made of material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius R and outer radius 3R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. What is the effective thermal conductivity of the system ?
View Solution

