A source and a detector move away from each other, each with a speed of 10ms−1 with respect to the ground with no wind. If the detector detects a frequency 1950 Hz of the sound coming from the source, what is the original frequency of the source? Speed of sound in air =340ms−1.
To find the original frequency of the sound emitted by the source, we can use the formula for the apparent frequency when both the source and the observer are moving away from each other.
1. Identify Given Values:
- Apparent frequency (μ′) = 1950 Hz
- Speed of sound in air (V) = 340 m/s
- Speed of source (Us) = 10 m/s (moving away)
- Speed of observer (Uob) = 10 m/s (moving away)
2. Understand the Formula:
The formula for the apparent frequency when the source and observer are moving away from each other is given by:
μ′=μ(V−UobV+Us)
Rearranging this formula to solve for the original frequency (μ):
μ=μ′(V+UsV−Uob)
3. Substitute the Values:
Substitute the known values into the rearranged formula:
μ=1950(340+10340−10)
4. Calculate the Numerator and Denominator:
- Numerator: 340+10=350
- Denominator: 340−10=330
5. Calculate the Fraction:
Now calculate the fraction:
350330=3533
6. Calculate the Original Frequency:
Now, multiply this fraction by the apparent frequency:
μ=1950×3533
7. Perform the Final Calculation:
μ≈1950×1.0606≈2070 Hz
Final Answer:
The original frequency of the source is approximately 2070 Hz.
---
- Apparent frequency (μ′) = 1950 Hz
- Speed of sound in air (V) = 340 m/s
- Speed of source (Us) = 10 m/s (moving away)
- Speed of observer (Uob) = 10 m/s (moving away)
The formula for the apparent frequency when the source and observer are moving away from each other is given by:
μ′=μ(V−UobV+Us)
Rearranging this formula to solve for the original frequency (μ):
μ=μ′(V+UsV−Uob)
Substitute the known values into the rearranged formula:
μ=1950(340+10340−10)
- Numerator: 340+10=350
- Denominator: 340−10=330
Now calculate the fraction:
350330=3533
Now, multiply this fraction by the apparent frequency:
μ=1950×3533
μ≈1950×1.0606≈2070 Hz
The original frequency of the source is approximately 2070 Hz.
Topper's Solved these Questions
Similar Questions
A source and a detector move away from each other, each with a speed of 10 m/s with respect to ground with no wind. If the detector detects a frequency 1650 Hz of the sound coming from the source, what is the original frequency of the source? (speed of sound = 340 m/s
View SolutionTwo cars A and B are moving away from each other in opposite directions. Both the cars are moving with a speed of 20ms−1 with respect to the ground. If an observer in car A detects a frequency 2000 Hz of the sound coming from car B, what is the natural frequency of the sound source in car B? (Speed of sound in air =340ms−1)
View SolutionAn observer travelling with a constant velocity of 16.5ms−1, passes closes to a stationary source of sound and notices that there is a change of frequency of 50 Hz as he passes the source. Calculate the frequency of the source. (Speed of sound in air = 330 ms−1).
View SolutionA siren emitting a sound of frequency 2000 Hz moves away from you towards a cliff at a speed of 8 m/s.
(a) What is the frequency of the sound you hear coming directly from the siren.
(b) What is the frequency of sound you hear reflected off the cliff. Speed of sound in air is 330ms.View SolutionOn the same path, the source and observer are moving such a way that the distance between these two increases with the time. The speeds of source and observer are same and equal to 10ms−1 with respect to the ground while no wind in blowing. The apparent frequency received by observer is 1950Hz then the original frequency must be (the speed of sound in present medium is 340m/s
View SolutionThe figure shows the location of a source and detector at time t = 0. The source and detector are moving with velocities vs=5ˆims−1andvD=10ˆjms−1 respectively . The frequency of signals received by the detector at the moment when the source cros the origin is (the frequency of the source is 100 Hz . Velocity bof sound 330ms−1 .)
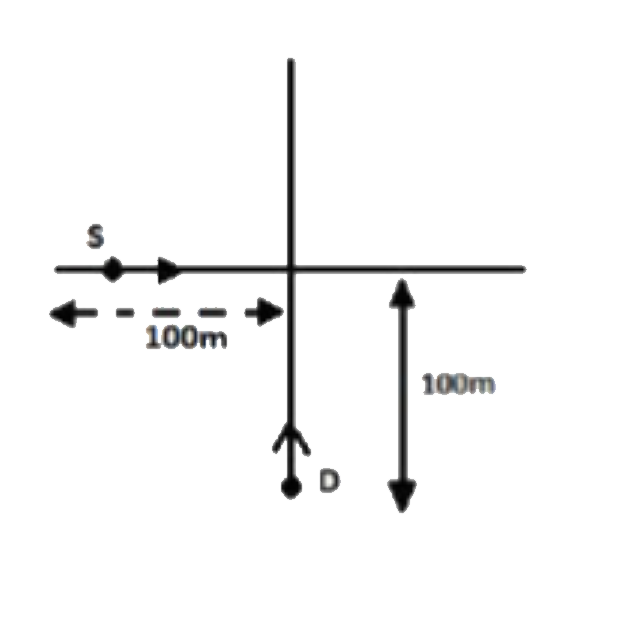 View Solution
View SolutionTwo sound waves, originating from the same source, travel along different paths in air and then meet at a point. If the source vibrates at a frequency of 1.0 kHz and one path is 83 cm longer than the other, what will be the nature of interference? The speed of sound in air is 332ms−1.
View SolutionA sound detector is placed on a railway platform. A train, approaching the platform at a speed of 36kmh−1, sounds its whistle. The detector detects 12.0 kHz is the most dominant frequency in the whistle. If the train stops at the platform and sounds the whistle, what would be the most dominant frequency detected? The speed of sound in air is 340ms1.
View SolutionA source of sound and an observer are approaching each other with the same speed, which is equal to (110) times the speed of sound. The apparent relative change in frequency of source is
View SolutionA stationary source emits the sound of frequency f0=492 Hz. The sound is reflected by a large car approaching the source with a speed of 2ms−1. The reflected signal is received by the source and superposed with the original. What will be the beat frequency of the resulting signal in Hz? (Given that the speed of sound in air is 330ms−1 and the car reflects the sound at the frequency it has received).
View Solution

