A source of sound is travelling with a velocity of 30ms towards a stationary observer. If actual frequency of source is 1000 Hz and the wind is blowing with velocity 20ms in a direction at 60∘C with the direction of motion of source, then the apparent frequency heard by observer is (speed of sound is 340ms)
1011 Hz
1000 Hz
1094 Hz
1086 Hz
The correct Answer is:C
To find the apparent frequency heard by the observer when a source of sound is moving towards them, we can use the Doppler effect formula. Let's break down the solution step by step.
1. Identify the Given Values:
- Actual frequency of the source, N=1000Hz
- Velocity of the source, Vs=30m/s (towards the observer)
- Velocity of the observer, V0=0m/s (stationary)
- Speed of sound in still air, V=340m/s
- Velocity of wind, Vw=20m/s (at an angle of 60∘ with the direction of motion of the source)
2. Calculate the Effective Speed of Sound:
Since the wind affects the speed of sound, we need to calculate the effective speed of sound. The wind's contribution to the speed of sound can be calculated using the cosine of the angle:
Veffective=V+Vw⋅cos(60∘)
Veffective=340+20⋅12=340+10=350m/s
3. Apply the Doppler Effect Formula:
The formula for the apparent frequency N′ when the source is moving towards a stationary observer is:
N′=N⋅V+V0V−Vs
Substituting the values we have:
N′=1000⋅350+0350−30
N′=1000⋅350320
4. Calculate the Apparent Frequency:
Now, simplify the fraction:
N′=1000⋅1.09375=1093.75Hz
Rounding to the nearest whole number gives:
N′≈1094Hz
5. Final Answer:
The apparent frequency heard by the observer is approximately 1094Hz.
Summary of the Steps:
- Identify the given values.
- Calculate the effective speed of sound considering the wind.
- Apply the Doppler effect formula.
- Simplify to find the apparent frequency.
- Actual frequency of the source, N=1000Hz
- Velocity of the source, Vs=30m/s (towards the observer)
- Velocity of the observer, V0=0m/s (stationary)
- Speed of sound in still air, V=340m/s
- Velocity of wind, Vw=20m/s (at an angle of 60∘ with the direction of motion of the source)
Since the wind affects the speed of sound, we need to calculate the effective speed of sound. The wind's contribution to the speed of sound can be calculated using the cosine of the angle:
Veffective=V+Vw⋅cos(60∘)
Veffective=340+20⋅12=340+10=350m/s
The formula for the apparent frequency N′ when the source is moving towards a stationary observer is:
N′=N⋅V+V0V−Vs
Substituting the values we have:
N′=1000⋅350+0350−30
N′=1000⋅350320
Now, simplify the fraction:
N′=1000⋅1.09375=1093.75Hz
Rounding to the nearest whole number gives:
N′≈1094Hz
The apparent frequency heard by the observer is approximately 1094Hz.
- Identify the given values.
- Calculate the effective speed of sound considering the wind.
- Apply the Doppler effect formula.
- Simplify to find the apparent frequency.
Topper's Solved these Questions
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Multiple Correct
44 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Assertion-Reasoning
24 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Subjective
56 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
Similar Questions
A source of sound S is moving with a velocity of 50m/s towards a stationary observer. The observer measures the frequency of the source as 1000 Hz. What will be the apparent frequency of the source as 1000 Hz. What will be the apparent frequency of the source when it is moving away from the observer after crossing him? The velocity of the sound in the medium is 350m/s
View SolutionA source of sound S is moving with a velocity of 50m/s towards a stationary observer. The observer measures the frequency of the source as 1000 Hz. What will be the apparent frequency of the source as 1000 Hz. What will be the apparent frequency of the source when it is moving away from the observer after crossing him? The velocity of the sound in the medium is 350m/s
View SolutionTwo tuning forks A and B lying on opposite sides of observer 'O' and of natural frequency 80Hz move with velocity 10m/s relative to stationary observer O. Fork A moves away from the observer while the fork B moves towards him. A wind with a speed 10m/s is blowing in the direction of motion of fork A. Find the beat frequency measured by the observer in Hz. [Take speed of sound in air as 340m/s].
View SolutionA person going away from a factory on his scooter at a speed of 36 km/hr listens to the siren of the factory. If the actual frequency of the siren is 700 Hz and a wind is blowing along the direction of the scooter at 36 km//hr, find the observed frequency heard by the person. (Given speed of sound =340m/s)
View SolutionA source of sound is travelling towards a stationary observer. The frequency of sound heard by the observer is 25% more that the actual frequency. If the speed of sound is v, that of the source is
View SolutionA sound source moves with a speed of 80m/s relative to still air toward a stationary listener. The frequency of sound is 200HZ and speed of sound in air is 340m/s. (a) Find the wavelength of the sound between the source and the listener. (b) Find the frequency heard by the listener.
View SolutionAt t=0 , observer and source are at same place. Now the source is projected with velocity 60√2m/s at 45∘ . Natural frequency of source is 1000 Hz.find the frequency heard by the observer at t=2s. Take speed of sound = 340 m/s
View SolutionTwo trains A and B are moving in the same direction with velocities 30 m/s and 10 m/s respectively, B is behind from A, blows a horn of frequency 450 Hz. Then the apparent frequency heard by B is (The velocity of sound is 330 m/s) :-
View SolutionA source is moving with constant speed υs=20m/s towards a stationary observer due east of the source. Wind is blowing at the speed of 20m/s at 60∘ north of east. The source has frequency 500HZ. Speed of sound =300m/s. The frequency resgistered by the observer is approximately
View Solution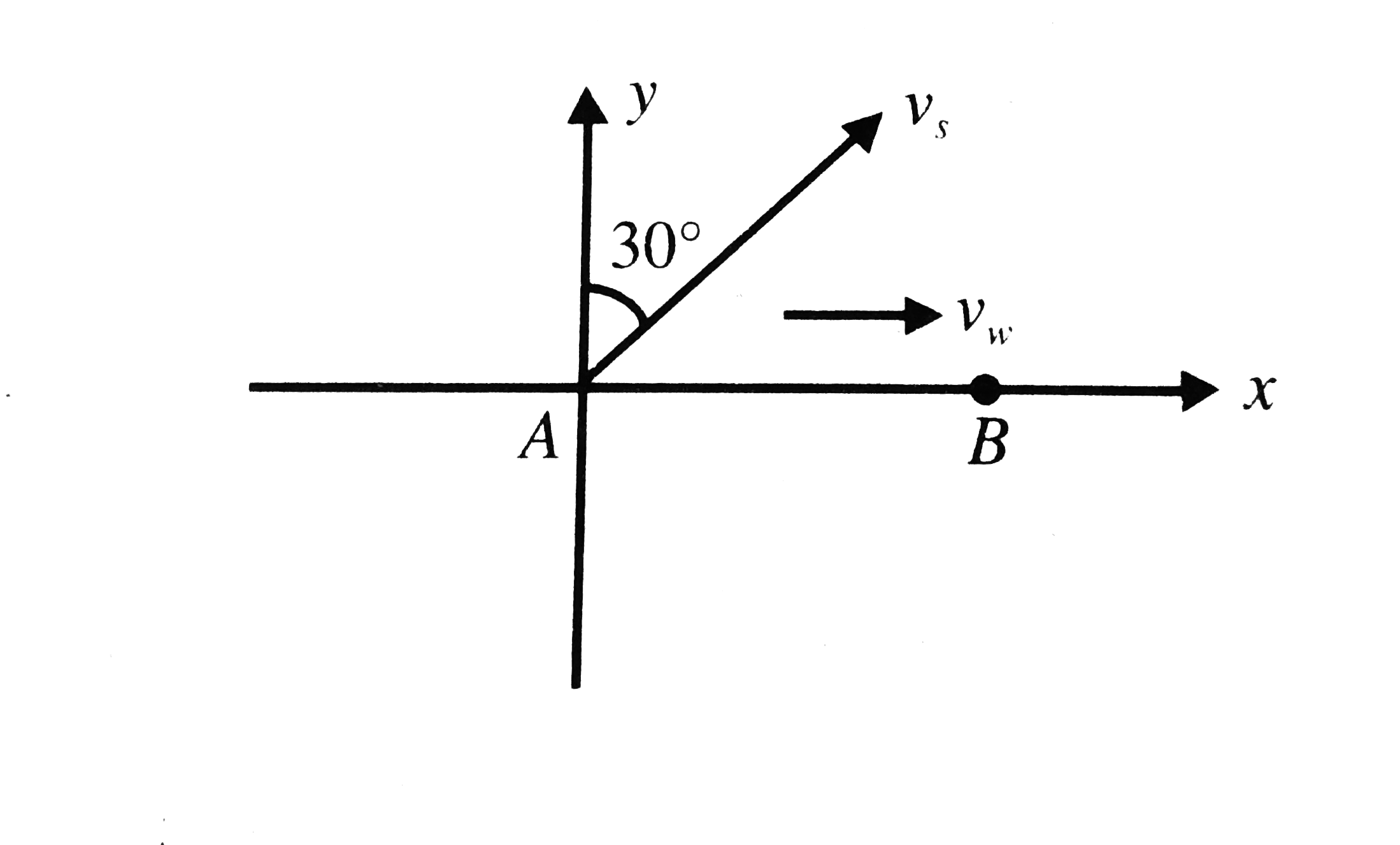
In the figure shown, a source of sound of frequency 510 Hz moves with constant velocity vS=20ms in the direction shown. The wind is blowing at a constant velocity vW=20ms towards an observer who is at rest at point B. corresponding to the sound emitted by the source at initial position A, the frequency detected by the observer is equal to (speed of sound relative to air is 330ms)
View Solution
