A standard car developes 40 H.P. Find the maximum speed the car can attain agains a resistance of 20kg wt. due to air and friction. Gvien efficiency of the engin is 25%.1H.P.=746W and g=10ms−2.
Here,
P=40H.P.=40×746watt
v=?F=20kgwt.=20×10N
η=25%,g=10m/s2
As η=output powerinput power
∴ output power = force × velocity
∴velocity=output powerforce
v=746020×10=37.3m/s
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Conceptual Problems
27 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Very Short Anwer Question.
54 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Curiosity questions
2 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Find the maximum speed at which a car can take turn round a curve of 30 cm radius on a level road if the coefficient of friction between the tyres and the road is 0.4. Take g=10ms−2.
View SolutionFind the maximum speed at which a car can turn round a curve of 30m radius on a level road if coefficient of friction between the tyress and road is 0.4.Takeg=10m/s2.
View Solution
Knowledge Check
A box of mass 2 kg is placed on the roof of a car. The box would remain stationary until the car attains a maximum acceleration. Coefficient of static friction between the box and the roof of the car is 0.2 and 1g=10 ms−2.
The maximum acceleration of the car, for the box to remain stationary, isA8 ms−2B6 ms−2C4 ms−2D2 ms−2A box of mass 2 kg is placed on the roof of a car. The box would remain stationary untill the car attains a maximum acceleration. Coefficient of static friction between the box and the roof of the car is 0.2 and g = 10ms−2. This maximum acceleration of the car, for the box to remain stationary, is
A8ms−2B6ms−2C4ms−2D2ms−2What is the maximum speed at which a car can turn round a curve of 30 m radius on a level road if the coefficient of friction between the types and the road is 0.4 .(Given, acceleration due to gravity =10ms−2
A12ms−1B10ms−1C11ms−1D15ms−1
The power of a car is 20 hp . The car is facing resistance of 10 kg wt . Due to air and friction . Calculate the maximum speed attained by the car against this resistance .
View SolutionFind the power of an engine which can maintain speed of 50ms−1 for a train of mass 3×105kg on a rough line. The coefficient of friction is 0.05. Take g=10ms−2
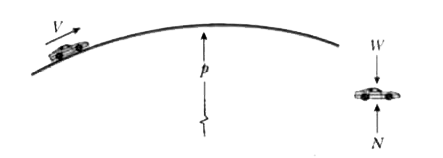
View SolutionIf the crest of the hill has a radius of curvature p determine the maximum constnat speed at which the car can travel over it without leaving the surface of the road. Neglect the size of the car in the calculation. The car has mass m. Given p=40m, m=1700kg, g=10m/s2
View SolutionA truck of amss 10 ton is movng up an incline of 1 in 50 with a uniform velocity of 72 km/h. If the resistance opposing the motion of the truck due to friction is 5 kg per ton, the power of the engine is (g=10m/s2)
View SolutionThe maximum speed (in ms−1 ) with which a car driver can traverse on a horizontal unbanked curve of radius 80 m with coefficient of friction 0.5 without skidding is : (g = 10 m/s2)
View Solution
