A steel wire of original length 1m and cross-sectional area 4.00mm2 is clamped at the two ends so that it lies horizontally and without tension. If a load of 2.16kg is suspended from the middle point of the wire, what would be its vertical depression ?
Y of the steel =2.0×1011Nm−2. Take g=10ms−2
The correct Answer is:A, C
To find the vertical depression of the steel wire when a load is suspended from its middle point, we can follow these steps:
Step 1: Identify the given values
- Original length of the wire, L=1m
- Cross-sectional area, A=4.00mm2=4.00×10−6m2
- Mass of the load, m=2.16kg
- Young's modulus of steel, Y=2.0×1011N/m2
- Acceleration due to gravity, g=10m/s2
Step 2: Calculate the force exerted by the load
The force F exerted by the load can be calculated using:
F=m⋅g
Substituting the values:
F=2.16kg×10m/s2=21.6N
Step 3: Set up the equation for depression
When the load is applied, it creates a tension in the wire. The relationship between stress, strain, and Young's modulus is given by:
Y=StressStrain=F/AΔL/L
Where:
- ΔL is the change in length (depression),
- L is the original length.
Rearranging gives:
ΔL=F⋅LY⋅A
Step 4: Substitute the values into the equation
Now, substituting the known values:
ΔL=21.6N⋅1m(2.0×1011N/m2)⋅(4.00×10−6m2)
Step 5: Calculate the denominator
Calculating the denominator:
Y⋅A=2.0×1011N/m2⋅4.00×10−6m2=800N
Step 6: Calculate the depression
Now substituting back into the equation for ΔL:
ΔL=21.6N800N=0.027m=2.7cm
Step 7: Conclusion
Thus, the vertical depression of the steel wire is approximately:
ΔL≈2.7cm
- Original length of the wire, L=1m
- Cross-sectional area, A=4.00mm2=4.00×10−6m2
- Mass of the load, m=2.16kg
- Young's modulus of steel, Y=2.0×1011N/m2
- Acceleration due to gravity, g=10m/s2
The force F exerted by the load can be calculated using:
F=m⋅g
Substituting the values:
F=2.16kg×10m/s2=21.6N
When the load is applied, it creates a tension in the wire. The relationship between stress, strain, and Young's modulus is given by:
Y=StressStrain=F/AΔL/L
Where:
- ΔL is the change in length (depression),
- L is the original length.
ΔL=F⋅LY⋅A
Now, substituting the known values:
ΔL=21.6N⋅1m(2.0×1011N/m2)⋅(4.00×10−6m2)
Calculating the denominator:
Y⋅A=2.0×1011N/m2⋅4.00×10−6m2=800N
Now substituting back into the equation for ΔL:
ΔL=21.6N800N=0.027m=2.7cm
Thus, the vertical depression of the steel wire is approximately:
ΔL≈2.7cm
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Question For short Anser18videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-129videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Worked out Example17videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer15videos
SIMPLE HARMONIC MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions133videos
SOUND WAVES
Similar Questions
A mild steel wire of length 1.0 m and cross-sectional are 0.5×10−2cm2 is streached, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid point of the wire, calculate the depression at the mid point.
g=10ms−2,Y=20×1011Nm−2.
 View Solution
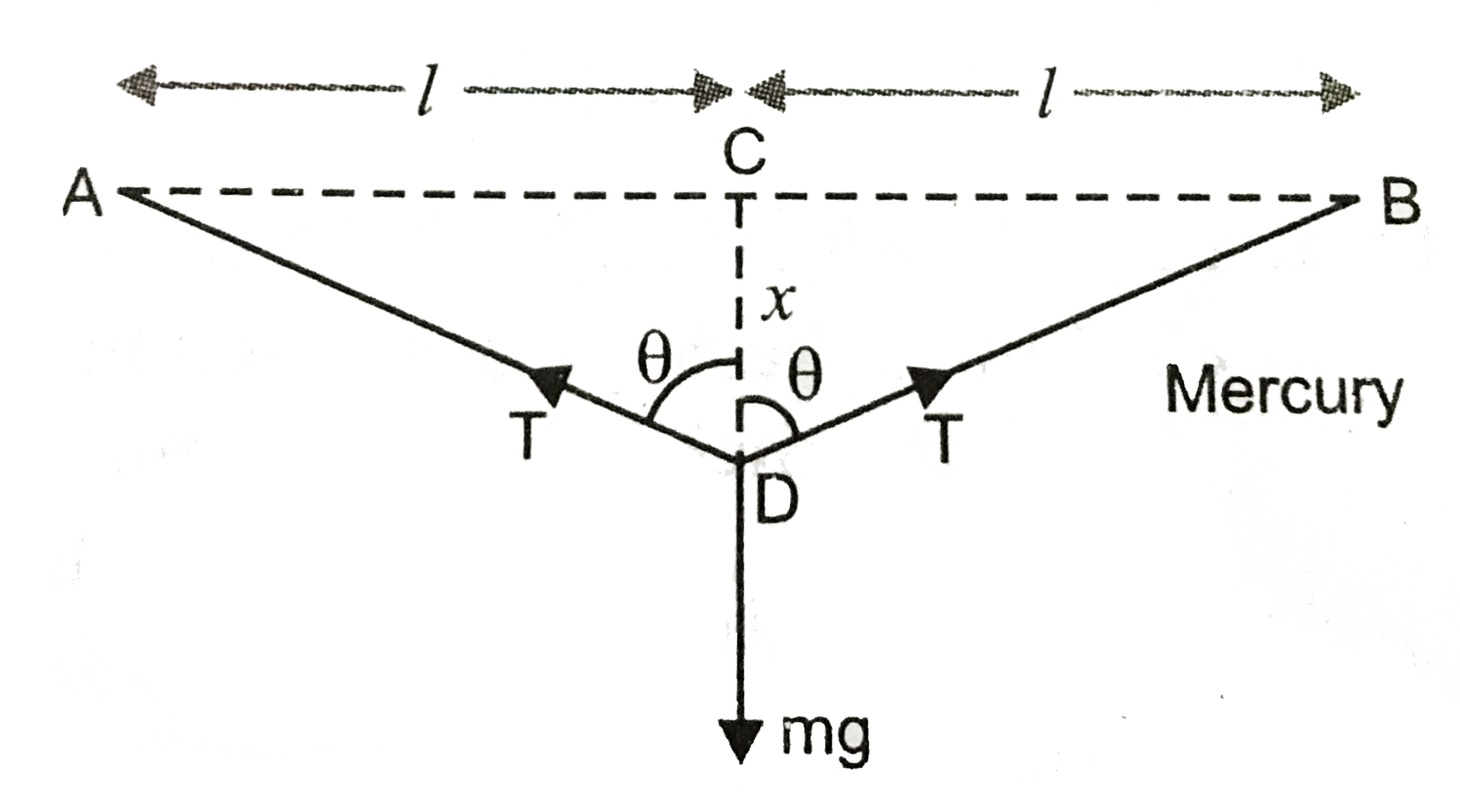
View SolutionA steel wire of length 2L and cross-section area A is stretched, wetl within elastic limit horizontally between two pillars, a mass m is suspended from the mid-point of wire such that depression at the middle is x. Strain in the wire is proportional to
View SolutionOne end of a wire 2m long and 0.2 cm^2 in cross section is fixed in a ceiling and a load of 4.8 kg is attached to the free end. Find the extension of the wire. Young modulus of steel =2.0×1011Nm−2. Take g=10ms−2.
View SolutionA steel wire and a copper wire of equal length and equal cross sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of a. The stresses developed in the two wire and b. the strains developed. Y of steel =2×1011Nm−2. Y of copper =1.3×1011Nm−2.
View SolutionA steel wire of negligible mass, length 2I, cross sectional area 'A' and Young's modulus 'Y' is held between two rigid walls. A small body of mass 'm' is suspended from its middle point 'O' as shown. The vertical descent of the middle point 'O' of the wire is
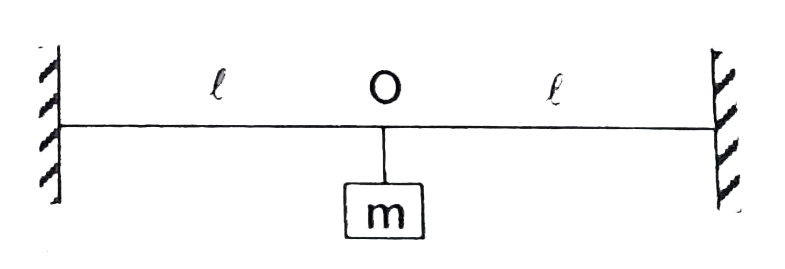 View Solution
View SolutionA wire of area of cross section 3.0mm2 and natural length 50 cm is fixed at one end and a mas of 2.1 kg is hung from the other end. Find the elastic potential energy stored in the wire in steady state. Young modulus of the material of the wire =1.9×1011Nm−2.Takeg=10ms−2
View SolutionA wire of length 50cm and cross sectional area of 1 sq. mm is extended by 1mm. The required work will be (Y=2×1010Nm−2)
View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of
copper/steel
(a) the stresses developed . In the two wires,
(b) the strains developed. (Y of steel =2×1011N/m2)View SolutionA brass of length 2m and cross-sectional area 2.0cm2 is attached end to end to a steel rod of length and cross-sectional area 1.0cm2. The compound rod is subjected to equal and oppsite pulls of magnitude 5×104N at its ends. If the elongations of the two rods are equal, the length of the steel rod (L) is
{YBrass=1.0×1011N/m2 and Ysteel=2.0×1011N/m2View SolutionWhen a load of 10 kg is suspended on a metallic wire, its length increase by 2 mm. The force constant of the wire is
View Solution
