A stone of m ass m tied to the end of a string revolves in a vertical circle of radius R. The net force at the lowest and highest points of the circle d irected vertically dow nw ards are : [Choose the correct alternative]
Lowest Point
(a) mg - T1
(b) mg+T1
(c) mg+T1−(mv21)/R
(d) mg−T1−(mv21)/R Highest Point mg+T2
mg - T2
mg−T2+(mv21)/R
mg+T2+mv21R
T1 and denote the tension and speed at the lowest point. T2 and v2 denote corresponding values at the highest point.Solution in Gujrati
When stone is tield at end of string and whirled in circular path in vertical plane let H be highest point and L be lowest point
At point L :
Tension T1 toward centre and weigth mg is in downward direction
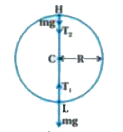
Resultant force acting on stone is in downward direction
Resultant force =mg = T1
At point H : Here tensiion T2 and weight mg
Hence option ( A) is correct .
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - LAW OF MOTIONEXERCISE - SECTION -B (NUMERICAL FROM DARPAN BASED ON TEXTBOOK)8videos
LAW OF MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - LAW OF MOTIONEXERCISE - SECTION-C(OBJECTIVE QUESTIONS) (VSQS)55videos
LAW OF MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - LAW OF MOTIONEXERCISE - SECTION -B (NUMERICAL FROM TEXTUAL EXERCISE)23videos
LAW OF MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - GRAVITATIONEXERCISE - QUESTIONS PAPER Section - D1videos
GRAVITATION
- BOOK - KUMAR PRAKASHANCHAPTER - MACHANICAL PROPERTIES OF SOLIDS EXERCISE - QUESTION PAPER11videos
MACHANICAL PROPERTIES OF SOLIDS
Similar Questions
A soldier fires bullets, each of mass 50 g, from his automatic rifle with a velocity of 1000 m/s. If he can bear a maximum force of 200 N on his shoulder, find the number of bullets which he can fire in a second.
03:08View SolutionArea of F → t graph gives which physical quantity ?
02:15View SolutionGive the magnitude and direction of the net force acting on (a) a drop of rain falling down with a constant speed, (b) a cork of mass 10 g floating on water, (c) a kite skillfully held stationary in the sky, (d) a car moving with a constant velocity of 30 km/h on a rough road, (e) a high-speed electron in space far from all material objects, and free of electric and magnetic fields.
01:13View SolutionA spaceship is stationed on Mars. How much energy must be expended on the spaceship to launch it out of the solar system ? Mass of the spaceship = 1000 kg, mass of the sun =2×1030kg, mass of mars =6.4×1023kg, radius of mars = 3395 km, radius of the orbit of mars =2.28×108km,G=6.67×10−11Nm2kg2
View SolutionWhat is called brittle material ?
06:15View SolutionA main revolves a stone of mass m tied to the end of a string in a circle of radius R The net force at the lowest and beight point of the circle directed vertical downward are Here T_(1),T_(2) and (v_(1),v_(2)) denote the tension in the string (and the speed of the stone) at the lowest and highest points, respectively.
View SolutionA stone of mass m tied to one end of a string is revolved in a vertical circle of radius R the net forces at the lowest (FL and highest (FH) points of the circle directed vertically downwards are :
(a) FL=mg−T1FH=mg+T2
(b) FL=mg+T1,FH=mg−T2
(c) FL=mg+T1−mυ21R,FH=mg−T2+mυ21R
(d) FL=mg−T1−mυ21R,FH=mg+T2+mυ12R
Choose corret alternative T1,υ1 denote the tension and speed at the lowest point T2,υ2 denote corresponding valuse at the highest point .03:43View SolutionA stone of mass m tied to the end of a string revolves in a vertical circle of radius R. The net forces at the lowest and highest points of the circle directed vertically downwards are: [Choose the correct alternative] Lowest point Highest point T_(1) and V_(1) denote the tension and speed at the lowest point T_(2) and V_(2) denote the corresponding values at the highest points.
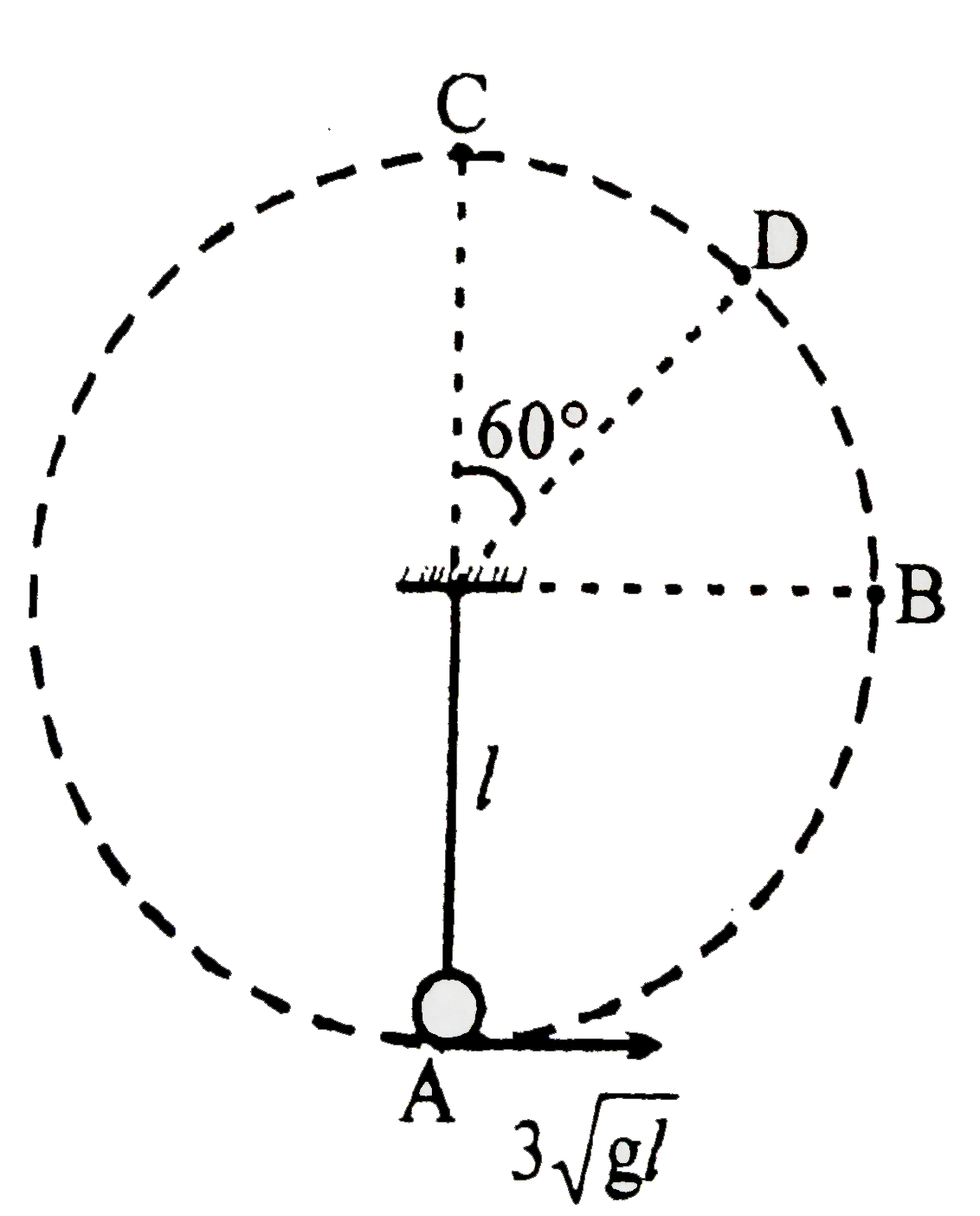
04:09View SolutionA particle of mass m is suspended from a string so that it can move in a circular path in vertical plane. It is given v=3√glat the bottom most point. Match the values of tension in string at A,B,C,D
Column IColumn II(A) TA(P) 10 mg ),((B) T_(B),,(Q)7mg),((C) T_(C),,(R)4mg),((D) T_(D),,(S)5mg),(,,(T)5.5mgView Solutionm संहति के पत्थर को किसी डोरी के एक सिरे से बांधकर R त्रिज्या के ऊध्वार्धर वृत्त में घुमाया जाता है। वृत्त के निम्नतम तथा उच्तम बिन्दुओ पर ऊर्ध्वधरत: अधोमुखी दिशा में नेट बल है: (सही विकल्प चुनिए)
निम्नतम बिंदु पर उच्चतम बिंदु पर
(i)mg−T1mg+T2(ii)mg+T1mg−T2(iii)mg+T1(mv21)/Rmg−T2+(mv22)/R(iv)mg−T1−(mv21)/Rmg+T2+(mv22)/R
यहाँ T1 तथा v1 निम्नतम बिंदु पर तनाव तथा चाल दर्शाते है। T2 तहत v2 इनके उच्चतम बिंदु पर तदनुरूपी मान है।02:17View SolutionA small body of mass m is tried to a string and revolved in a vertical circle of radius r. If the tension in the string at the highest point is mg, what is its speed there?
View Solutionm संहति के पत्थर को किसी डोरी के एक सिरे से बांधकर R त्रिज्या के ऊर्ध्वाधर वृत्त में घुमाया जाता है । वृत्त के निम्नतम तथा उच्चतम बिन्दुओ पर उर्ध्वाधरतः अधोमुखी दिशा में नेट बल है - (सही विकल्प चुनिए)
निम्न बिंदु परउच्चतम बिंदु पर(i)mg−T1mg+T2(ii)mg+T1mg−T2(iii)mg+T1−(mv21)/Rmg−T2+(mv22)/R(iv)mg−T1−(mv21)/Rmg+T2+(mv22)/R
यहाँ T1 तथा v1 निम्नतम बिंदु पर तनाव तथा चाल दर्शाते है T2 तथा v2 इनके उच्चतम बिंदु पर तदनुरूपी मान है ।02:42View SolutionA stone of mass 1kg is tied with a string and it is whirled in a vertical circle of radius 1 m. If tension at the highest point is 14 N, then velocity at lowest point will be
02:41View SolutionA stone of mass m tied to the end of a string revolves in a vertical circle of radius R. The net force at the lowest and highest points of the circle directed vertically downwards are: [ Choose the correct alternative]
Lowest Point Highest point
(a) mg +T1 mg+T2
(b) mg +T1 mg−T2
(c ) mg + T1−(mv21)/R mg−T2+(mv21)/R (d) mg -T1−(mv21)/R mg+T2+(mv21)/R
T1andv1 denote the tension and speed at the lowest point. T2andv2 denote corresponding values at the highest point.02:06View SolutionA stone of mass m tied to the end of a string revolves in a vertical circle of radius R. The net forces at the lowest and highest points of the circle directed vertically downwards are : [Choose the correct alternative
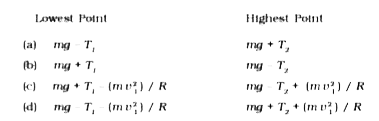
T1 and v1 denote the tension and speed at the lowest point. T2 and v2 denote corresponding values at the highest point.02:34View Solution
