A particle of mass m is suspended from a string so that it can move in a circular path in vertical plane. It is given v=3√glat the bottom most point. Match the values of tension in string at A,B,C,D
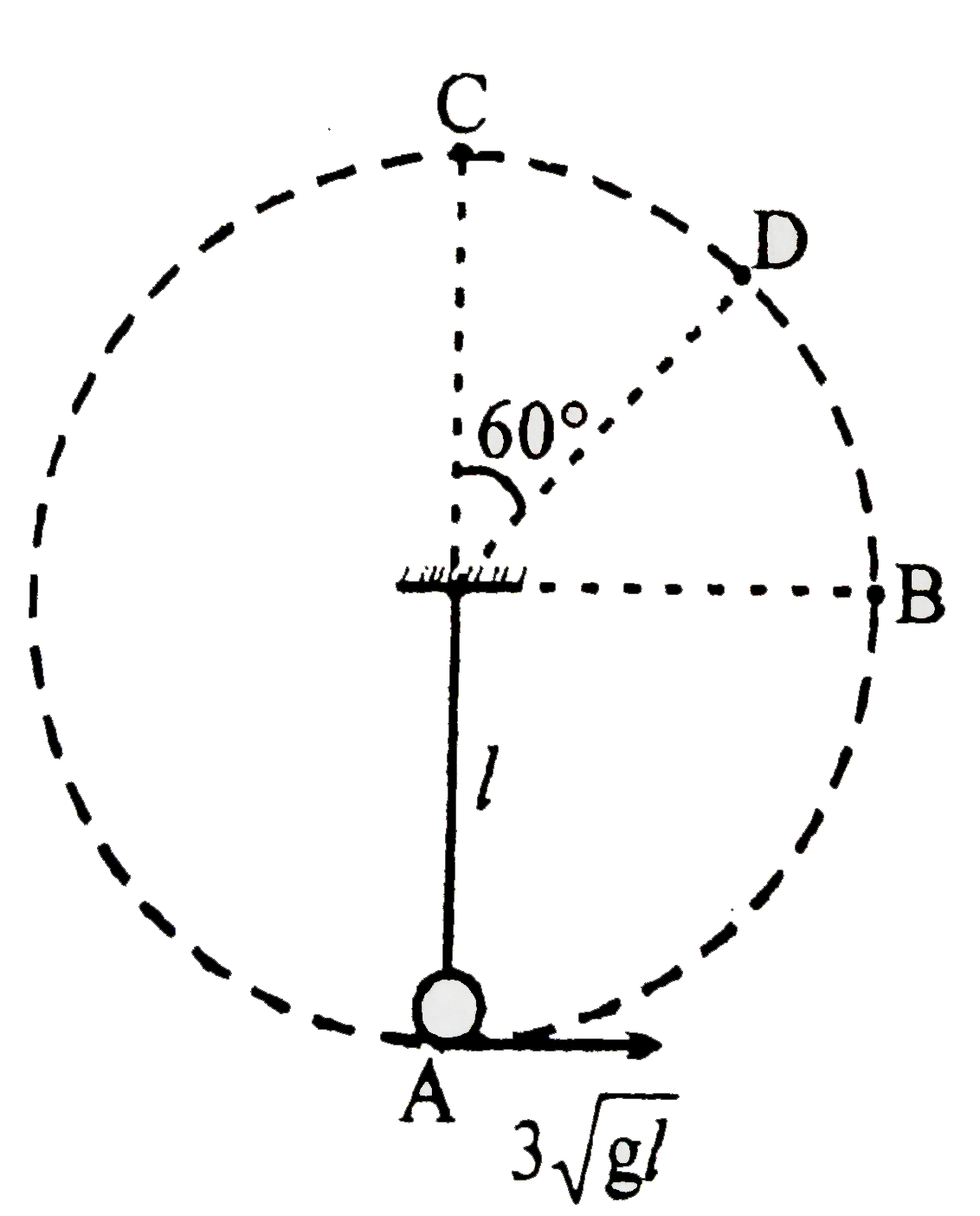
Column IColumn II(A) TA(P) 10 mg ),((B) T_(B),,(Q)7mg),((C) T_(C),,(R)4mg),((D) T_(D),,(S)5mg),(,,(T)5.5mg
The correct Answer is:P
Q
R
T
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom. Match the following:

Column-1 Column-2(A)Velocity at B(P) 7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5mg(T)None
View SolutionA particle of mass m is tied to light string and rotated with a speed v along a circular path of radius r. If T=tension in the string and mg= gravitational force on the particle then actual forces acting on the particle are
View Solution
Knowledge Check
A particle of mass m is tied to light string and rotated with a speed v along a circular path of radius r. If T=tension in the string and mg= gravitational force on the particle then actual forces acting on the particle are
Amg and T onlyBmg, T and an additional force of mv2/r directed inwardsCmg, T and an additational force of mv2/r directed outwardsDonly a force mv2/r directed outwardAssuming the earth as an spherical body, for seconds pendulum
Column - IColumn - II(a) at pole(p) T>2s(b) on a satellite(q) T<2s(c) at mountain(r) T = 2s(d) at centre of earth(s) T = 0(t) T>∞Aa - q, b - t, c - p, d - tBa - q, b - s, c - t, d - tCa - t, b - p, c - s, d - tDa - t, b - s, c - q, d - tA particle is suspended from a string of length R. It is given a velocity u=3√gR at the lowest point.

column−I column−II(A)Velocity at B (P)7mg(B) Velocity at C (Q)√5qR(C)Tension in string at B(R) (R)√7gR(D)Tension in string atC(S) (S)4mgAA-R, B-Q, C-P, D-SBA-Q, B-R, C-S, D-PCA-R, B-P, C-Q, D-SDA-P, B-S, C-R, D-Q
Match the following :
Column-IColumn-II(A)3s1(p)n=3(B)3p1(q)l=0(C)4s1(r)l=1(D)4d1(s)m=0(t)m=±1
View SolutionMatch the following :
Column-IColumn-II(A)3s1(p)n=3(B)3p1(q)l=0(C)4s1(r)l=1(D)4d1(s)m=0(t)m=±1
View SolutionA particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
View SolutionA particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
View SolutionA particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
View Solution
