A thin spherical shell of radius R lying on a rough horizontal surface is hit sharply and horizontally by a cue. Where should it be hit so that the shell does not slip on the surface?
The correct Answer is:A, B, C
Let the cue strickes at as height h above the center for pure rolling
Vc=Rω
Applying law of conservation of angular momentum at point A.
−μCh−θω=0
mvCh=23mR2(V0R)
h=2R3
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 2
15 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
A thin spherical shell lying on a rough horizontal surface is hit by as cue in such a way that the line of action passes through the centre of the shell. As a result the shell starts moving with a linear speed v without any initial angualr velocity. Find the linear speed of the shell after it starts pure rolling on the surface.
View SolutionA uniform wheel of radius R is set lying on a rough horizontal surface is hit by as cue is such a way that the line of action passes thruogh the centre of the shell. As a result the shelll starts movign with a linear speed v without any initial angular velocity. Find the linear speed of the shell after it starts pure rolling on the surface.
View Solution
Knowledge Check
A sphere of radius R is rolling on a rough horizontal surface. The magnitude of velocity of A with respect to ground will be
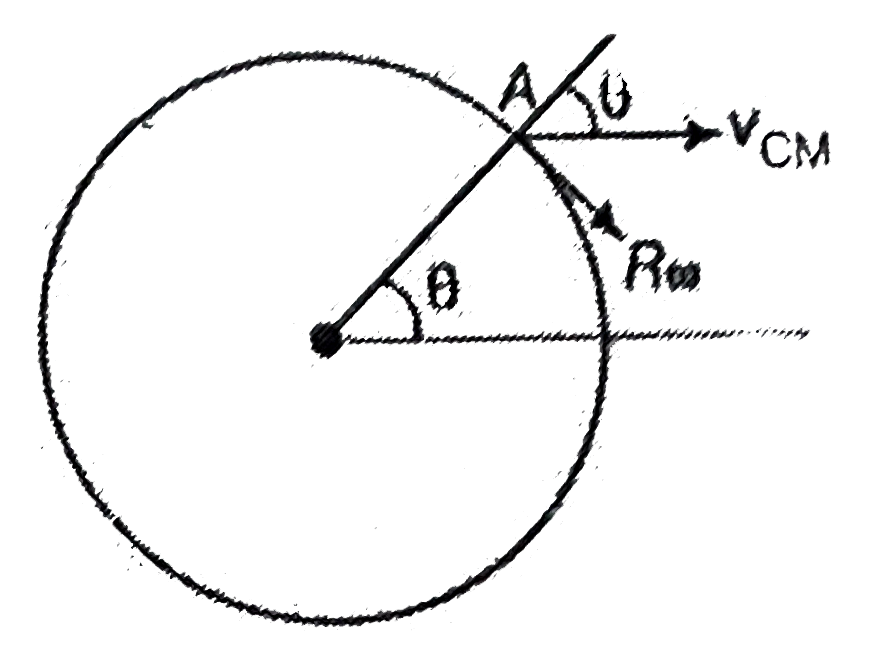 A√2vCMB2VCMsinθC√2vCM√1+sinθDNo sufficient information
A√2vCMB2VCMsinθC√2vCM√1+sinθDNo sufficient informationA solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
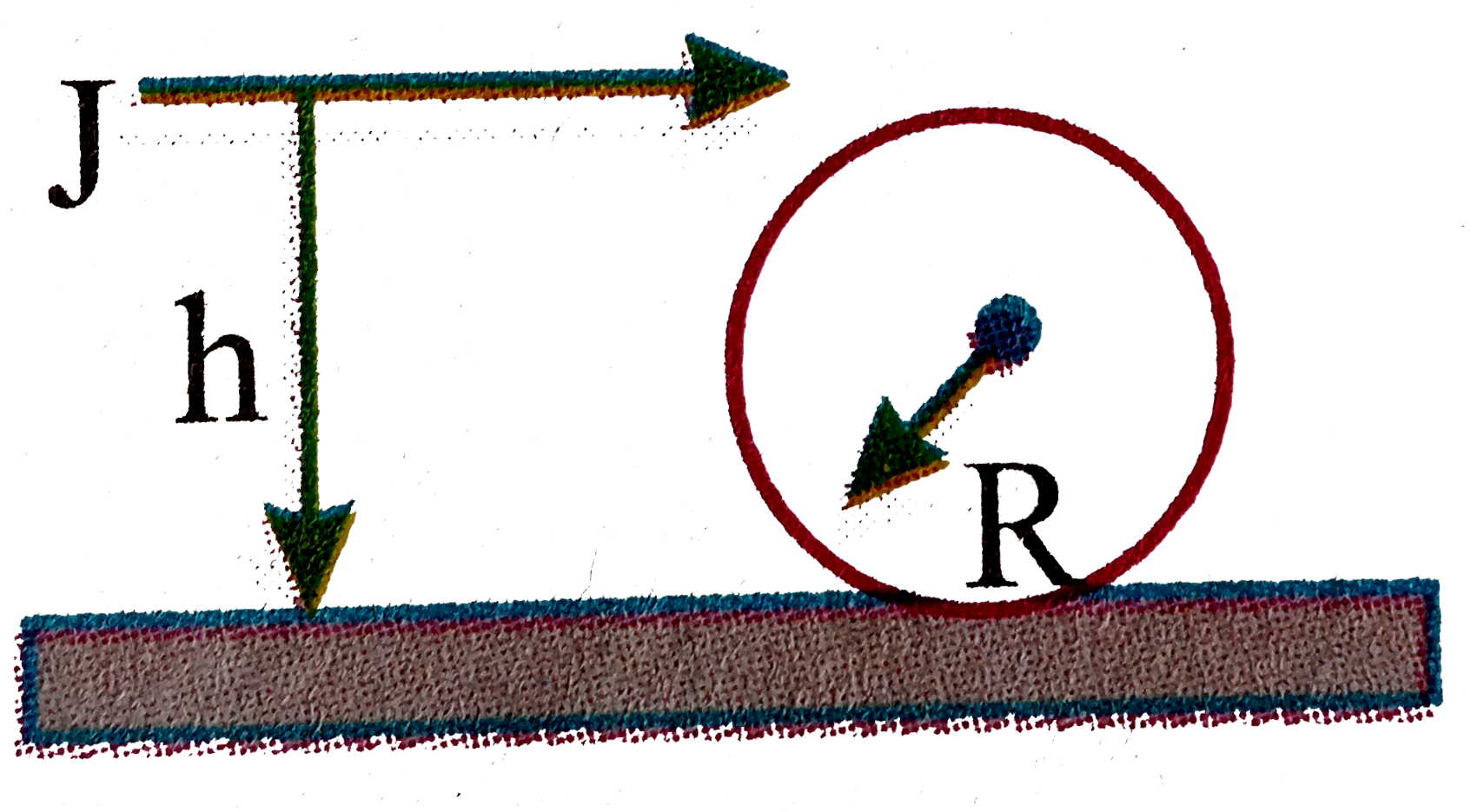 A2R5B5R2C7R5D9R5
A2R5B5R2C7R5D9R5A disc of radius R rolls on a rough horizontal surface. The distance covered by the point A in one revolution is
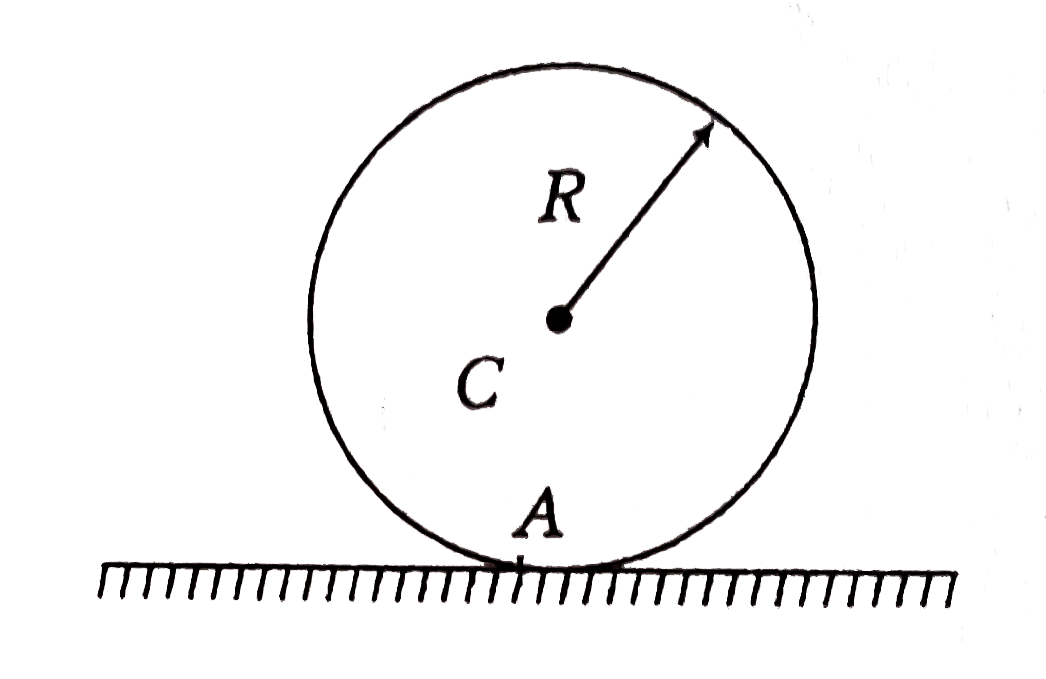 A2πRB2RC8RDπR
A2πRB2RC8RDπR
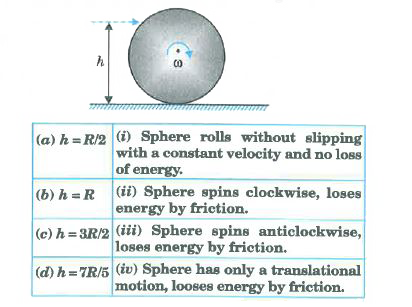
A uniform sphere of mass m and radius R is placed on a rough horizontal surface. The sphere is struck horizontally at a heighth from the floor. Match the following:
View SolutionA solid cylindrical roller of mass M and radius R is rolled on a rough horizontal surface by applying a horizontal force F at the top most point. The frictional force between the roller and the surface, is
View SolutionA unifrom sphere of mass m and radius R is placed on a rough horizontal surface (figure) .the sphere is stuuck horizontally at a height h from the floor . Match the following
View SolutionA sphereicla ball of mass M and radius R is projected along a rough horizontal surface so that initially (t=0) it slides with a linear speed v0 but does not rotate. As it slides, it begins to spin and eventually rolls without slipping. How long does it take to begin rolling without slipping?
View SolutionInside a uniformly accelerating thin-walled spherical shell of radius R which is rolling on horizontal surface there is a small body slipping around Angle of friction between body and inner surface of sphere is 23∘ which of the following can be the acceleration a of the center of sphere to ensure that the small body stays at R/2 distance from the surface?

View Solution
