A transverse waves is travelling in a string. Study following statement.
(i) Equation of the wave is equal to the shape of the string at an instant t. (ii) Equation of thhe wave is general equation for displacement of a particle of the string (iii) Equation of the wave must be sinusoidal equation (iv) Equation of the wave is an equation for displacement of the particle at one end only.correct statement are
(i) and (ii)
(ii) and (iii)
(i) and (iii)
(ii) and (iv)
The correct Answer is:a
To solve the question, we need to analyze each of the four statements regarding the transverse wave traveling in a string and determine which ones are correct.
1. Statement (i): "Equation of the wave is equal to the shape of the string at an instant t."
- The wave equation can be expressed as y=Asin(ωt−kx).
- If we fix time t (e.g., t=2 seconds), we can express the equation as y=Asin(2ω−kx).
- In this form, y is a function of x for a fixed t, which represents the shape of the wave at that instant.
- Conclusion: This statement is true.
2. Statement (ii): "Equation of the wave is general equation for displacement of a particle of the string."
- The wave equation y=Asin(ωt−kx) describes the displacement of particles in the string as a function of both time and position.
- If we consider a specific position x (e.g., x=1), the equation becomes y=Asin(ωt−k), showing that the displacement at that position varies with time.
- Conclusion: This statement is true.
3. Statement (iii): "Equation of the wave must be sinusoidal equation."
- While many waves are sinusoidal, the general form of a wave can also be non-sinusoidal. For example, wave equations can take forms like y=(x−vt)2 or y=(x−vt)3, which are valid waveforms but not sinusoidal.
- Conclusion: This statement is false.
4. Statement (iv): "Equation of the wave is an equation for displacement of the particle at one end only."
- The wave equation describes the displacement of all particles along the string, not just at one end. Each point on the string has its own displacement as a function of time and position.
- Conclusion: This statement is false.
Final Summary:
The correct statements are:
- Statement (i) is true.
- Statement (ii) is true.
- Statement (iii) is false.
- Statement (iv) is false.
Thus, the correct statements are 1 and 2.
- The wave equation can be expressed as y=Asin(ωt−kx).
- If we fix time t (e.g., t=2 seconds), we can express the equation as y=Asin(2ω−kx).
- In this form, y is a function of x for a fixed t, which represents the shape of the wave at that instant.
- Conclusion: This statement is true.
- The wave equation y=Asin(ωt−kx) describes the displacement of particles in the string as a function of both time and position.
- If we consider a specific position x (e.g., x=1), the equation becomes y=Asin(ωt−k), showing that the displacement at that position varies with time.
- Conclusion: This statement is true.
- While many waves are sinusoidal, the general form of a wave can also be non-sinusoidal. For example, wave equations can take forms like y=(x−vt)2 or y=(x−vt)3, which are valid waveforms but not sinusoidal.
- Conclusion: This statement is false.
- The wave equation describes the displacement of all particles along the string, not just at one end. Each point on the string has its own displacement as a function of time and position.
- Conclusion: This statement is false.
The correct statements are:
- Statement (i) is true.
- Statement (ii) is true.
- Statement (iii) is false.
- Statement (iv) is false.
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer
1 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct
25 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Subjective
16 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
Knowledge Check
A transverse wave is travelling along a string from left to right. The figure below represents the shape of the string at a given instant. At this instant, among the following, choose the wrong statement.
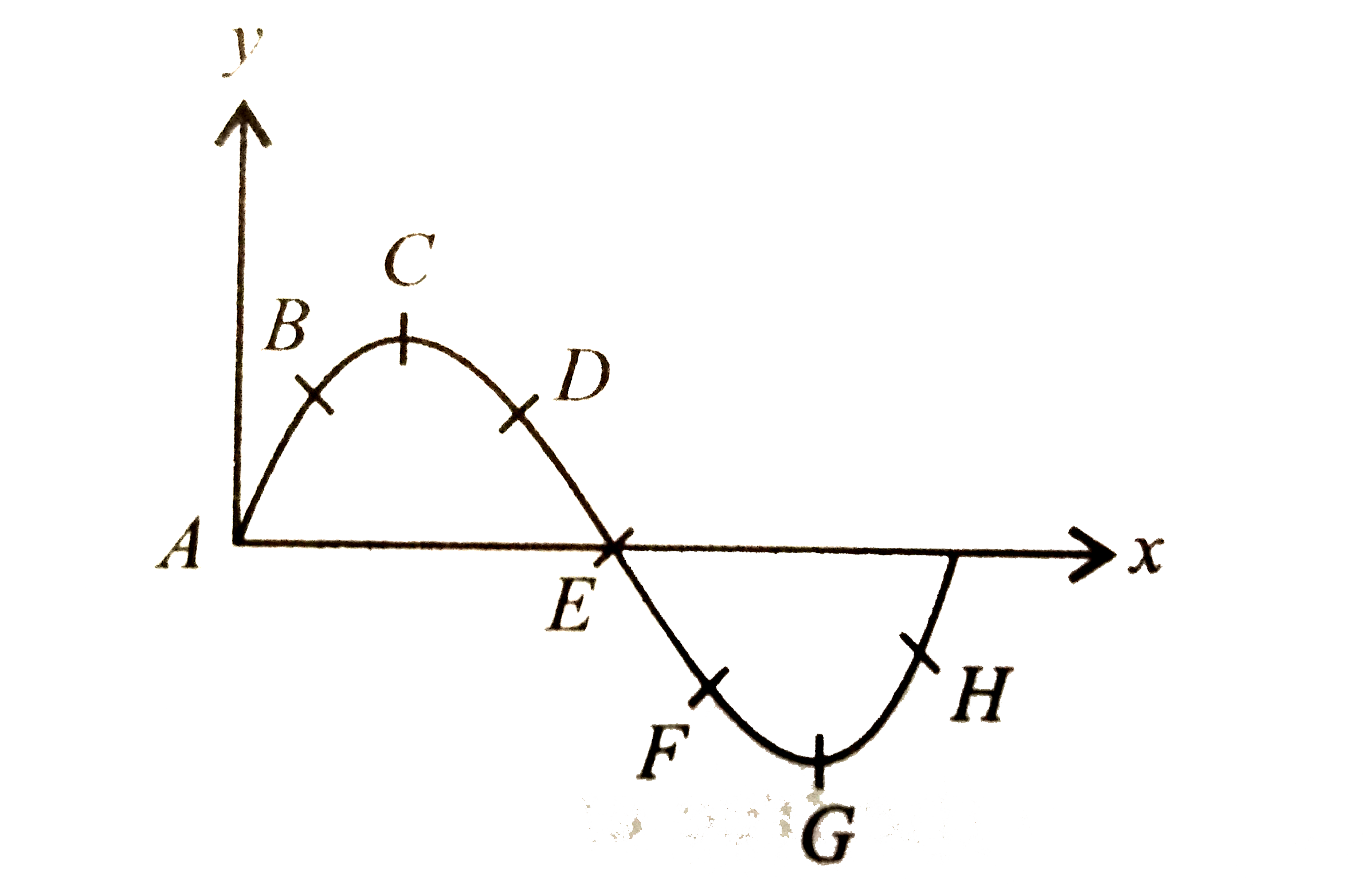 APoints D, E, F have upward positive velocity.BPoints A, B and H have downward negative velocityCPoint C and G have zero velocity.DPoints A and E have minimum velocity.
APoints D, E, F have upward positive velocity.BPoints A, B and H have downward negative velocityCPoint C and G have zero velocity.DPoints A and E have minimum velocity.
Of the following, the equation of progressive wave is
View SolutionIn case of a transverses wave in a string
View SolutionWhich of the following is a wave equation?
View SolutionThe displacement of A particle at x = 0 of a stretched string carrying a wave in the positive X-direction is given by f(t)=Ae−t2. The wave speed is V. Write equation of the wave.
View SolutionA transverse wave travelling on a stretched string is is represented by the equation
y=2(2x−6.2t)2+20. Then ,
View SolutionA transverse mechanical harmonic wave is travellling on a string. Maximum velocity and maximum acceleration of a particle on the string are 3m/s and 90m/s2, respectively. If the wave is travelling with a speed of 20m/s on the string, write wave function describing the wave. \
View SolutionA wave pulse is travelling on a string with a speed v towards the positive X-axis. The shape of the string at t = 0 is given by g(x)=Asin(xa), where A and a are constants. (a) What are the dimensions of A and a ? (b) Write the equation of the wave for a general time 1, if the wave speed is v.
View Solution