A uniform cylinder of length L and mass M having cross-sectional area A is suspended, with its length vertical, from a fixed point by a massless spring such that it is half submerged in a liquid of density σ at equilibrium position. The extension x0 of the spring when it is in equlibrium is:
Mgk(1+LaσM)
Mgk
Mgk(1+LaσM)
Mgk(1−Laσ2M)
The correct Answer is:D
To find the extension x0 of the spring when the cylinder is in equilibrium, we can follow these steps:
Step 1: Identify the forces acting on the cylinder
In equilibrium, the forces acting on the cylinder include:
- The weight of the cylinder, W=Mg (downward).
- The spring force, Fs=kx0 (upward).
- The buoyant force, FB (upward).
Step 2: Write the equilibrium condition
At equilibrium, the sum of the forces acting on the cylinder must be zero. Therefore, we can write the equation:
Mg=kx0+FB
Step 3: Calculate the buoyant force
The buoyant force FB can be calculated using Archimedes' principle. The volume of the submerged part of the cylinder is:
V=A⋅L2
Thus, the buoyant force is given by:
FB=σ⋅V⋅g=σ⋅(A⋅L2)⋅g=σALg2
Step 4: Substitute the buoyant force into the equilibrium equation
Substituting FB into the equilibrium equation gives:
Mg=kx0+σALg2
Step 5: Solve for the extension x0
Rearranging the equation to solve for x0:
kx0=Mg−σALg2
x0=Mg−σALg2k
Step 6: Factor out common terms
Factoring out g gives:
x0=gk(M−σAL2)
Final Expression
Thus, the extension x0 of the spring when the cylinder is in equilibrium is:
x0=gk(M−σAL2)
In equilibrium, the forces acting on the cylinder include:
- The weight of the cylinder, W=Mg (downward).
- The spring force, Fs=kx0 (upward).
- The buoyant force, FB (upward).
At equilibrium, the sum of the forces acting on the cylinder must be zero. Therefore, we can write the equation:
Mg=kx0+FB
The buoyant force FB can be calculated using Archimedes' principle. The volume of the submerged part of the cylinder is:
V=A⋅L2
Thus, the buoyant force is given by:
FB=σ⋅V⋅g=σ⋅(A⋅L2)⋅g=σALg2
Substituting FB into the equilibrium equation gives:
Mg=kx0+σALg2
Rearranging the equation to solve for x0:
kx0=Mg−σALg2
x0=Mg−σALg2k
Factoring out g gives:
x0=gk(M−σAL2)
Thus, the extension x0 of the spring when the cylinder is in equilibrium is:
x0=gk(M−σAL2)
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Exemplar Problems
5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Assertion and reason
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
A uniform cylinder of length (L) and mass (M) having cross sectional area (A) is suspended, with its length vertical, from a fixed point by a massless spring, such that it is half - submerged in a liquid of density (ρ) at equilibrium position. When the cylinder is given a small downward push and released it starts oscillating vertically with small amplitude. If the force constant of the spring is (k), the frequency of oscillation of the cylinder is.
View SolutionA uniform cylinder of length (L) and mass (M) having cross sectional area (A) is suspended, with its length vertical, from a fixed point by a massless spring, such that it is half - submerged in a liquid of density (rho) at equilibrium position. When the cylinder is given a small downward push and released it starts oscillating vertically with small amplitude. If the force constant of the spring is (k), the prequency of oscillation of the cylindcer is.
View SolutionA uniform cylinder of length (L) and mass (M) having cross sectional area (A) is suspended, with its length vertical, from a fixed point by a massless spring, such that it is half - submerged in a liquid of density (rho) at equilibrium position. When the cylinder is given a small downward push and released it starts oscillating vertically with small amplitude. If the force constant of the spring is (k), the prequency of oscillation of the cylindcer is.
View SolutionA block of known mass is suspended from a fixed support through a ligh spring. Can you find the time period of vertical oscillation only by measuring the extension of the spring when the block is in equilibrium?
View SolutionA rod of length l and mass m, pivoted at one end, is held by a spring at its mid - point and a spring at far end. The spring have spring constant k. Find the frequency of small oscillations about the equilibrium position.

View SolutionA block of density 2000kg/m3 and mass 10kg is suspended by a spring stiffness 100N/m. The other end of the spring is attached to a fixed support. The block is completely submerged in a liquid of density 1000kg/m3. If the block is in equilibrium position.
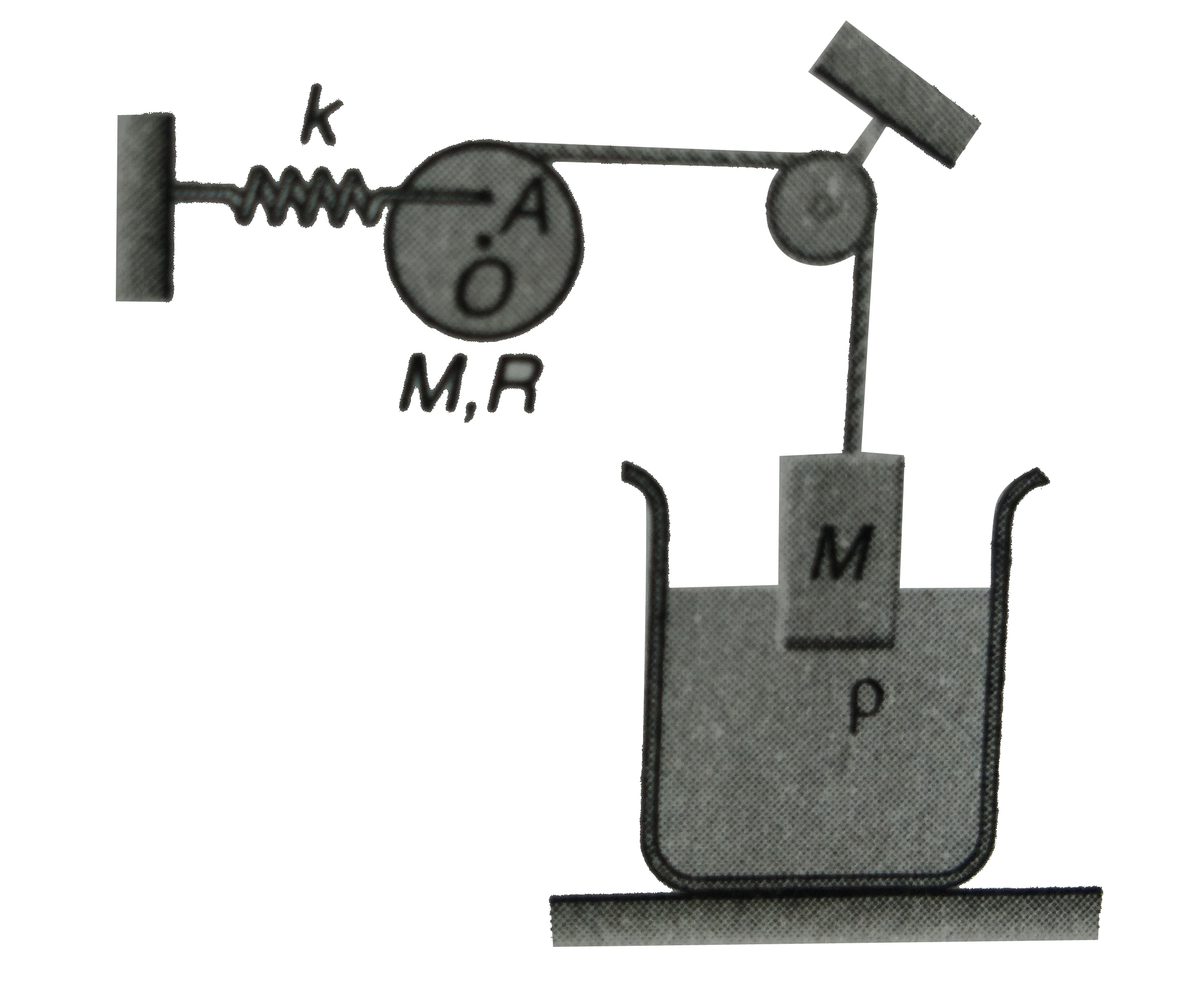
View SolutionFigure shows a solid uniform cylinder of radius R and mass M, which is free to rotate about a fixed horizontal axis O and passes through centre of the cylinder. One end of an ideal spring of force constant k is fixed and the other end is higed to the cylinder at A. Distance OA is equal to R2. An inextensible thread is wrapped round the cylinder and passes over a smooth, small pulley. A block of equal mass M and having cross sectional area A is suspended from free end of the thread. The block is partially immersed in a non-viscous liquid of density ρ.
If in equilibrium, spring is horizontal and line OA is vertical, calculate frequency of small oscillations of the system.
View SolutionA cube of mass m and density D is suspended from a point P by a spring of stiffness k. The system is kept inside a beaker filled with a liquid of density d. The elongation in the spring, assuming D>d, is :

View SolutionA uniform rod of length l, mass m, cross-sectional area A and Young's modulus Y is rotated in horizontal plane about a fixed vertical axis passing through one end, with a constant angular velocity ω. Find the total extension in the rod due to the tension produced in the rod.
View SolutionTwo wires have identical lengths and areas of cross-section are suspended by mass M. Their Young's modulus are in the ratio 2:5.
View Solution
