A uniform cylinder of length (L) and mass (M) having cross sectional area (A) is suspended, with its length vertical, from a fixed point by a massless spring, such that it is half - submerged in a liquid of density (ρ) at equilibrium position. When the cylinder is given a small downward push and released it starts oscillating vertically with small amplitude. If the force constant of the spring is (k), the frequency of oscillation of the cylinder is.
The correct Answer is:i.mg−kx0Aρgii. (1)/(2 pi) sqrt((k + A rho g)/(m))
To find the frequency of oscillation of the uniform cylinder suspended from a spring and partially submerged in a liquid, we can follow these steps:
Step 1: Identify the Forces Acting on the Cylinder
When the cylinder is in equilibrium, the forces acting on it include:
- The buoyant force (upward)
- The spring force (upward)
- The weight of the cylinder (downward)
Step 2: Write the Expression for the Buoyant Force
The buoyant force Fb can be expressed as:
Fb=ρVg
where V is the volume of the liquid displaced by the submerged part of the cylinder. Since the cylinder is half-submerged, the volume displaced can be calculated as:
V=A⋅L2
Thus, the buoyant force becomes:
Fb=ρ(A⋅L2)g
Step 3: Write the Expression for the Spring Force
The spring force Fs when the spring is extended by a distance x is given by Hooke's law:
Fs=kx
Step 4: Set Up the Equation of Motion
At equilibrium, the sum of the forces acting on the cylinder is zero. When displaced by a small distance x and released, the net force Fnet acting on the cylinder is:
Fnet=Fb+Fs−Mg
Substituting the expressions for Fb and Fs:
Fnet=ρ(A⋅L2)g+kx−Mg
Step 5: Apply Newton's Second Law
According to Newton's second law, the net force is also equal to mass times acceleration:
Fnet=Ma
where a is the acceleration of the cylinder. Since acceleration can be expressed as a=d2xdt2, we can write:
Md2xdt2=ρ(A⋅L2)g+kx−Mg
Step 6: Rearrange the Equation
Rearranging gives us:
Md2xdt2+kx+ρ(A⋅L2)g−Mg=0
This can be simplified to:
d2xdt2+kMx=−ρAgL2M+gM
Step 7: Identify the Angular Frequency
The equation of motion is in the form of simple harmonic motion (SHM):
d2xdt2+ω2x=0
where:
ω2=kM+ρAg2M
Step 8: Calculate the Frequency
The frequency f of the oscillation is given by:
f=ω2π=12π√kM+ρAg2M
Final Answer
Thus, the frequency of oscillation of the cylinder is:
f=12π√kM+ρAg2M
When the cylinder is in equilibrium, the forces acting on it include:
- The buoyant force (upward)
- The spring force (upward)
- The weight of the cylinder (downward)
The buoyant force Fb can be expressed as:
Fb=ρVg
where V is the volume of the liquid displaced by the submerged part of the cylinder. Since the cylinder is half-submerged, the volume displaced can be calculated as:
V=A⋅L2
Thus, the buoyant force becomes:
Fb=ρ(A⋅L2)g
The spring force Fs when the spring is extended by a distance x is given by Hooke's law:
Fs=kx
At equilibrium, the sum of the forces acting on the cylinder is zero. When displaced by a small distance x and released, the net force Fnet acting on the cylinder is:
Fnet=Fb+Fs−Mg
Substituting the expressions for Fb and Fs:
Fnet=ρ(A⋅L2)g+kx−Mg
According to Newton's second law, the net force is also equal to mass times acceleration:
Fnet=Ma
where a is the acceleration of the cylinder. Since acceleration can be expressed as a=d2xdt2, we can write:
Md2xdt2=ρ(A⋅L2)g+kx−Mg
Rearranging gives us:
Md2xdt2+kx+ρ(A⋅L2)g−Mg=0
This can be simplified to:
d2xdt2+kMx=−ρAgL2M+gM
The equation of motion is in the form of simple harmonic motion (SHM):
d2xdt2+ω2x=0
where:
ω2=kM+ρAg2M
The frequency f of the oscillation is given by:
f=ω2π=12π√kM+ρAg2M
Thus, the frequency of oscillation of the cylinder is:
f=12π√kM+ρAg2M
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct
107 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct
35 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.2
23 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
A uniform cylinder of length L and mass M having cross-sectional area A is suspended, with its length vertical, from a fixed point by a massless spring such that it is half submerged in a liquid of density σ at equilibrium position. The extension x0 of the spring when it is in equlibrium is:
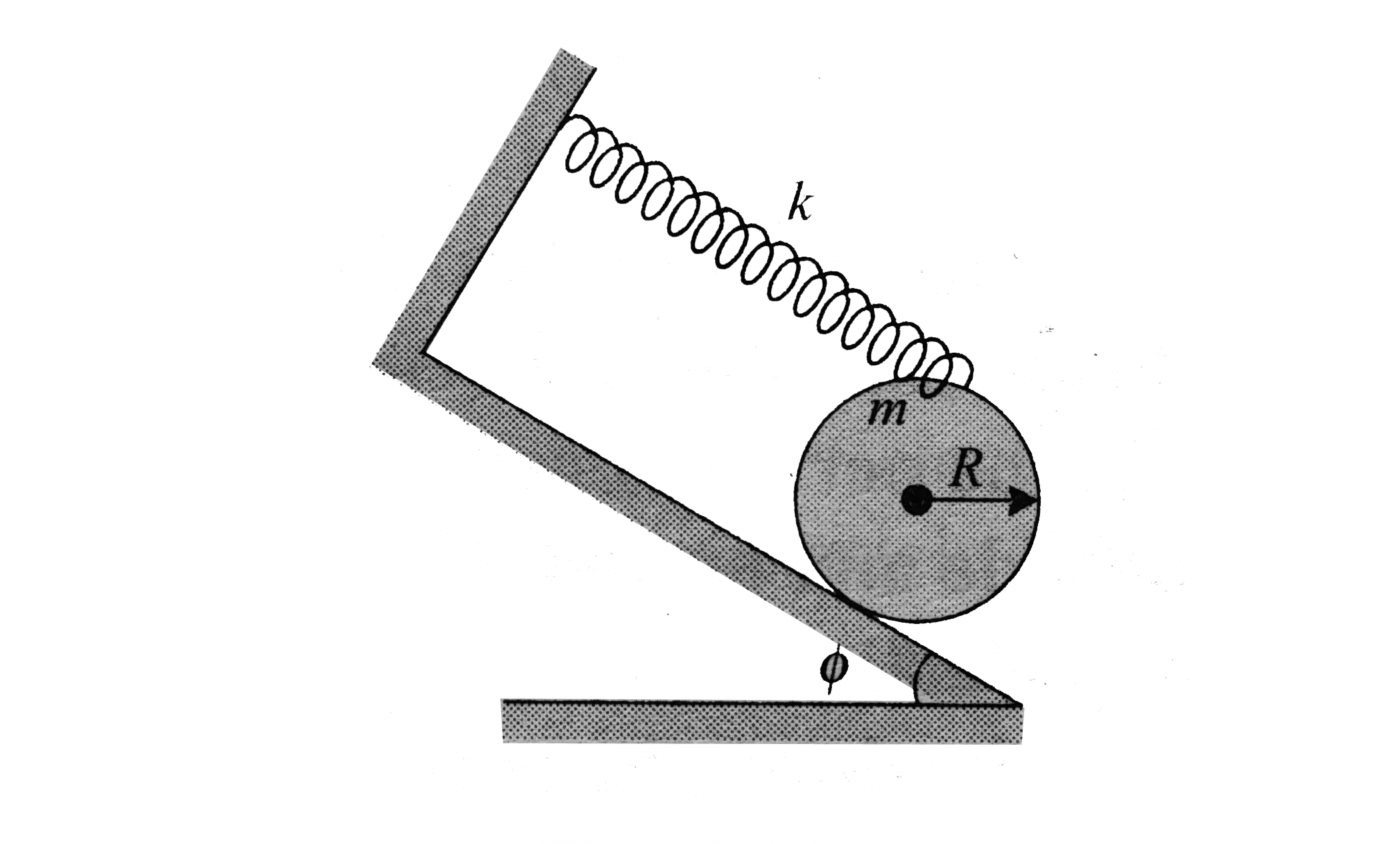
View SolutionA uniform cylinder of mass m and radius R is in equilibrium on an inclined by the action of a light spring of stiffnesk, gravity and reaction force acting on it .If the angle of inclination of the plane is ϕ , find the angular frequency of small oscillation of the cylinder
View Solution
Knowledge Check
A uniform cylinder of length L and mass M having cross-sectional area A is suspended, with its length vertical, from a fixed point by a massless spring such that it is half submerged in a liquid of density σ at equilibrium position. The extension x0 of the spring when it is in equlibrium is:
AMgk(1+LaσM)BMgkCMgk(1+LaσM)DMgk(1−Laσ2M)A particle of mass m is hanging vertically by an ideal spring of force constant K . If the mass is made to oscillate vertically, its total energy is
Amaximum at the extreme positionBmaximum at the mean positionCminimum at the mean positionDsame at all positions.
A rod of length l and mass m, pivoted at one end, is held by a spring at its mid - point and a spring at far end. The spring have spring constant k. Find the frequency of small oscillations about the equilibrium position.

View SolutionA rod of length L, cross sectional area A and density ρ is hanging from a rigid support by spring of stiffries k. A very small sphere of mass m is rigidly attached at the bottom of the rod. The rod is partially immersed in a liquid of density ρ. Find the period of small oscillations.
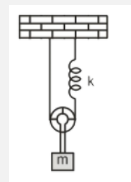
View SolutionA system shown in the figure consists of a massless pulley, a spring of force constant k displaced vertically downwards from its equilibrium position and released, then the Period of vertical oscillations is
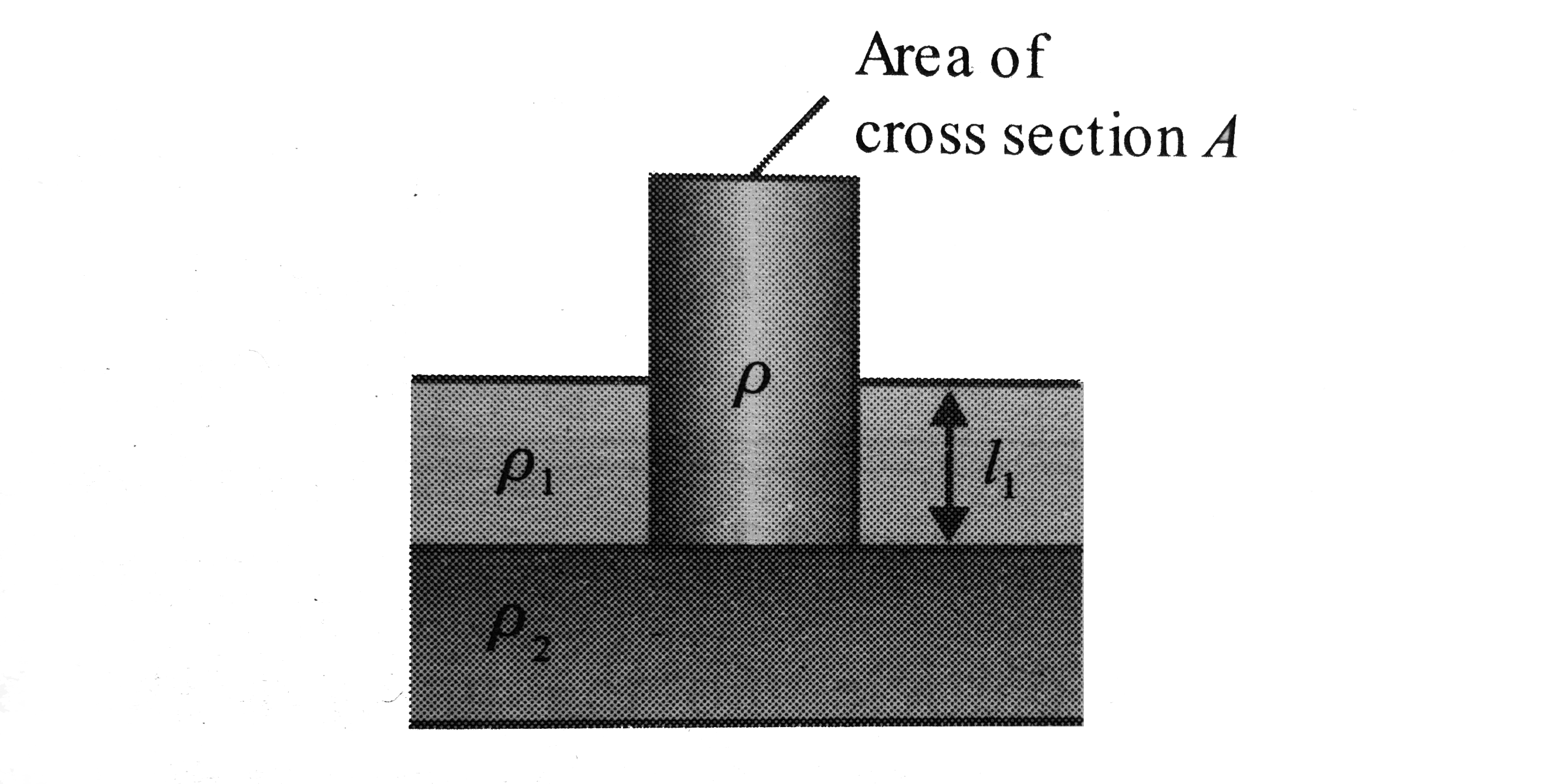
View SolutionA vertical pole of length l , density ρ , area of cross section A floats in two immiscible liquids of densities ρ1and ρ2. In equuilibrium possition the bottom end is at the interface of the liquids. When the cylinder is displaced vertically, find the time period of oscillation.
View SolutionA solid cylinder of mass M=10kg and cross - sectional area A=20cm2 is suspended by a spring of force contant k=100N/m and hangs partically immersed in water. Calculate the period of small oscillation of the cylinder.
View Solution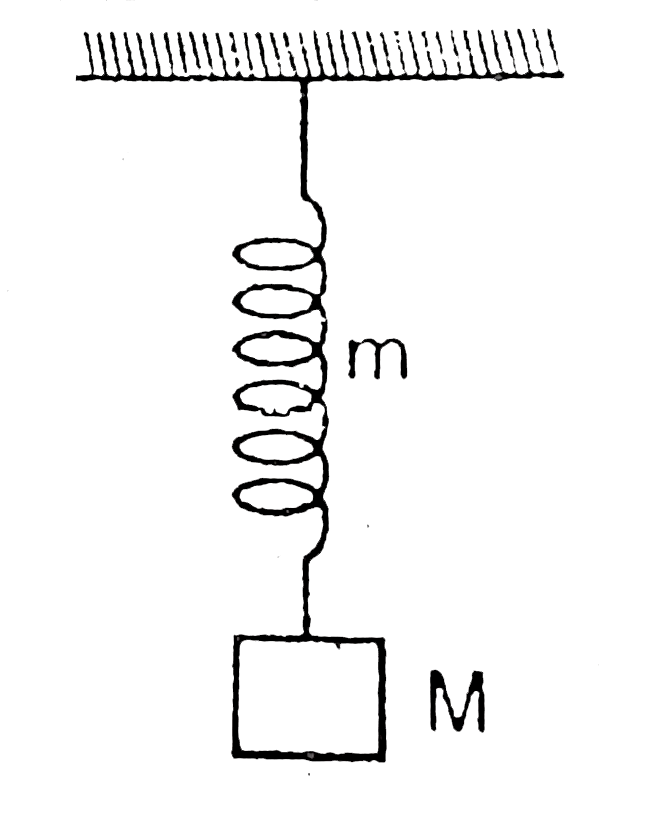
A block of M is suspended from a vertical spring of mass m as shown in the figure. When block is pulled down and released the mass oscillates with maximum velocity v.
View Solution
