A uniform disc of radius 5.0 cm and mass 200g is fixed at its centre to a metal wire, the other end of which is fixed with a clamp. The hanging disc is rotated about the wire through an angle and is released. If the disc makes torsional oscillations with time period 0.20s, find the torsional constant of the wire.
To find the torsional constant of the wire, we will follow these steps:
Step 1: Identify the given values
- Radius of the disc, r=5.0cm=0.05m
- Mass of the disc, m=200g=0.2kg
- Time period of oscillation, T=0.20s
Step 2: Calculate the moment of inertia I of the disc
The moment of inertia I of a uniform disc about an axis through its center is given by the formula:
I=12mr2
Substituting the values:
I=12×0.2kg×(0.05m)2
Calculating:
I=12×0.2×0.0025=0.00025kg m2=2.5×10−4kg m2
Step 3: Use the formula for the time period of torsional oscillations
The time period T of a torsional pendulum is given by the formula:
T=2π√Ik
Where k is the torsional constant. Rearranging this formula to solve for k:
T2=4π2Ik⟹k=4π2IT2
Step 4: Substitute the values to find k
Substituting the values of I and T:
k=4π22.5×10−4kg m2(0.20s)2
Calculating T2:
T2=(0.20)2=0.04s2
Now substituting this back into the equation for k:
k=4π22.5×10−40.04
Calculating k:
k=4×(3.14)2×2.5×10−40.04
k≈4×9.86×6.25×10−3≈0.25kg m2/s2
Final Answer
The torsional constant k of the wire is approximately:
k≈0.25N m/rad
- Radius of the disc, r=5.0cm=0.05m
- Mass of the disc, m=200g=0.2kg
- Time period of oscillation, T=0.20s
The moment of inertia I of a uniform disc about an axis through its center is given by the formula:
I=12mr2
Substituting the values:
I=12×0.2kg×(0.05m)2
Calculating:
I=12×0.2×0.0025=0.00025kg m2=2.5×10−4kg m2
The time period T of a torsional pendulum is given by the formula:
T=2π√Ik
Where k is the torsional constant. Rearranging this formula to solve for k:
T2=4π2Ik⟹k=4π2IT2
Substituting the values of I and T:
k=4π22.5×10−4kg m2(0.20s)2
Calculating T2:
T2=(0.20)2=0.04s2
Now substituting this back into the equation for k:
k=4π22.5×10−40.04
Calculating k:
k=4×(3.14)2×2.5×10−40.04
k≈4×9.86×6.25×10−3≈0.25kg m2/s2
The torsional constant k of the wire is approximately:
k≈0.25N m/rad
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - worked out Examples
22 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Objective -1
21 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-2
7 Videos
Similar Questions
A uniform disc of mass m and radius r is suspended through a wire attached to its Centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
View SolutionA circular disc of mass 10kg is suspended by a wire attahced to its centre. The wire is twisted by rotating the disc and released. The period of torsional oscillations is found to be 1.5s. The radius of the disc is 15cm. Determing the torsional spring constant of the wire. (Torsional spring constant α is definied by the relation J=−αθ, where J is the restoring coubple and θ the angle of twist.
View Solution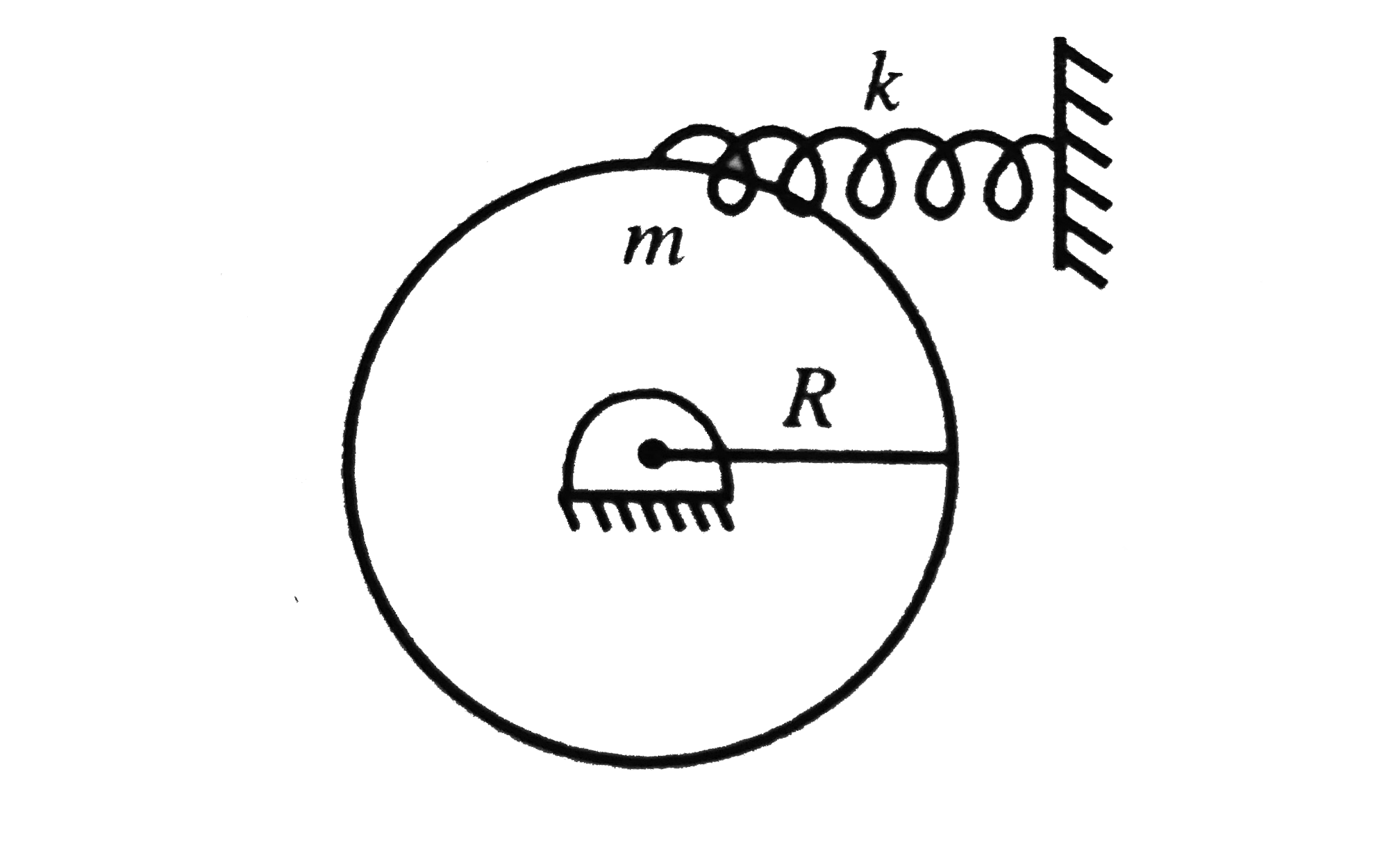
A uniform disc of mass m and radius R is pivoted smoothly at its centre of mass. A light spring of stiffness k is attached with the dics tangentially as shown in the Fig. Find the angular frequency in rads of torsional oscillation of the disc. (Take m=5kg and K=10Nm.)
View Solution
A uniform disc of mass m and radius R is pivoted smoothly at its centre of mass. A light spring of stiffness k is attached with the dics tangentially as shown in the Fig. Find the angular frequency in rads of torsional oscillation of the disc. (Take m=5kg and K=10Nm.)
View SolutionA metal rod of length 'L' and mass 'm' is pivoted at one end. A thin disk of mass 'M' and radius 'R' (< L) is attached at its centre to the free end of the rod. Consider two ways the disc is attached: (case A) - The disc is not free to rotate about its centre and (case B) - the disc is free to rotate about its centre. The rod-disc system after being released from the same displaced position. Which of the following statement(s) is(are) true?
View SolutionA metal rod of length 'L' and mass 'm' is piovted at one end. A thin disk of mass 'M' and radius 'R'(<L). Is attached at its centre to the free end of the rod. Consider two ways the disc is attached: (case A). The disc is not free to rotate about its centre and (case B) the disc is free to rotate about its center. The rod-disc sustem perform SHM in vertical plane after being released from the same displaced potion. Which of the following statement(s) is (are) true?
View SolutionOne end of spring of spring constant k is attached to the centre of a disc of mass m and radius R and the other end of the spring connected to a rigid wall. A string is wrapped on the disc and the end A of the string (as shown in the figure) is pulled through a distance a and then released.
The disc is placed on a horizontal rough surface and there is no slipping at any contact point. What is the amplitude of the oscillation of the centre of the disc?

View SolutionA uniform disc of radius R and mass M is free to rotate about a fixed horizontal axis perpendicular to its plane and passing through its centre. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The block is released from rest. If string does not slip on the rim then find the acceleration of the block. Neglect the mass of the string.
View SolutionA disc of mass 1 kg and radius 10 cm is suspended by vertical wire at its centre. If the time period is 3.2 s. Find the modulus of torison.
View SolutionA uniform disc of mass m and radius R rotates about a fixed vertical axis passing through its centre with angular velocity ω. A particle of same mass m and having velocity of 2ωR towards centre of the disc collides with the disc moving horizontally and sticks to its rim. Then
View Solution
