A uniform metal chain is placed on a rough table such that the one end of chain hangs down over the edge of the table, when one-third of its length hang over the edge, the chain starts sliding. Then the coefficient of static friction is
34
14
23
12
The correct Answer is:D
To solve the problem step by step, we can follow this approach:
Step 1: Understand the setup
We have a uniform metal chain placed on a rough table, with one-third of its length hanging over the edge. When this hanging part reaches a certain point, the chain starts to slide.
Step 2: Define the variables
Let:
- L = total length of the chain
- M = total mass of the chain
- g = acceleration due to gravity
- μ = coefficient of static friction
Step 3: Determine the weight of the hanging part
When one-third of the chain is hanging, the length of the hanging part is:
Length of hanging part=L3
The mass of the hanging part is:
Mass of hanging part=ML⋅L3=M3
The weight of the hanging part is:
Whanging=M3⋅g=Mg3
Step 4: Determine the weight of the part on the table
The length of the part on the table is:
Length on table=L−L3=2L3
The mass of the part on the table is:
Mass on table=ML⋅2L3=2M3
The weight of this part is:
Wtable=2M3⋅g=2Mg3
Step 5: Calculate the normal force
The normal force N acting on the part of the chain on the table is equal to its weight:
N=Wtable=2Mg3
Step 6: Write the equation for limiting friction
The limiting friction Ffriction is given by:
Ffriction=μN=μ⋅2Mg3
Step 7: Set up the equilibrium condition
At the point of sliding, the weight of the hanging part equals the limiting friction:
Whanging=Ffriction
Substituting the values:
Mg3=μ⋅2Mg3
Step 8: Solve for the coefficient of static friction
Cancelling Mg/3 from both sides (assuming M and g are not zero):
1=2μ
Thus,
μ=12
Conclusion
The coefficient of static friction between the metal chain and the table is:
μ=12
We have a uniform metal chain placed on a rough table, with one-third of its length hanging over the edge. When this hanging part reaches a certain point, the chain starts to slide.
Let:
- L = total length of the chain
- M = total mass of the chain
- g = acceleration due to gravity
- μ = coefficient of static friction
When one-third of the chain is hanging, the length of the hanging part is:
Length of hanging part=L3
Mass of hanging part=ML⋅L3=M3
Whanging=M3⋅g=Mg3
The length of the part on the table is:
Length on table=L−L3=2L3
Mass on table=ML⋅2L3=2M3
Wtable=2M3⋅g=2Mg3
The normal force N acting on the part of the chain on the table is equal to its weight:
N=Wtable=2Mg3
The limiting friction Ffriction is given by:
Ffriction=μN=μ⋅2Mg3
At the point of sliding, the weight of the hanging part equals the limiting friction:
Whanging=Ffriction
Substituting the values:
Mg3=μ⋅2Mg3
Cancelling Mg/3 from both sides (assuming M and g are not zero):
1=2μ
Thus,
μ=12
The coefficient of static friction between the metal chain and the table is:
μ=12
Topper's Solved these Questions
Similar Questions
A uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?View SolutionA uniform chain of length l is placed on a rough table, with length l/3 hanging over the edge. If the chain just begins to slide off the table by itself from this portion, the coefficient of friction between the chain and the table is 1/K. Then find the value of K.
View Solution
Knowledge Check
- Question 1 - Select One
A unifrom heavy chain is placed on a table with a part of it hanging over the edge. It just begins to slide when this part is one-third of its length. The coefficient of friction between the table and the chain is
A12B13C23D34 - Question 2 - Select One or More
A uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
AThe acceleration of the chain is (gx/L), where x is the length of the hanging part of chain.BThe acceleration of the chain is (g/L)(L−x), where x is the length of hanging part of chain.CThe velocity of the chain is x√gL, where x is the length of hanging part of chain.DThe velocity of the chain is (L−x)√gL, where x is the length of hanging part of chain. - Question 3 - Select One
A chain is lying on a rough table with a fraction 1/n of its length hanging down from the edge of the table. if it is just on the point of sliding down from the table, then the coefficient of friction between the table and the chain is -
A1nB1(n−1)C1(n+1)Dn−1(n+1)
A chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
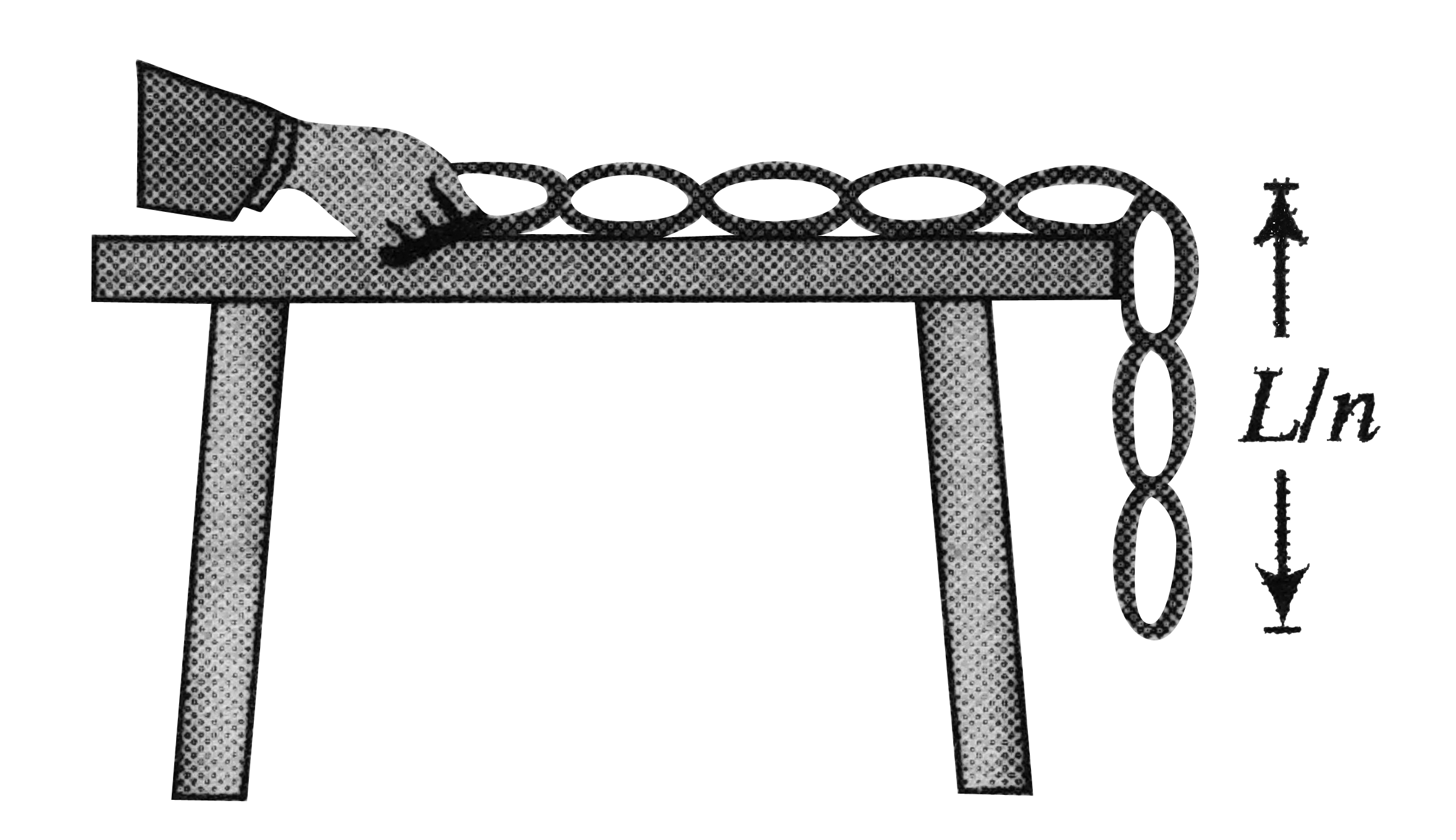 View Solution
View SolutionA uniform chain of length l is placed on rough table with length l/n (where n>1) , hanging over the edge. If the chain just begins to slide off the table by itself from this position, the coefficient of friction between the chain and the table is
View SolutionA uniform rope of length l and mass m lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small part of the rope having over the edge. The rope starts sliding from rest under the weight of the over hanging end. The velocity of the rope when the length of the hanging part is . x
View SolutionA rope lies on atable such that a part of it hangs down the table, when the length of hanging part is 1/3 of entire length the rope just begins to slide. The coefficient of friction between the rope and the table is:-
View SolutionA chain of mass M is placed on a smooth table with 1/n of its length L hanging over the edge. The work done in pulling the hanging portion of the chain back to the surface of the table is:
View Solution

