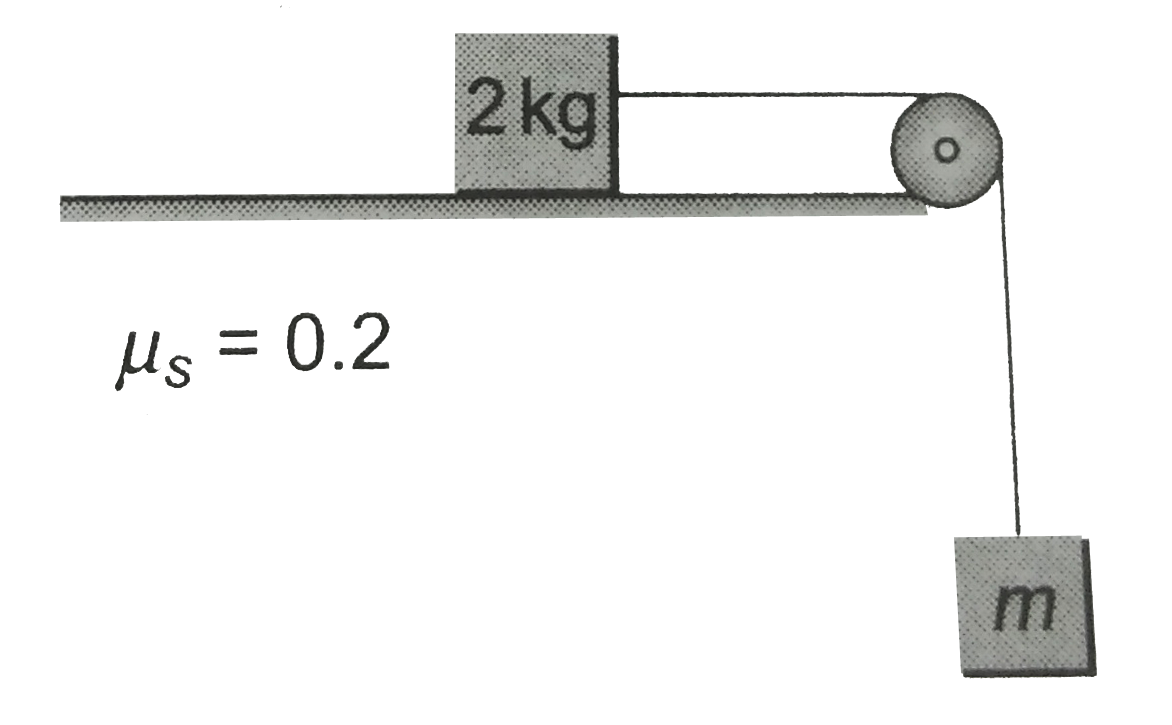
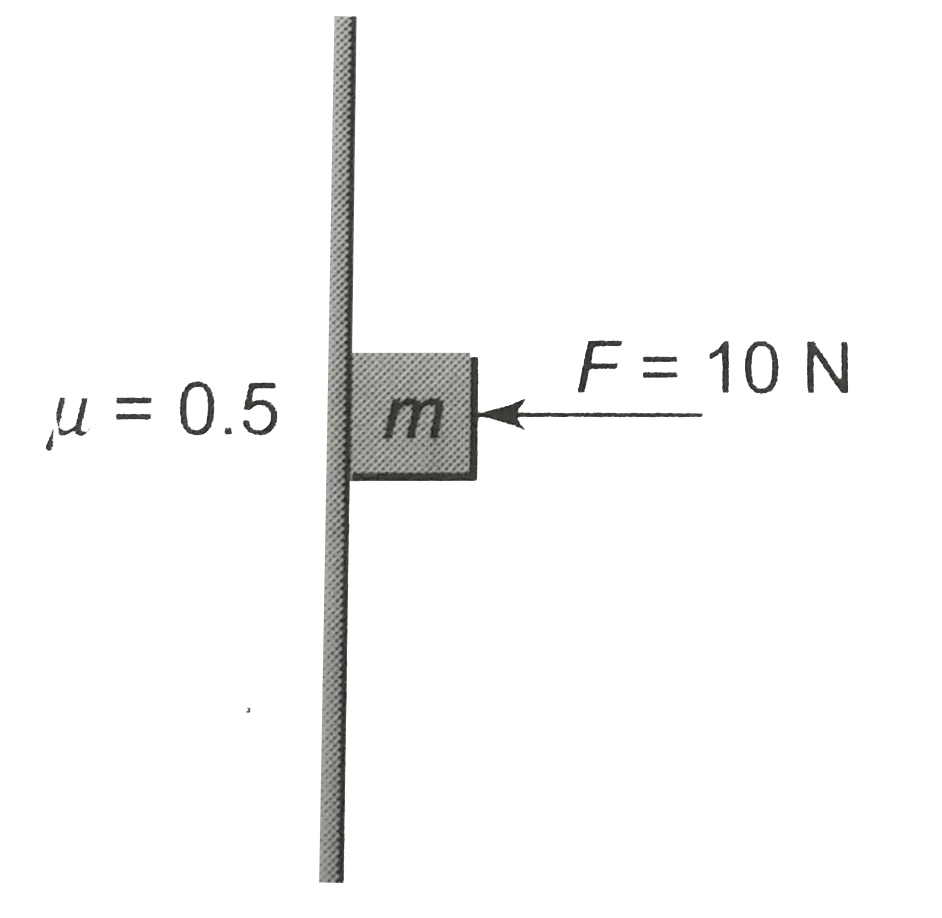
Consider the situation as shown in the figure.
The maximum value of m so that the blocks do not move is
0.2kg
0.4kg
0.6kg
0.8kg
The correct Answer is:B
mg≤μs(2g)⇒m≤(2μs=2×0.2)
m≤0.4kg
Topper's Solved these Questions
Similar Questions
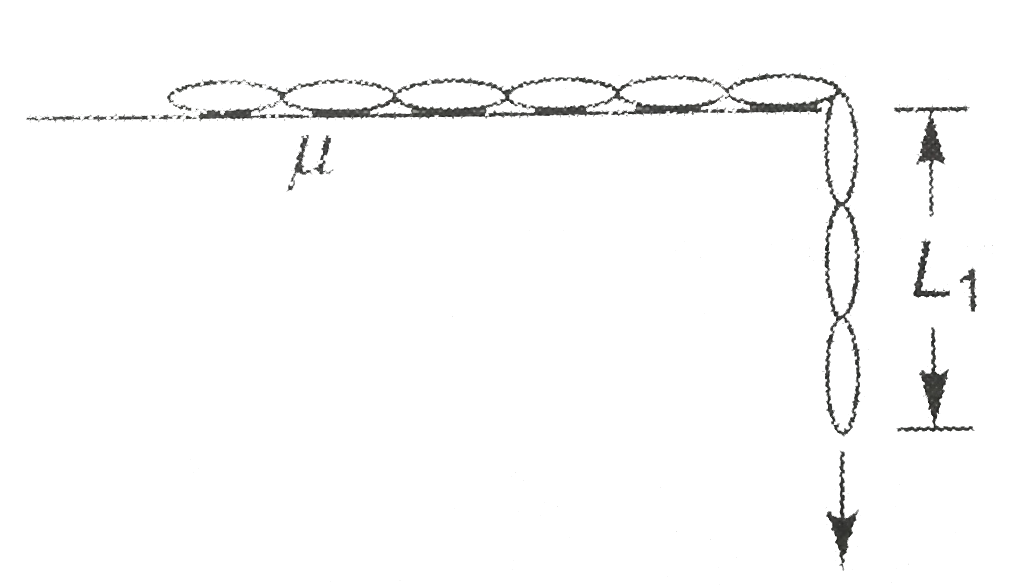
A homogeneous chain of length L lie on a table. If the coefficent of friction is μ , find the maximum length L1 of the chain which can hang from the edge of the table without the chain sliding down.
(b) Consider the situation as shown in the figure. Find teh minimum value of m so that the blocks do not move.
 View Solution
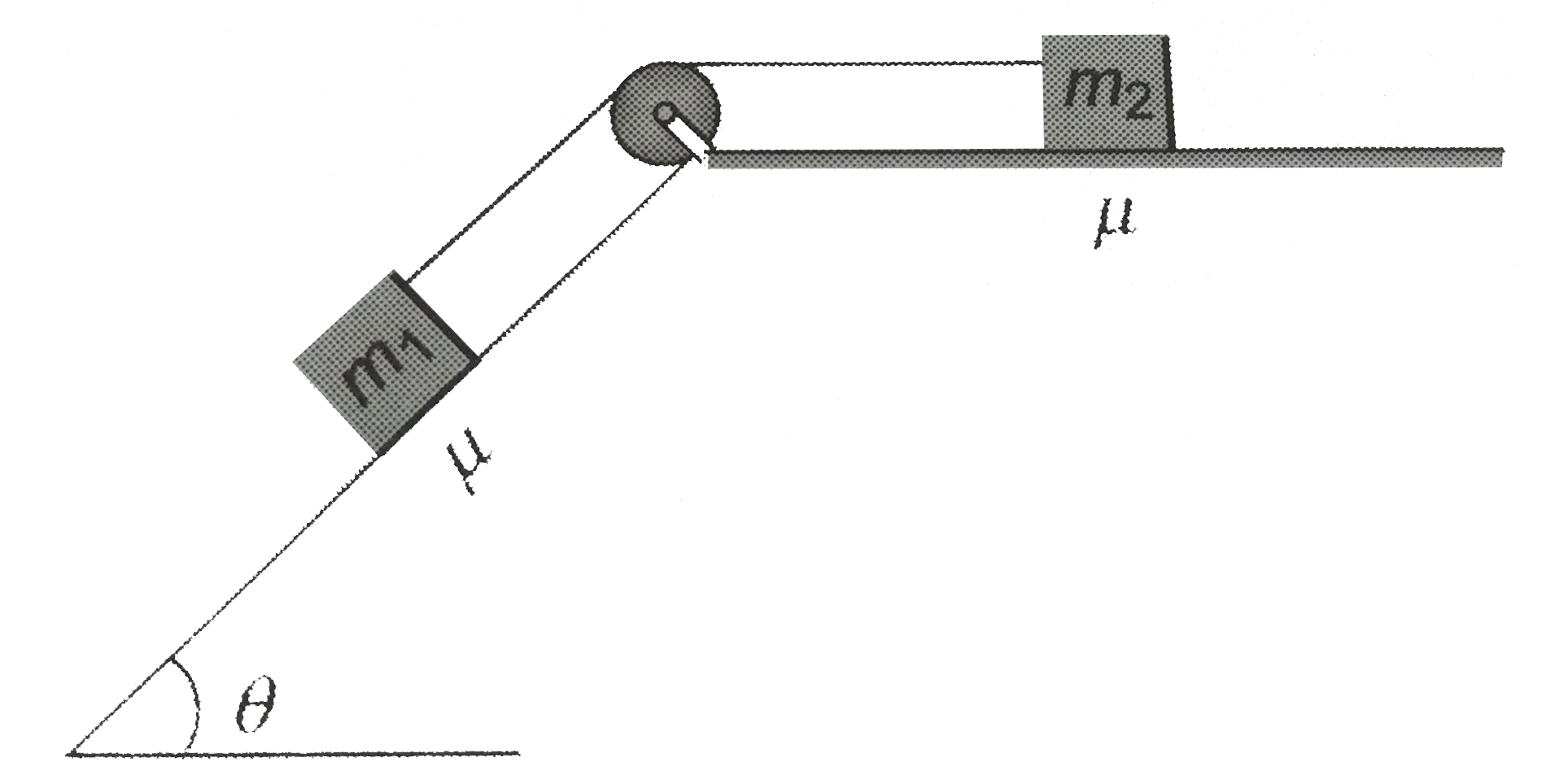
View SolutionConsider the situation as shown in the figure. Find the maximum value of m1/m2 so that the system remains at rest. What will happen if tanθ<μ?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Consider the situation as shown in the firgure.
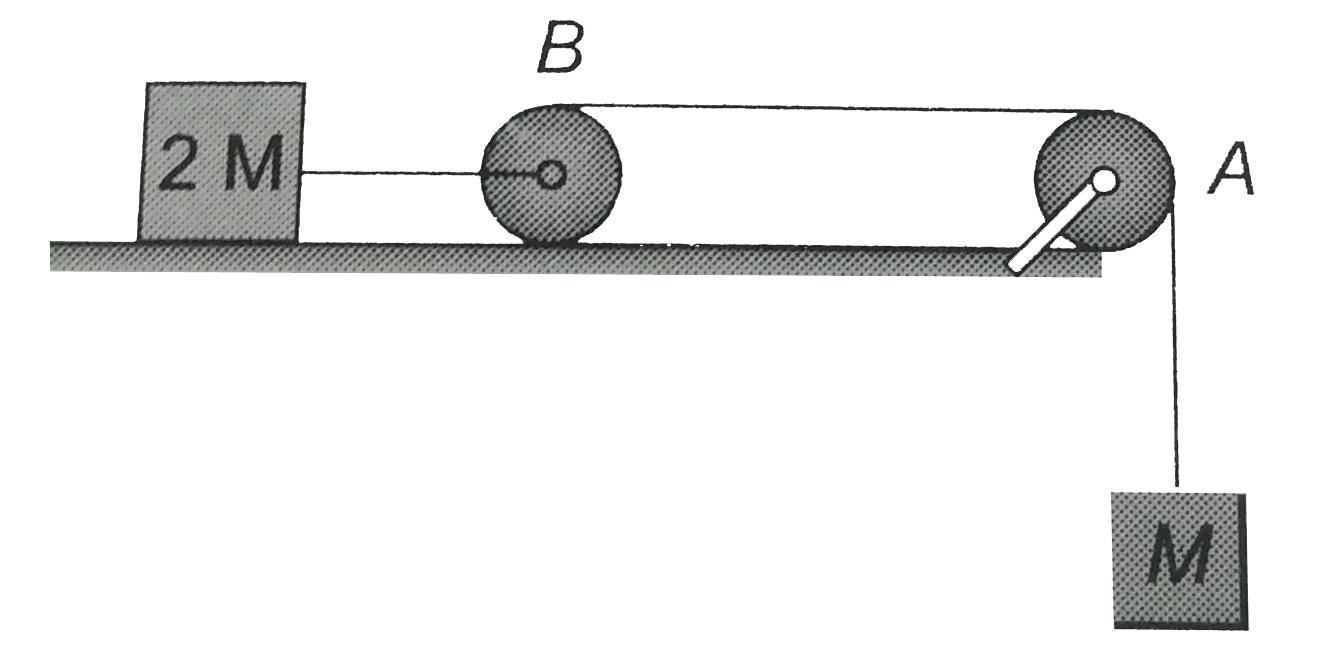 AThe accelaration of M is 2g/3.BThe tension in the string connecting A and B is Mg/3CThe reaction on pulley A is √2 Mg/3 at an angle 45∘ with the horizontal.DAll the options are correct.
AThe accelaration of M is 2g/3.BThe tension in the string connecting A and B is Mg/3CThe reaction on pulley A is √2 Mg/3 at an angle 45∘ with the horizontal.DAll the options are correct. - Question 2 - Select One
Consider the situation shown in the figure.
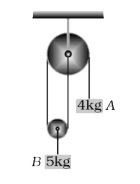
Find acceleration of A.A2g7Bg7C3g7D2g5 - Question 3 - Select One
Consider the situation shown in the figure.
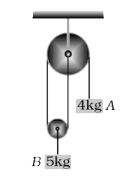
Find acceleration of B.Ag7B2g7C3g7D2g5
Consider the situation as shown in the figure. Locate the center of mass.
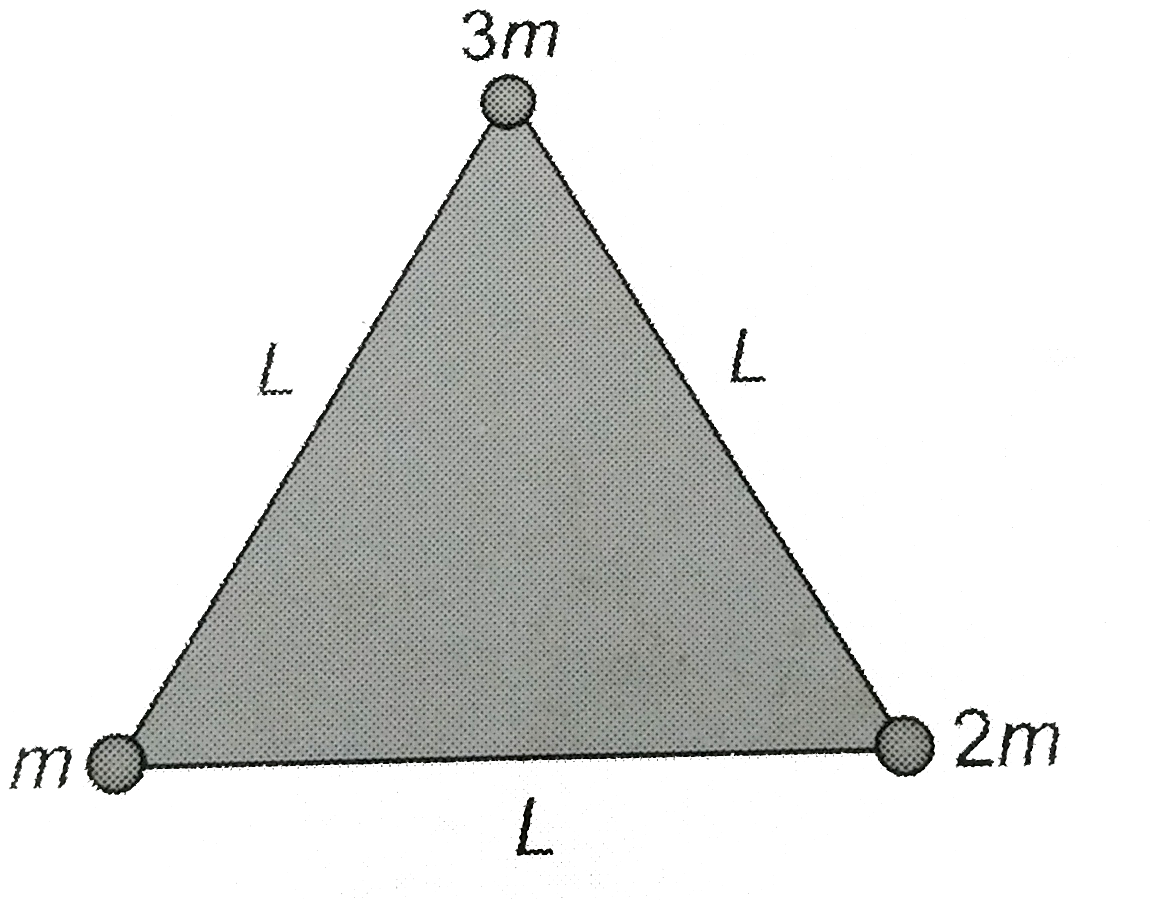 View Solution
View SolutionConsider the situation as shown in the figure. Find TminTmax.
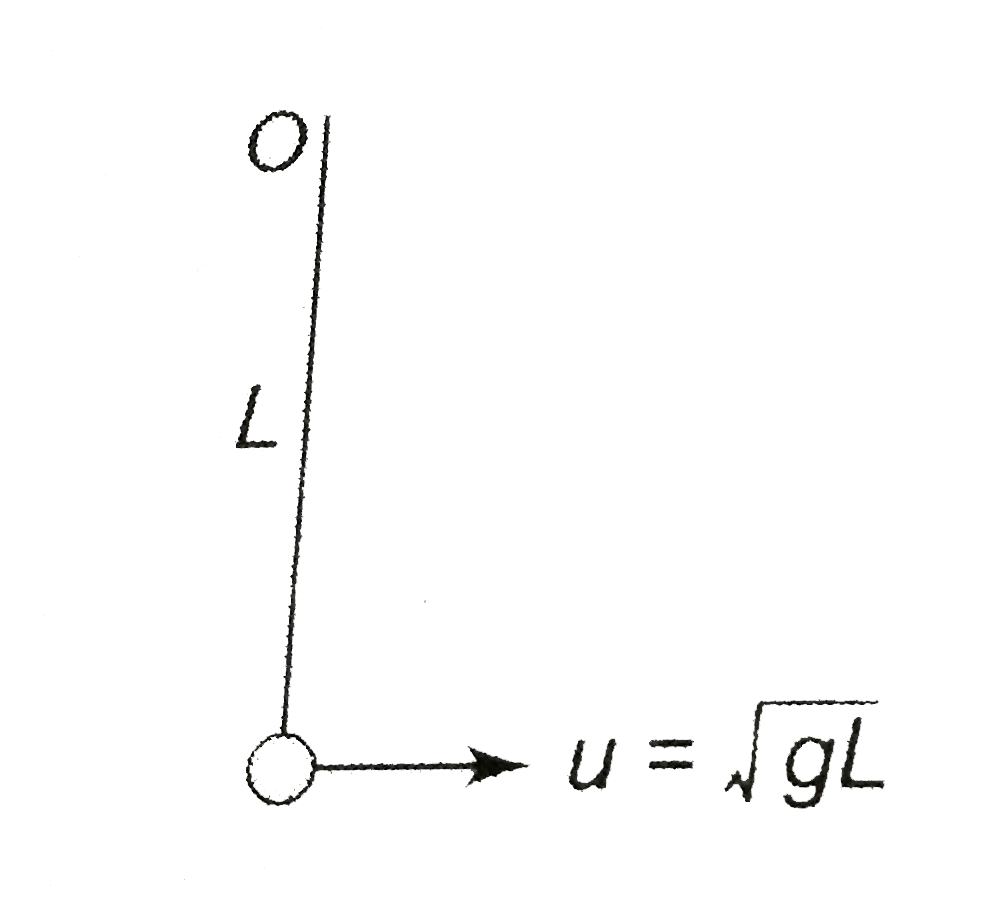 View Solution
View SolutionConsider the situation as shown in the figure. Choose the correct option.
 View Solution
View SolutionA uniform cubical block of mass M is attached to a pulley. One end of a string, passing over the pulley is fixed to a light pole while is fixed on the bigger block and its another end is attached to a block of mass m as shown in figure. The maximum value of the mass m so that the block does not topple is : (string and pulley are ideal)
 View Solution
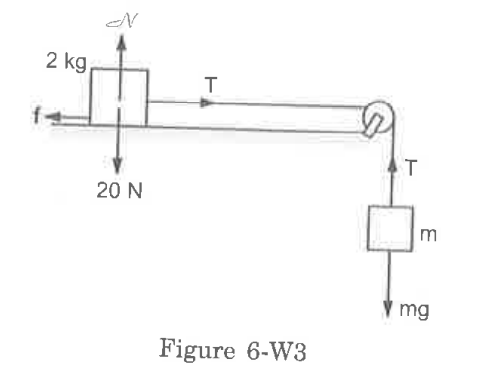
View SolutionThe coefficient of static friction between the block of 2 kg and the table shown in figure is μs=02. What should be the maximum value of m so that the blocks does not move? Take g=10m/s2. The string and the pulley are light and smooth.
 View Solution
View Solution

